TRANSFORMATIONS OF FUNCTIONS
In this section, you will learn how to do different types of transformations of functions like translation, stretch, compression and reflection.
To make the students to understand the different types of transformations, we have explained each kind of transformation with step by step explanation along with the corresponding figures.
Transformations - Definition
A very simple definition for transformations is, whenever a figure is moved from one location to another location, a Transformation occurs.
If a figure is moved from one location another location, we say, it is transformation.
Our next question is, how will the transformation be?
To know that, we have to be knowing the different types of transformations.
Now, let us come to know the different types of transformations.
Different Types of Transformations
The different types of transformations which we can do in the functions are
3. Reflection across the x-axis
4. Reflection across the y-axis
5. Horizontal Stretch and Compression
6. Vertical Stretch and Compression
How different types of transformations occur in terms of x-coordinate and y-coordinate have been summarized below.
Summary of Transformation
Transformation
Appearance in Function
Transformation of Point
Vertical Translation
f(x) ---> f(x) + d
(x, y) --> (x, y + d)
Horizontal Translation
f(x) ---> f(x - c)
(x, y) --> (x + c. y)
Vertical Stretch or Compression
f(x) ---> af(x)
(x, y) --> (x, ay)
Horizontal Stretch or Compression
f(x) ---> f(kx)
(x, y) --> (x/k, y)
Reflection in x-axis
f(x) ---> -f(x)
(x, y) --> (x, -y)
Reflection in y-axis
f(x) ---> f(-x)
(x, y) --> (-x, y)
Order of Transformations
In transformations of functions, if we have more than one transformation, we have to do the transformations one by one in the following order.
1. Stretches/Compressions
2. Reflections
3. Translations
What is image and pre-image?
When a transformation occurs, the original figure is known as the pre-image and the new figure is known as the image.
It has been clearly shown in the below picture.
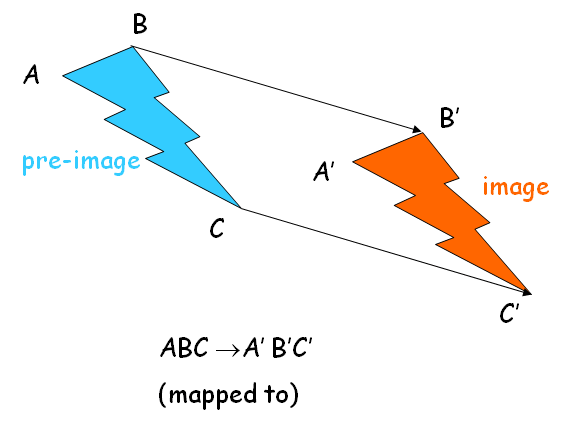
When a figure is moved from one location to another location, we say that it is a transformation.
In this point, always students have the following questions.
How is this transformation made?
More clearly, on what grounds is the transformation made ?
Is there any pre-decided rule to make transformation?
Yes, there is a pre-decided rule to make each and every transformation.
The rule we apply to make transformation is depending upon the kind of transformation we make.
We have already seen the different types of transformations in functions.
For example, if we are going to make transformation of a function using reflection through the x-axis, there is a pre-decided rule for that. According to the rule, we have to make transformation.
The rule that we apply to make transformation using reflection and the rule we apply to make transformation using rotation are not same.
Hence, for each type of transformation, we may have to apply different rule.
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
10 Hard SAT Math Questions (Part - 30)
Oct 17, 25 07:27 PM
10 Hard SAT Math Questions (Part - 30) -
10 Hard SAT Math Questions (Part - 29)
Oct 16, 25 06:04 AM
10 Hard SAT Math Questions (Part - 29) -
10 Hard SAT Math Questions (Part - 28)
Oct 14, 25 10:57 AM
10 Hard SAT Math Questions (Part - 28)
