SPHERE
Definition of Sphere :
This is a solid generated when a semicircle is being rotated about its diameter. In three dimensional space, this is also known as perfect round geometrical object.

A plane is at the center of the spherical solid divides the solid in to two equal parts. Each shape is called hemisphere.
Example :
A very good example we can say for spherical shaped solid is globe. Ball is another good example for spherical shaped solid.
Curved surface area A = 4πr2
Volume of sphere = (4/3)πr3
Example 1 :
The formula for calculating the total surface area A of a sphere of radius r is A = 4πr2.
Find:
a) the total surface area of a sphere of radius 7.5 cm
b) the radius, in cm, of a spherical balloon which has a surface area of 2 m2.
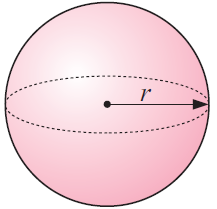
Solution :
Radius = 7.5 cm
Total surface area of the sphere A = 4πr2
= 4π(7.5)2
= 225π cm2
So, the total surface area of the sphere is 225π cm2.
Example 2 :
A sphere of radius r has volume given by
V = (4/3)πr3
Find :
a) the volume of a sphere of radius 2.37 m
b) the radius of a sphere that has volume 2500 cm3.
Solution :
(a) Volume of sphere (V ) = (4/3)πr3
Radius (r) = 2.37 m
= (4/3)π(2.37)3
= (4/3)π(133.12)
= 177.49 π cm3
(b) Volume of sphere = 2500 cm3
(4/3)πr3 = 2500
r3 = 2500/4.18
r = 8.42
Example 3 :
A spherical art piece has diameter 2 meters. Find
(a) The surface area of the sphere
(b) The cost of painting the sphere (with 3 coats) given each square meter will cost $13.50 for paint and labour.
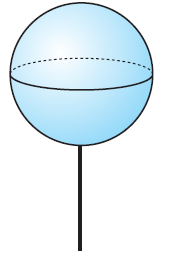
Solution :
Diameter (a) = 2 m and radius = 1 m
(a) Surface area of sphere = 4πr2
= 4π(1)2
= 4π cm2
(b) Cost of painting = $13.50
Cost for 1 coat = 4π (13.50)
= 54π
= 54 x 3.14
= 169.56
Cost for 3 coats = 3(169.56)
= $508.68
So, the required cost is $508.68.
Example 4 :
Find the size of the radius in each of the spheres below. Give your answers to one decimal place
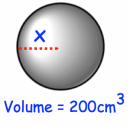
Solution :
Volume of sphere = (4/3)πr3
Here r = x cm
200 = (4/3)πx3
200 x (3/4) x 1/3.14 = x3
x3 = 47.7
x = 3.6 cm
Example 5 :
A metal cuboid measuring 4cm by 5cm by 12cm is melted down and a sphere is made. Calculate the radius of the sphere.
Solution :
Since the cuboid is melted and made a sphere, the volume of cuboid and sphere both will be equal.
Volume of cuboid = volume of sphere
l x b x h = (4/3)πr3
4 x 5 x 12 = (4/3)πr3
r3 = 4 x 5 x 12 x (3/4) x 1/3.14
r3 = 57.32
r = 3.85 cm
So, the radius of the sphere is 3.85 cm.
Example 6 :
Julie thinks that if you double the radius of the sphere shown at the right, the surface area will double. Is she right? Explain your reasoning

Solution :
From the given figure, the radius of the sphere = 3 cm
When we double the radius, the new radius will be 6 cm.
Surface area of having the radius of 3 cm.
= 4πr2
= 4π(3)2
= 36 π cm2
Surface area of having the radius of 6 cm.
= 4π (6)2
= 144 π cm2
When we double the radius the surface area will not become twice. So, her argument is not correct.
Example 7 :
Estimate the volume of air in a beach ball that has a 12 inch diameter. Round your answer to the nearest whole number.
Solution :
Diameter = 12 inch
Radius of sphere = 6 inches
Volume of hemisphere = (2/3)πr3
= (2/3)π(6)3
= 2/3 x 3.14 x 216
= 452.16 cubic inches.
Example 8 :
Find the volume of a sphere whose surface area is 154 cm2 .
Solution :
Surface area of sphere = 154 cm2
2πr2 = 154
2 x 22/7 x r2 = 154
r2 = 154 x (7/22) x (1/2)
r2 = 24.5
r = 4.94 cm
r = 5 cm approximately
Example 9 :
A capsule of medicine is in the shape of a sphere of diameter 3.5 mm. How much medicine (in mm3 ) is needed to fill this capsule?
Solution :
Diameter of the sphere = 3.5 mm
Radius of the sphere = 1.75 mm
Volume of sphere = (4/3)πr3
= (2/3)π(1.75)3
= 3.57 π mm3
Example 10 :
The diameter of a sphere is decreased by 25%. By what % does its volume decreased.
Solution :
Diameter of the sphere = D
radius of the sphere = D/2
Volume of sphere = (4/3)π(D/2)3
= (4/3)π(D3/8)
= πD3/6
New radius of the sphere = 75% of (D/2)
= 0.75D/2
= 0.375 D
Volume of the sphere = (4/3)πr3
= (4/3)π(0.375D)3
= 0.05(4/3)πD3
= (5/100)(4/3)πD3
= 0.05(4) (πD3/3)
= 0.05(4) (2/2) (πD3/3)
= 0.4 (1/2) (πD3/3)
= 40% of (πD3/6)
So, 60% is reduced.
Example 11 :
The volumes of two hemispheres are in the ratio 27:125. Find the ratio of the radii.
Solution :
Let r1 and r2 be radii of two hemispheres.
(2/3)πr13 : (2/3)πr23 = 27 : 125
r13 : r23 = 27 : 125
r13 : r23 = 33 : 53
So, the ratio between their radii is 3 : 5.
Related Pages
- How to find volume of sphere
- Volume of sphere practice problems
- Surface area and volume of spheres
- Curved surface area and total surface area of sphere and hemisphere
- Examples on volume of sphere and hemisphere
- Volume of sphere and hemisphere worksheet
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
10 Hard SAT Math Questions (Part - 30)
Oct 17, 25 07:27 PM
10 Hard SAT Math Questions (Part - 30) -
10 Hard SAT Math Questions (Part - 29)
Oct 16, 25 06:04 AM
10 Hard SAT Math Questions (Part - 29) -
10 Hard SAT Math Questions (Part - 28)
Oct 14, 25 10:57 AM
10 Hard SAT Math Questions (Part - 28)
