SLOPE OF A LINE WORKSHEET

Problem 1 :

Find the angle of inclination of the straight line whose slope is ¹⁄√₃.

Problem 2 :

Find the slope of the straight line passing through the points (3, -2) and (-1, 4). 

Problem 3 :

Using the concept of slope, show that the points A(5, -2), B(4, -1) and C(1, 2) are collinear.

Problem 4 :

Find the slope of the line 3x - 2y + 7 = 0. 

Problem 5 :

If the straight line 5x + ky - 1 = 0 has the slope 5, find the value of k.  

Problem 6 :

If the straight line kx - 2y + 3  =  0 is passing through the (1, 3), find its slope.  

Problem 7 : 

Find the slope of the line shown below using rise over run formula.  

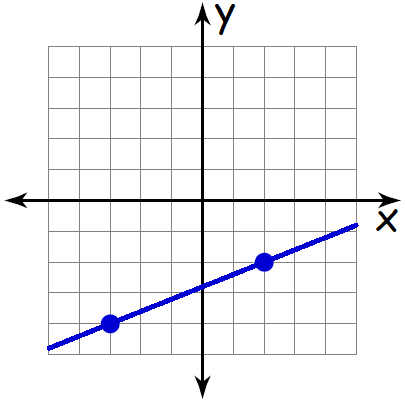
Problem 8 :

Find the slope of the line shown below using rise over run formula.  

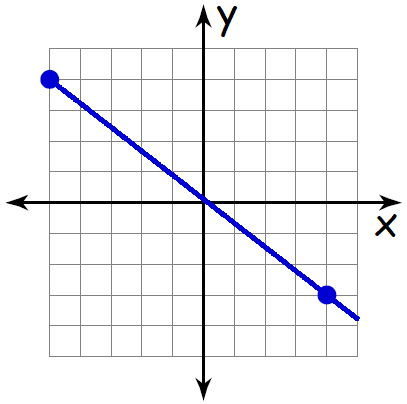
Problem 9 :

Find the slope of the line shown below using rise over run formula.  

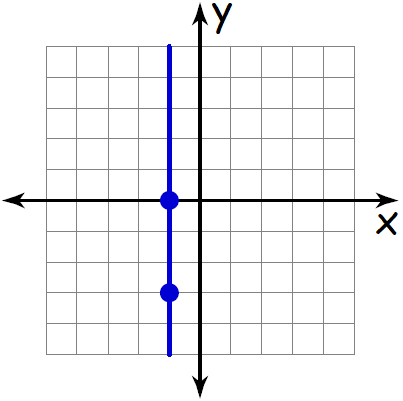
Problem 10 :

Find the slope of the line shown below using rise over run formula.  

Answers

1. Answer :

Let θ be the angle of inclination of the line. 

Then, slope of the line is

m = tanθ

Given : Slope = ¹⁄√₃.

tanθ = ¹⁄√₃  

θ = tan^-1(¹⁄√₃)

θ = 30°

2. Answer :

Let (x₁, y₁) = (3, -2) and (x₂, y₂) = (-1, 4).

Then, the formula to find the slope,

m = ⁽ʸ2 ⁻ ʸ1⁾⁄₍ₓ₂ ₋ ₓ₁₎

Substitute (x₁, y₁) = (3, -2) and (x₂, y₂)  = (-1, 4).

m = ⁽⁴ ⁺ ²⁾⁄₍₋₁ ₋ ₃₎

= -⁶⁄₄

= -³⁄₂

3. Answer :

Slope of the line joining (x₁, y₁) and (x₂, y₂) is,

m = ⁽ʸ2 ⁻ ʸ1⁾⁄₍ₓ₂ ₋ ₓ₁₎

Using the above formula, slope of the line AB joining the points A(5, -2) and B(4, -1) is

m = ⁽⁻¹ ⁺ ²⁾⁄₍₄ ₋ ₅₎

= -1

Slope of the line BC joining the points B(4, -1) and C(1, 2) is

 = ⁽² ⁺ ¹⁾⁄₍₁ ₋ ₄₎

= -1

Thus,

slope of AB = slope of BC

Also, B is the common point.

So, the points A, B and C are collinear.

4. Answer :

Equation of the given line :

3x - 2y + 7 = 0

Write the above equation in slope intercept form, that is

y = mx + b

where m is the slope and b is the intercept.

3x - 2y + 7 = 0

-2y = -3x - 7

2y = 3x + 7

y = ³ˣ⁄₂ + ⁷⁄₂

y = (³⁄₂)x + ⁷⁄₂

The above equation is in slope intercept form.

m = ³⁄₂

So, the slope of the given line is ³⁄₂.

5. Answer :

Equation of the given line :

5x + ky - 1 = 0

Write the above equation in slope intercept form.

5x + ky - 1 = 0

ky = -5x + 1

y = -⁵ˣ⁄k + ¹⁄k

y = (-⁵⁄k)x + ¹⁄k

The above equation is in slope intercept form.

m = -⁵⁄k

Given : Slope = 5.

5 = -⁵⁄k

5k = -5

Divide both sides by -5.

-1 = k

6. Answer :

Because the straight line kx - 2y + 3 = 0 is passing through the point (1, 3), we can substitute x = 1 and y = 3 into the equation.

k(1) - 2(3) + 3 = 0

k - 6 + 3 = 0

k - 3 = 0

k = 3

Equation of the given straight line :

x - 2y + 3 = 0

Write the above equation in slope intercept form.

x - 2y + 3 = 0

-2y = -x - 3

2y = x + 3

y = ˣ⁄₂ + ³⁄₂

y = (½)x + ³⁄₂

The above equation is in slope intercept form.

m = ½

7. Answer :

The above line is a rising line. So, its slope will be a positive value.

Measure the rise and run.

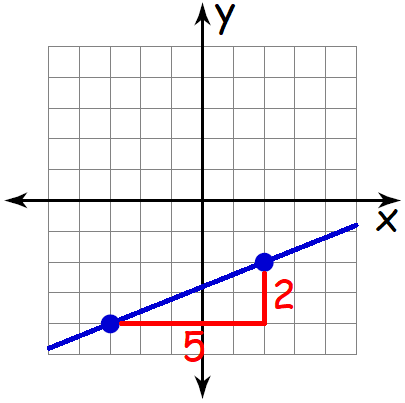
For the above line,

rise = 2

run = 5

Then,

slope = ʳⁱˢᵉ⁄ᵣᵤₙ

slope = ⅖

8. Answer :

The above line is a falling line. So, its slope will be a negative value.

Measure the rise and run.

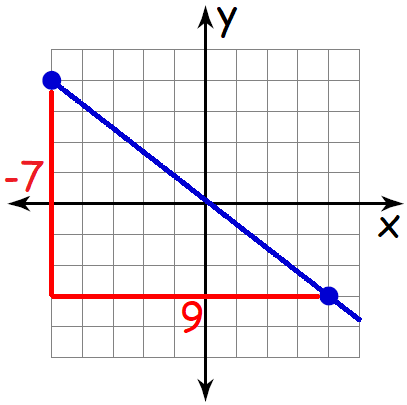
For the above line,

rise = 7

run = 9

Then,

slope = ʳⁱˢᵉ⁄ᵣᵤₙ

slope = -⁷⁄₉

9. Answer :

The above line is a vertical line.

Measure the rise and run.

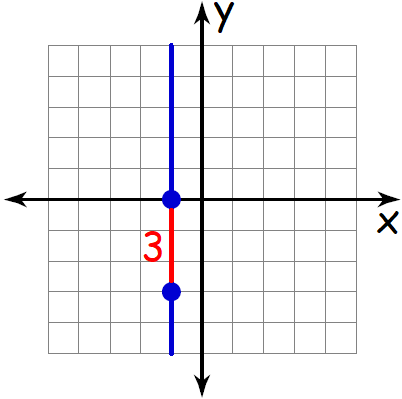
For the above line,

rise = 3

run = 0

Then,

slope = ʳⁱˢᵉ⁄ᵣᵤₙ

slope = ³⁄₀

slope = undefined

Note :

The slope of a vertical line is always undefined.

10. Answer :

The above line is an horizontal line.

Measure the rise and run.

For the above line,

rise = 0

run = 4

Then,

slope = ʳⁱˢᵉ⁄ᵣᵤₙ

slope = ⁰⁄₄

slope = 0

Note :

The slope of an horizontal line is always zero.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.