HOW TO FIND THE EQUATION OF A LINE WITH ONE POINT

Question 1 :

Write the equations of the straight lines parallel to x-axis which are at a distance of 5 units from the x-axis

Solution :

The line which is drawn from above the x axis and parallel to the x axis will be a horizontal line.

So, the required equations will be y = 5 and y = -5.

Question 2 :

Find the equations of the straight lines parallel to the coordinates axes and passing through the point (-5, -2)

Solution :

via one point, we can draw infinite number of lines. Since the required lines will be parallel to x and y axis, we can draw two lines as follows.

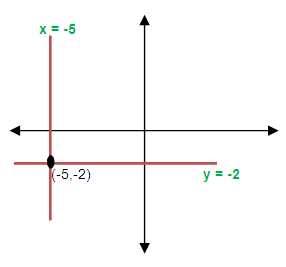
Therefore the two required equations are

y = -2 and x = -5.

Question 3 :

Find the equation of a straight line whose

(i) Slope is -3 and y-intercept is 4.

Solution :

Slope (m)  =  -3

Y-intercept (b)  =  4

Equation of the straight line:

y  =  mx + b

y  =  3x + 4

So, the required equation of the line y = 3x+4.

(ii) Angle of inclination is 60 degree and y-intercept is 3.

Solution :

Slope (m)  =  tan  θ

m  =  tan 60

m  =  √3

y-intercept (b) = 3

Equation of the straight line :

y  =  mx + b

y  =  √3x + 3

So, the required equation of the line y = √3x + 3.

Question 4 :

Find the equation of the line intersecting the y-axis at a distance of 3 units above the origin and

tan  θ = 1/2

where Ѳ is the angle of inclination.

Solution :

The required line is intersecting the y-axis at a distance of 3 units above the origin. So we can take y-intercept as 3

tan θ  =  1/2

m  =  1/2

Equation of the straight line :

y  =  mx + b

y  =  (1/2)x + 3

y  =  (x + 6)/2

2y  =  x + 6

x – 2y + 6  =  0

Question 5 :

Find the equation of the straight line which passes through the midpoint of the line segment joining

(4, 2) and (3, 1)

whose angle of inclination is 30 degree.

Solution :

First we have to find midpoint of the line segment joining the points (4, 2) and (3, 1)

Midpoint  =  (x₁ + x₂)/2, (y₁ + y₂)/2

=  (4 + 3)/2, (2 + 1)/2

=  (7/2, 3/2)

angle of inclination  =  30°

θ  =  30°

Slope (m)  =  tan θ

m  = tan 30°

m  =  1/√3

Equation of the line :

(y - y₁)  =  m(x - x₁)

(y - (3/2))  =  (1/√3)(x - (7/2))

(2y - 3)  =  (1/√3)(2x - 7)

2√3y - 3√3  =  2x - 7

2x - 2√3y - 7 + 3√3 = 0

Question 6 :

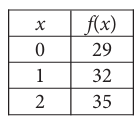
For the linear function f, the table shows three values of x and their corresponding values of f x equation defines f(x) ?

a)  f(x) = 3x + 29      b)  f(x) = 29x + 32

c)  f(x) = 35x + 29     d)  f(x) = 32x + 35

Solution :

From the table, choosing two points from the table (0, 29) and (1, 32) we find the slope.

Slope = (32 - 29) / (1 - 0)

= 3/1

= 3

y-intercept is 29

Equation of the line :

y = mx + b

y = 3x + 29

So, option a is correct.

Question 7 :

Hana deposited a fixed amount into her bank account each month. The function

f(t) = 100t + 25

gives the amount, in dollars, in Hana’s bank account after t monthly deposits. What is the best interpretation of 25 in this context?

a) With each monthly deposit, the amount in Hana’s bank account increased by $25.

b) Before Hana made any monthly deposits, the amount in her bank account was $25.

c) After 1 monthly deposit, the amount in Hana’s bank account was $25.

d) Hana made a total of 25 monthly deposits.

Solution :

f(t) = 100t + 25

Here t represents monthly deposits and f(t) will be the amount. 

f(1) = 100 + 25 ==> 125

f(2) = 200 + 25 ==>  225

So, option b, before Hana made any monthly deposits, the amount in her bank account was $25 is correct.

Question 8 :

Which of the following equations represents a line that passes through (7, 6) and is parallel to the -x axis?

A) x = 6   B) x = 7    C) y = 6    D) y = 7

Solution :

Via a point we can draw infinite number of lines, since we are drawing a line which is parallel to x-axis the required equation will be x = 7. 

Question 9 :

Which of the following equations represents a line that passes through (-5, 1) and is parallel to the y axis?

A) y = -5   B) y = 1   C) x = -5    D) x = 1

Solution :

Equation of a line which is parallel to y-axis will be in the form y = a. So, the required equation will be 

y = 1

Question 10 :

f(x) = ax + 2

In the function above, a is a constant. If f(-1) = 4, what is the value of f(-1/2) ?

Solution :

f(x) = ax + 2

f(-1) = 4

Applying x = -1 and y = 4

4 = a(-1) + 2

4 - 2 = -a

-a = 2

a = -2, then 

f(x) = -2x + 2

Now applying -1/2 for x, we get

f(-1/2) = -2(-1/2) + 2

= 1 + 2

= 3

So, the value of f(-1/2) is 3.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.