BASIC TRIGONOMETRIC RATIOS

We know that six trigonometric ratios can be formed using the three lengths a, b and c of sides of a right triangle ABC.

Interestingly, these ratios lead to the definitions of six basic trigonometric functions.

First, let us recall the trigonometric ratios which are defined with reference to a right triangle.

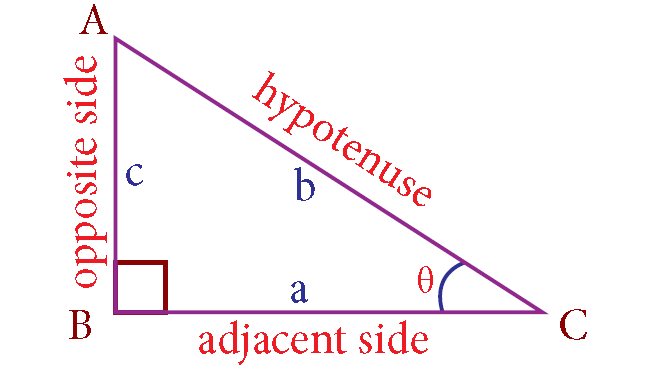
sin θ  =  opposite side/hypotenuse

cos θ  =  adjacent side/hypotenuse

With the help of sin θ and cos θ, the remaining trigonometric ratios tan θ, cot θ, csc θ and sec θ are determined by using the relations

tan θ  =  sin θ/cos θ

csc θ  =  1/sin θ

sec θ  =  1/cos θ

cot θ  =  cos θ/sin θ

And also, 

sin θ  =  1/csc θ

cos θ  =  1/sec θ

Note :

1. sin θ and csc θ are reciprocal to each other.

2. cos θ and sec θ are reciprocal to each other.

3. tan θ and cot θ are reciprocal to each other.

Example :

1. If sin θ  =  3/5, then csc θ  =  5/3.

2. If cos θ  =  4/5, then sec θ  =  5/4.

3. If tan θ  =  3/4, then cot θ  =  4/3.

Solved Problems

Problem 1 :

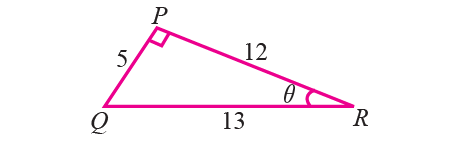
In the right triangle PQR given below, find the basic trigonometric ratios of the angle θ.

Solution :

In the triangle shown above, for the angle θ, 

opposite side  =  5

adjacent side  =  12

hypotenuse  =  13

Then, the basic trigonometric ratios of the angle θ are 

Problem 2 :

From the figure given below, find the six trigonometric ratios of the angle θ.

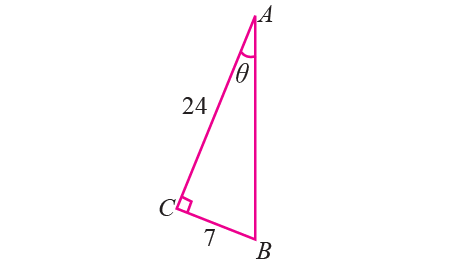
Solution :

In the triangle shown above, by Pythagorean Theorem, 

AB²  =  BC² + CA²

AB²  =  7² + 24²

AB²  =  49 + 576

AB²  =  625

AB²  =  25²

AB  =  25

In the triangle shown above, for the angle θ, 

opposite side  =  7

adjacent side  =  24

hypotenuse  =  25

Then, the basic trigonometric ratios of the angle θ are 

Problem 3 :

If sin θ  =  13/85 and cos θ  =  84/85, then find the values of tan θ and cos θ. 

Solution :

Finding the value of tan θ : 

tan θ  =  sin θ/cos θ

tan θ  =  (13/85)/(84/85)

tan θ  =  (13/85) ⋅ (85/84)

tan θ  =  (13 ⋅ 85)/(85 ⋅ 84)

tan θ  =  13/84

Finding the value of cot θ :

cot θ  =  84/13

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.