AP Calculus AB Problems with Solutions (Part - 23)

Problem 1 :

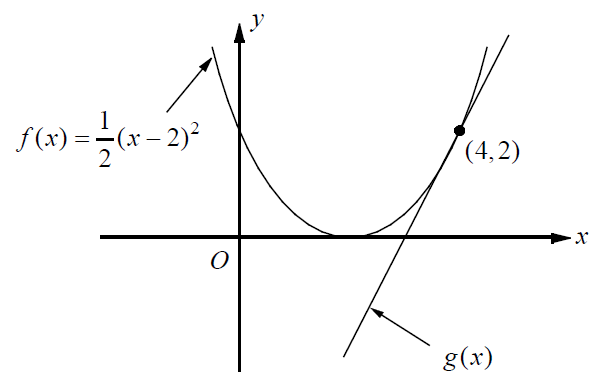
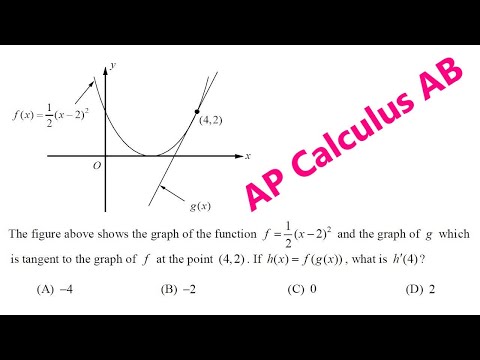
The figure above shows the graph of the function f = ½(x - 2)² and the graph of g which tangent to the graph of f at the point (4, 2). If h(x) = f[g(x)], what is h'(4)?

A)  -4

B)  -2

C)  0

D)  2

Solution :

Problem 2 :

A)  0

B)  1

C)  2

D)  3

Solution :

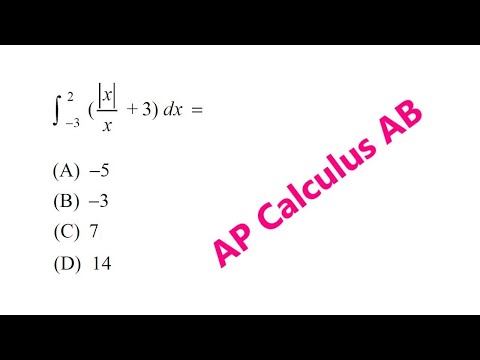
Problem 3 :

A)  -5

B)  -3

C)  7

D)  14

Solution :

Problem 4 :

If f is a continuous function and F'(x) = f(x) for all real numbers x, then

A)  2[F(2) - F(1)]

B)  2[F(4) - F(1)]

C)  ½[F(2) - F(1)]

D)  ½[F(4) - F(1)]

Solution :

Problem 5 :

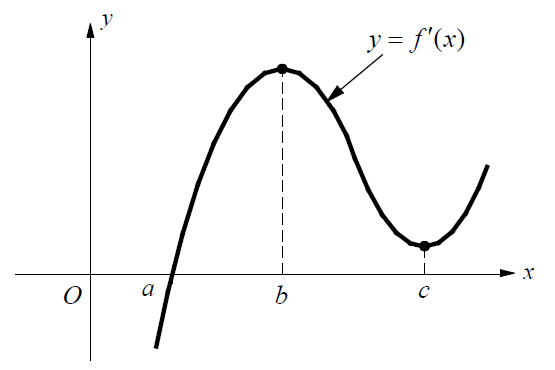
The graph of f' is shown in the figure above. Which of the following statements about f are true?

I.  f has a relative minimum at x = a.

II.  f has a relative maximum at x = b.

III.  f is decreasing on the interval b < x < c.

A)  None

B)  I only

C)  I and III only

D)  II and III only

Solution :

You might like these

AP Calculus AB Problems with Solutions (Part - 1)

AP Calculus AB Problems with Solutions (Part - 2)

AP Calculus AB Problems with Solutions (Part - 3)

AP Calculus AB Problems with Solutions (Part - 4)

AP Calculus AB Problems with Solutions (Part - 5)

AP Calculus AB Problems with Solutions (Part - 6)

AP Calculus AB Problems with Solutions (Part - 7)

AP Calculus AB Problems with Solutions (Part - 8)

AP Calculus AB Problems with Solutions (Part - 9)

AP Calculus AB Problems with Solutions (Part - 10)

AP Calculus AB Problems with Solutions (Part - 11)

AP Calculus AB Problems with Solutions (Part - 12)

AP Calculus AB Problems with Solutions (Part - 13)

AP Calculus AB Problems with Solutions (Part - 14)

AP Calculus AB Problems with Solutions (Part - 15)

AP Calculus AB Problems with Solutions (Part - 16)

AP Calculus AB Problems with Solutions (Part - 17)

AP Calculus AB Problems with Solutions (Part - 18)

AP Calculus AB Problems with Solutions (Part - 19)

AP Calculus AB Problems with Solutions (Part - 20)

AP Calculus AB Problems with Solutions (Part - 21)

AP Calculus AB Problems with Solutions (Part - 22)

AP Calculus AB Problems with Solutions (Part - 23)

AP Calculus AB Problems with Solutions (Part - 24)

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.