WORKSHEET ON ARITHMETIC MEAN

Question 1 :

Ages of the First 12 United States Presidents at the Beginning of Their Terms in Office.

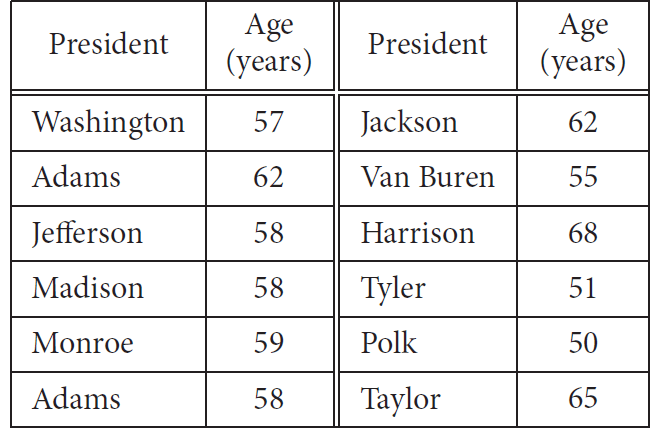
The table above lists the ages of the first 12 United States presidents when they began their terms in office. According to the table, what was the mean age, in years, of these presidents at the beginning of their terms? (Round your answer to the nearest tenth.)

Question 2 :

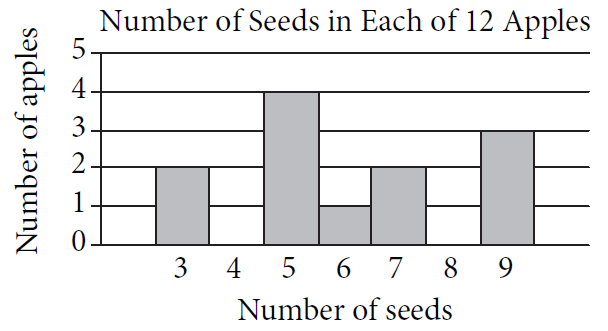
Based on the histogram above, of the following, which is closest to the average (arithmetic mean) number of seeds per apple?

A) 4

B) 5

C) 6

D) 7

Question 3 :

A survey was taken of the value of homes in a county, and it was found that the mean home value was $165,000 and the median home value was $125,000. Which of the following situations could explain the difference between the mean and median home values in the county?

A) The homes have values that are close to each other.

B) There are a few homes that are valued much less than the rest.

C) There are a few homes that are valued much more than the rest.

D) Many of the homes have values between $125,000 and $165,000.

Question 4 :

An online store receives customer satisfaction ratings between 0 and 100, inclusive. In the first 10 ratings the store received, the average (arithmetic mean) of the ratings was 75. What is the least value the store can receive for the 11th rating and still be able to have an average of at least 85 for the first 20 ratings?

Question 5 :

If x is the average (arithmetic mean) of m and 9, y is the average of 2m and 15, and z is the average of 3m and 18, what is the average of x, y, and z in terms of m?

A) m + 6

B) m + 7

C) 2m + 14

D) 3m + 21

Question 6 :

The mean score of 8 players in a basketball game was 14.5 points. If the highest individual score is removed, the mean score of the remaining 7 players becomes 12 points. What was the highest score?

A) 20

B) 24

C) 32

D) 36

1. Answer :

The mean of a data set is determined by calculating the sum of the values and dividing by the number of values in the data set.

Sum of ages :

= 57+ 62 + 58 + 58 + 59 + 58 + 62 + 55 + 68 + 51 + 50 + 65

= 703

Mean age :

= 703/12

≈ 58.6

2. Answer :

The average number of seeds per apple is the total number of seeds in the 12 apples divided by the number of apples, which is 12.

There are

3 apples with 2 seeds each ----> 3 x 2 = 6 seeds

5 apples with 4 seeds each ----> 5 x 4 = 20 seeds

6 apples with 1 seed each ----> 6 x 1 = 6 seeds

7 apples with 2 seeds each ----> 7 x 2 = 14 seeds

9 apples with 3 seeds each ----> 9 x 3 = 27 seeds

Total number of seeds in 12 apples :

= 6 + 20 + 6 + 14 + 27

= 73

Average number of seeds per apple :

= 73/12

≈ 6.08

Of the choices given, 6 is closest to 6.08.

The correct answer choice is (C).

3. Answer :

Median is the middle value of a data.

For the data given, the median is $125,000. So, 50% of the homes are valued less than $125,000 and 50% of the homes are valued more than $125,000.

For any data, mean the best representative all the values in the data.

In the data given, mean is greater than median. So, most of the homes are valued less than the mean and few homes are valued more than the mean.

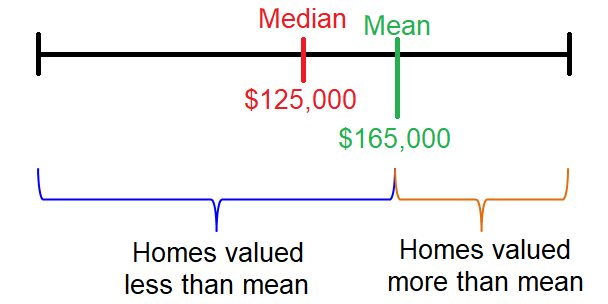
The correct answer choice is (C).

4. Answer :

Sum of the ratings from 11 to 20 :

= 1700 - 750

= 950

There are ten ratings from 11 to 20.

In these ten ratings from 11 to 20, if the nine ratings from 12 to 20 received the maximum rating 100 each, then the maximum possible value of the sum of the nine ratings (from 12 to 20) is

Therefore, the least possible value for the 11^th rating is

= 950 - 900

= 50

5. Answer :

x ----> average of m and 9

y ----> average of 2m and 15

z ----> average of 3m and 18

Then, we have

Average of x, y, and z :

The correct answer choice is (B).

6. Answer :

Highest individual score :

= 116 - 94

= 22

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.