SOLVING WORD PROBLEMS ON PROPORTION

A proportion is an equation that states that two ratios or rates are equivalent.

Examples :

⅓ and ²⁄₆ are equivalent ratios

⅓ = ²⁄₆ is a proportion

We use cross product rule in proportion to solve many real world problems.

Let us consider the proportion

a : b  =  c : d

To know the cross product rule, first we have to know about extremes and means. 

It has been explained in the picture given below. 

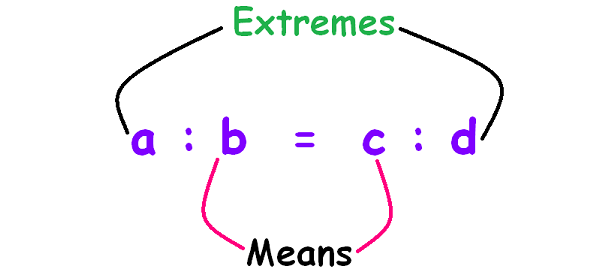
Cross Product Rule :

Product of extremes  =  Product of means

ad  =  bc

Problem 1 :

Christina takes 2 hours to travel 35 km. How far will she travel in 6 hours ?

Solution :

The ratio between number of hours and distance traveled is 

2 : 35 ----(1)

Let x be the distance traveled by her in 6 hours.

6 : x ----(2)

From (1) and (2), 

2 : 35 = 6 : x

2 ⋅ x = 6 ⋅ 35

2x = 210

Divide each side by 2. 

x = 105

So, the distance covered by Christina in 6 hours is 105 km.

Problem 2 :

If a person reads 20 pages in a book in 2 hours, how many pages will he read in 8 hours at the same speed ?

Solution :

The ratio between number of pages  and time taken 

= 20 : 2

= 10 : 1 ----(1)

Let x be the number of pages read in 8 hours.

x : 8 ----(2)

From (1) and (2), 

10 : 1 = x : 8

10 ⋅ 8 = 1 ⋅ x

80 = x

So, the person can read 80 pages in 8 hours. 

Problem 3 :

If 15 people can repair a road of length 150 meters, at the same rate, how many people are needed to repair a road of length 420 meters.

Solution :

The ratio between number of people and length of road repaired is

= 15 : 150

= 1 : 10 ----(1)

Let x be the number of people needed to repair a road of length 420 meters.

x : 420 ----(2)

From (1) and (2), 

1 : 10 = x : 420

1 ⋅ 420 = 10 ⋅ x

420 = 10x

Divide each side by 10. 

42 = x

So, 42 people are needed to repair a road of length 420 meters. 

Problem 4 :

If the cost of 10 kg rice is $400, then find the cost of 3 kg rice.

Solution :

The ratio between the number of kilograms of rice and the cost is

10 : 400 ----(1)

Let x be the cost of 3 kilograms of rice.

3 : x ----(2)

From (1) and (2), 

10 : 400 = 3 : x

10 ⋅ x = 3 ⋅ 400

10x = 1200

Divide each side by 10.

x = 120

So, the cost of 3 kg rice is $120.

Problem 5 :

A car needs 12 liters of petrol to cover a distance of 156 miles. How much petrol will be required for the car to cover a distance of 1300 miles?

Solution :

The ratio between the number of liters of petrol and distance covered is

= 12 : 156

= 1 : 13 ----(1)

Let x be the number of liters of petrol required to cover a distance 1300 km.

x : 1300 ----(2)

From (1) and (2), 

1 : 13 = x : 1300

1(1300) = x(13)

1300 = 13x

Divide each side by 13.

100 = x

So, 100 liters of petrol will be required to cover a distance of of 1300 miles.

Problem 6 :

If you can buy one can of pineapple chunks for $2 then how many can you buy with $10?

Solution :

From the given information, number of cans of pineapple chunks and the cost are in the ratio

1 : 2

Let x be the number of cans that we buy.

Then,

1 : 2 = x : 10

1 ⋅ 10 = 2 ⋅ x

10 = 2x

10 = 2x

5 = x

So, we can buy 5 apples with $10.

Problem 7 :

Shawna reduced the size of a rectangle to a height of 2 in. What is the new width, if it was originally 24 in wide and 12 in tall?

Solution :

From the given information, the width and height  of the rectangle are in the ratio

24 : 12 = 2 : 1

Because the original width and height of the rectangle are in the ratio 2 : 1, the width and height of he resized rectangle will also be in the same ratio.

And also, the height of the rectangle is reduced to 2 inches.

Let x be the width of the resized rectangle.

Then,

2 : 1 = x : 2

2 ⋅ 2 = 1 ⋅ x

4 = x

So, the width of the resized rectangle is 4 inches.

Problem 8 :

One cantaloupe costs $2. How many cantaloupes can we buy for $6?

Solution :

From the given information, number of cans of pineapple chunks and the cost are in the ratio

1 : 2

Let x be the number of cantaloupe that we buy.

Then,

1 : 2 = x : 6

1 ⋅ 6 = 2 ⋅ x

6 = 2x

3 = x

So, we can buy 3 cantaloupes for $6.

Problem 9 :

Ming was planning a trip to Western Samoa. Before going, she did some research and learned that the exchange rate is 6 Tala for $2. How many Tala would she get if she exchanged $6?

Solution :

From the given information, the exchange rate of Tala and Dollar are in the ratio

6 : 2 = 3 : 1

Let x be the number of Tala that Ming would expect.

Then,

3 : 1 = x : 6

3 ⋅ 6 = 1 ⋅ x

18 = x

So, Ming would get 18 Tala, if she exchanged $6.

Problem 10 :

Jasmine bought 32 kiwi fruit for $16. How many kiwi she Lisa buy, if she has $4?

Solution :

From the given information, we come to know that number of kiwi fruits to the cost is in the ratio.

32 : 16 = 2 : 1

Let x be the number of kiwi that jasmine buy for $4.

2 : 1 = x : 4

2 ⋅ 4 = 1 ⋅ x

8 = x

So, Jasmine can buy 8 kiwi fruits for $4.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.