USING PROPERTIES OF DEFINITE INTEGRALS

Problem 1 :

Evaluate the following integrals using properties of integration :

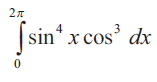
Solution :

Since the limit is 0 to 2π, we may use one of the properties.

If f(2a-x) = f(x)
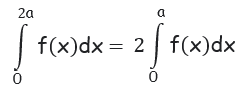If f(2a-x) = -f(x)

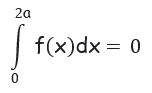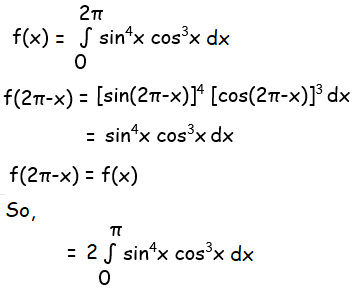
[sin(π-x)]⁴ [cos(π-x)]³ dx

= -sin⁴x cos³x dx

f(π-x) = -f(x)

So, the answer is 0.

Problem 2 :

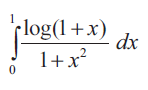
Solution :

Let f(x) = log (1+x)/(1+x²)

Applying x = tan θ then θ = tan^-1x

dx = sec²θ dθ

1+x² = 1+tan² θ

If x = 0, θ = tan^-1(0) ==>  0

If x = 1, θ = tan^-1(1) ==>  π/4

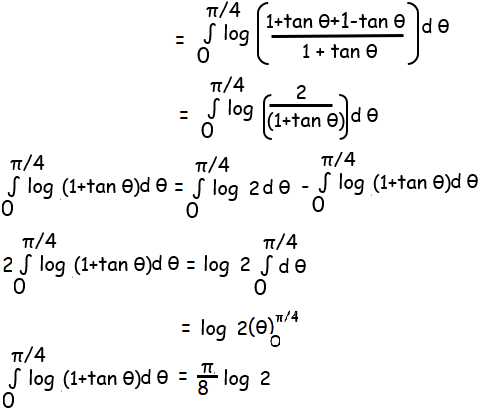
Problem 3 :

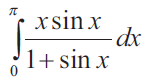
Solution :

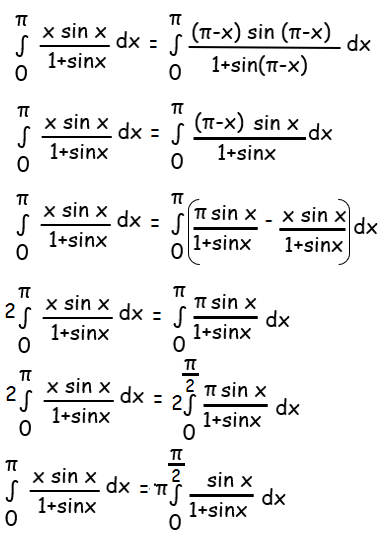
Multiplying by its conjugate, we get

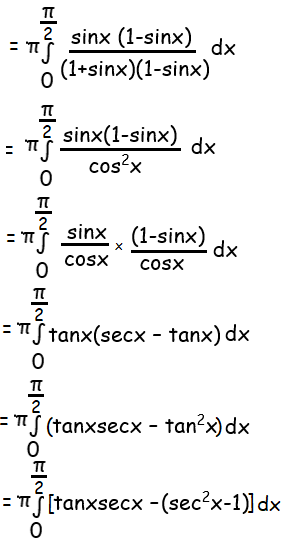
= π[sec x - tan x + x]

By applying the limits, we get

= π[sec(π//2)-tan(π/2)+(π/2) - (sec0-tan0+0)]

= π[(π/2) - 1]

= (π²/2)-π

Problem 4 :

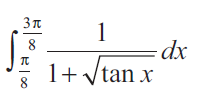
Solution :

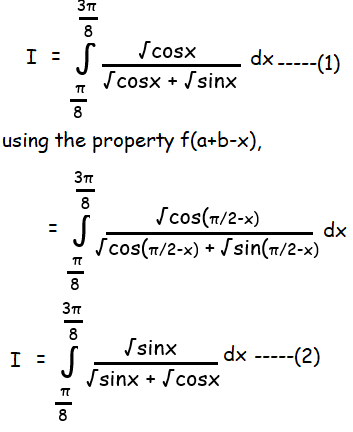
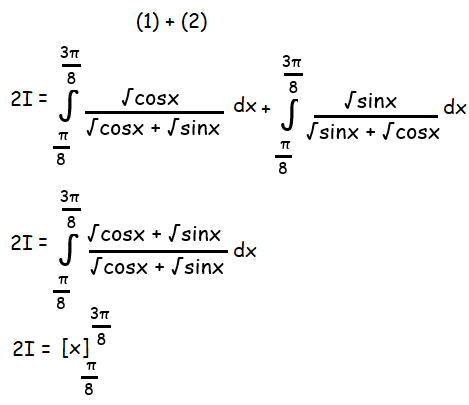
By applying limit, we get

2I = 3π/8 - π/8

2I = 2π/8

I = π/8

Problem 5 :

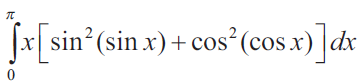
Solution :

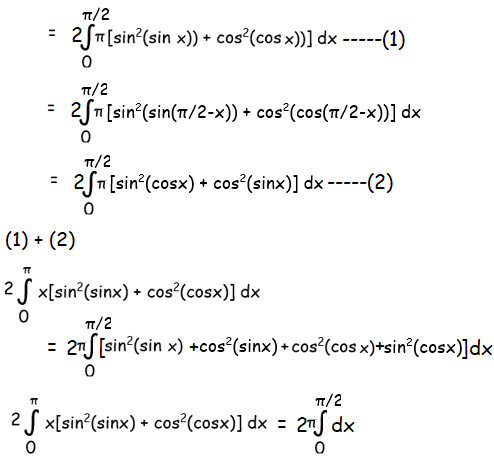
Dividing by 2 and integrating it, we get

= π[x] 0 to π/2

= π(π/2)

= π²/2

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.