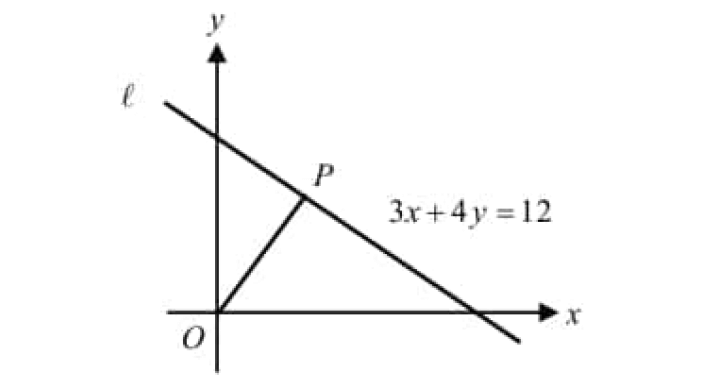
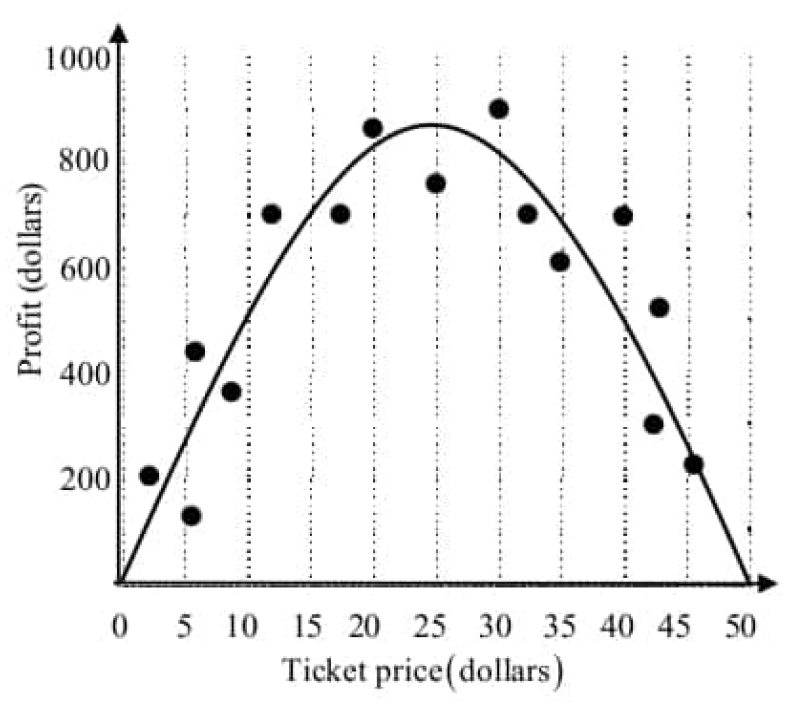
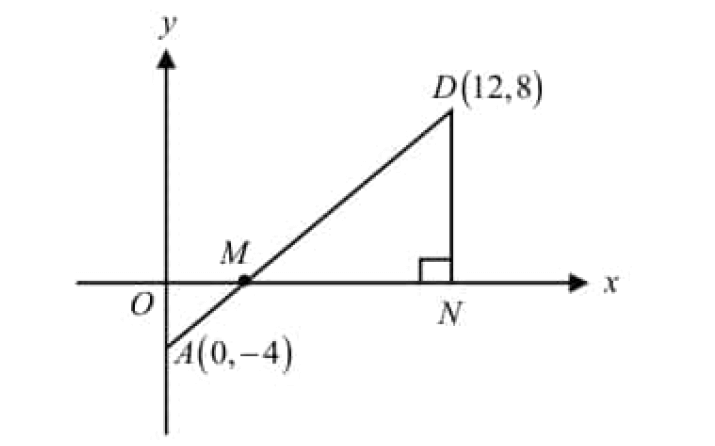
TYPES OF SURDS

Simple Surd

A surd having only one term is called a simple surd.

For example, 

√2, √3

Pure Surd

A surd which is completely irrational is called pure surd.

In other words, a surd which has unity only as rational factor the other factor being irrational is called pure surd

For example,

√3, √5

Similar Surds

Two or more surds are said to be similar or like surds if they have the same surd-factor.

√3, 7√3, 10√3, -2√3

All the above surds are similar or like surds. Because, we have the same surd factor √3.

And also, two or more surds are said to be similar or like surds if they can be so reduced as to have the same surd-factor.

√5, 7√125, √20, -5√45

Each of the above surds can be expressed with the same surd-factor √5.

7√125 = 7√(25 x 5)

= 7(5√5)

= 35√5

√20 = √(4 x 5)

= 2√5

-5√45 = -5√(9 x 5)

= -5(3√5)

= -15√5

The given surds can be expressed as

√5, 35√5, 2√5, -15√5

All the above surds are similar or like surds. Because, we have the same surd factor √5.

Mixed Surds

Surds which are not completely irrational and they can be expressed as a product of a rational number and an irrational number.

For example,

5√2, 7√3

Compound Surds

An expression which contains addition or subtraction of two or more surds is called compound surd.

For example,

√2 + √5, √7 - √11 + √3

Binomial Surd

A compound surd which contains exactly two surds is called a binomial surd.

3√2 + √3

Trinomial Surds

A compound surd which contains exactly three surds is called a trinomial surd.

√7 - √11 + √3

Conjugate Surds

Two binomial surds which are differ only in signs (+/–) between them are called conjugate surds.

For example,

√7 + √3 and √7 - √3

©All rights reserved. onlinemath4all.com