TYPES OF SOLUTIONS OF PAIR OF LINEAR EQUATIONS IN TWO VARIABLES

In this section, we will learn the types of solution of a pair of linear equations are having. By comparing the coefficients of linear equations, we may find what type of solution they have.

Example 1 :

Which of the following pairs of linear equations has unique solution, no solution, infinitely many solutions. In case there is unique solution, find it by using cross multiplication method.

(i)   x – 3y – 3  =  0

     3x – 9y – 2  =  0

Solution :

From the above information, let us take the values of a₁, a₂, b₁, b₂, c₁ and c₂

 a₁  =  1, b₁  =  -3, c₁  =  -3

 a₁  =  3, b₁  =  -9, c₁  =  -2

a₁/a₂  =  1/3

b₁/b₂  =  -3/(-9)  =  1/3

c₁/c₂  =  -3/(-2)  =  3/2

Here a₁/a₂  =  b₁/b₂  ≠  c₁/c₂

From this we can decide the given lines are parallel.

(ii)  2x + y  =  5

      3x + 2y  =  8

Solution :

2x + y – 5  =  0

3x + 2y – 8  =  0

From the above information, let us take the values of a₁, a₂, b₁, b₂, c₁ and c₂

 a₁  =  2, b₁  =  1, c₁  =  -5

 a₁  =  3, b₁  =  2, c₁  =  -8

a₁/a₂  =  2/3

b₁/b₂  =  1/2

c₁/c₂  =  (-5)/(-8) = 5/8

Here, a₁/a₂  ≠  b₁/b₂

Therefore two given lines are intersecting

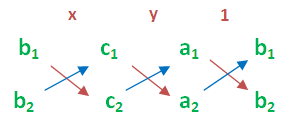
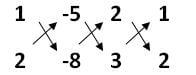
x/(-8  + 10)  =  y/(-15 + 16)  =  1/(4 – 3)

x/2  =  y/1  =  1/1

x/2  =  1           y/1  =  1

x  =  2           y  =  1

(iii) 3x – 5y  =  20

       6x – 10y  =  40

Solution :

  3 x – 5 y – 20  =  0 --------(1)

 6 x – 10 y – 40  =  0 --------(2)

From the above information, let us take the values of a₁, a₂, b₁, b₂, c₁ and c₂

 a₁  =  3, b₁  =  -5, c₁  =  -20

 a₁  =  6, b₁  =  -10, c₁  =  -40

a₁/a₂  =  3/6  =  1/2

b₁/b₂  =  -5/(-10)  =  1/2

c₁/c₂  =  (-20)/(-40)  =  1/2

here, a₁/a₂  =  b₁/b₂  =  c₁/c₂

Therefore the two given lines are coincident.

(iv) x – 3y – 7  =  0

     3x – 3y – 15  =  0

Solution :

From the above information, let us take the values of a₁, a₂, b₁, b₂, c₁ and c₂

 a₁  =  1, b₁  =  -3, c₁  =  -7

 a₁  =  3, b₁  =  -3, c₁  =  -15

a₁/a ₂ = 1/3

b₁/b ₂ = -3/(-3)  =  1

c₁/c ₂ = (-7)/(-15) = 7/15

here, a₁/a₂  ≠  b_1/ b₂

So, the given two lines are intersecting.

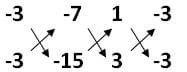
x/(45  - 21)  =  y/(-21 + 15)  =  1/(-3+9)

x/24  =  y/(-6)  =  1/6

x/24  =  1/6           y/(-6)  =  1/6

x  =  24/6               y  =  -6/6

x  =  4                       y  =  -1

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.