SUPPLEMENTARY ANGLES

Two angles are supplementary, if they add up to 180⁰. 

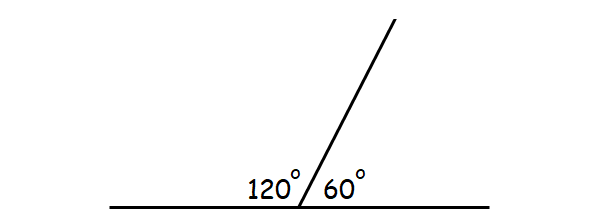
Example :

120° and 60° are supplementary angles.

Because,

120° + 60°  =  180°

Clearly, 120° is the supplement of 60° and 60° is the supplement of 120°.

Practice Problems

Problem 1 :

The measure of an angle is 108°. What is the measure of a supplementary angle?

Solution :

Let x be the measure of the required supplementary angle.

Because x and 108° are supplementary angles, 

x + 108°  =  180°

Subtract 108° from each side. 

x  =  72°

So, the measure of the supplementary angle is 72°.

Problem 2 :

The measure of an angle is 89°. What is the measure of a supplementary angle?

Solution :

Let x be the measure of the required supplementary angle.

Because x and 41° are supplementary angles, 

x + 89°  =  180°

Subtract 89° from each side. 

x  =  91°

So, the measure of the supplementary angle is 91°.

Problem 3 :

Find the value of  x :

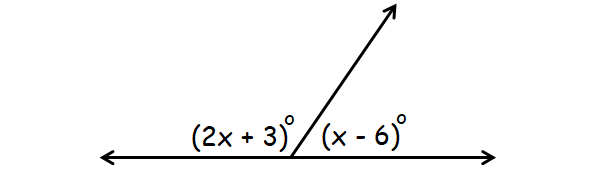
Solution :

From the picture above, it is clear that (2x+3) and (x-6) are supplementary angles. 

Then,

(2x+3) + (x-6)  =  180

2x + 3 + x - 6  =  180

Simplify. 

3x - 3  =  180

Add 3 to each side. 

3x  =  183

Divide each side by 3. 

x  =  61

So, the value of x is 61.

Problem 4 :

Find the value of  x :

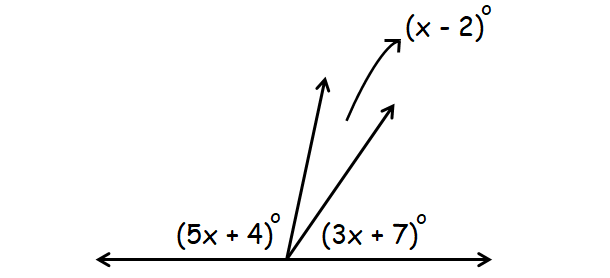
Solution :

From the picture above, it is clear (5x+4), (x-2) and (3x+7) are supplementary angles. 

Then, 

(5x+4) + (x-2) + (3x+7)  =  180

5x + 4 + x -2 + 3x + 7  =  180

Simplify. 

9x + 9  =  180

Subtract 9 from each side.

9x  =  171

Divide each side by 9.

x  =  19

So, the value of x is 19.

Problem 5 :

Two angles are supplementary. If one angle is double the other angle, find the two angles. 

Solution :

Let x be one of the angles. 

Then the other angle is 2x.

Because x and 2x are supplementary angles, 

x + 2x  =  180°

3x  =  180

Divide each side by 3. 

x  =  60

And,

2x  =  2(60)  =  120

So, the two angles are 60° and 120°.

Problem 6 :

Two angles are supplementary. If one angle is two times the sum of other angle and 3, find the two angles.

Solution :

Let x and y be the two angles which are supplementary.

So, we have

x + y  =  180° -----> (1)

Given : One angle is two times the sum of other angle and 3. 

Then, 

x  =  2(y + 3)

x  =  2y + 6 ----->(2)

Now, substitute (2y + 6) for x in (1). 

(1)-----> 2y + 6 + y  =  180

3y + 6  =  180

Subtract 6 from each side. 

3y  =  174

Divide each side by 3.

y  =  58

Substitute 58 for y in (2).

(2)-----> x  =  2(58) + 6

x  =  116 + 6

x  =  122

So, the two angles are 122° and 58°.

Apart from the stuff given above, if you need any other stuff, please use our google custom search here.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.

WORD PROBLEMS

HCF and LCM  word problems

Word problems on simple equations 

Word problems on linear equations 

Word problems on quadratic equations

Algebra word problems

Word problems on trains

Area and perimeter word problems

Word problems on direct variation and inverse variation 

Word problems on unit price

Word problems on unit rate 

Word problems on comparing rates

Converting customary units word problems 

Converting metric units word problems

Word problems on simple interest

Word problems on compound interest

Word problems on types of angles 

Complementary and supplementary angles word problems

Double facts word problems

Trigonometry word problems

Percentage word problems 

Profit and loss word problems 

Markup and markdown word problems 

Decimal word problems

Word problems on fractions

Word problems on mixed fractions

One step equation word problems

Linear inequalities word problems

Ratio and proportion word problems

Time and work word problems

Word problems on sets and Venn diagrams

Word problems on ages

Pythagorean theorem word problems

Percent of a number word problems

Word problems on constant speed

Word problems on average speed 

Word problems on sum of the angles of a triangle is 180 degree

OTHER TOPICS 

Profit and loss shortcuts

Percentage shortcuts

Times table shortcuts

Time, speed and distance shortcuts

Ratio and proportion shortcuts

Domain and range of rational functions

Domain and range of rational functions with holes

Graphing rational functions

Graphing rational functions with holes

Converting repeating decimals in to fractions

Decimal representation of rational numbers

Finding square root using long division

L.C.M method to solve time and work problems

Translating the word problems in to algebraic expressions

Remainder when 2 power 256 is divided by 17

Remainder when 17 power 23 is divided by 16

Sum of all three digit numbers divisible by 6

Sum of all three digit numbers divisible by 7

Sum of all three digit numbers divisible by 8

Sum of all three digit numbers formed using 1, 3, 4

Sum of all three four digit numbers formed with non zero digits

Sum of all three four digit numbers formed using 0, 1, 2, 3

Sum of all three four digit numbers formed using 1, 2, 5, 6