SOLVING PROBLEMS INVOLVING POLYNOMIALS

Problem 1 :

Factorize : x⁴ + 1. 

Solution :

x⁴ + 1  =  (x² + √2x + 1)(x² - √2x + 1)

Problem 2 :

If x² + x + 1 is a factor of the polynomial 3x³ + 8x² + 8x + a, then find the value of a.

Solution :

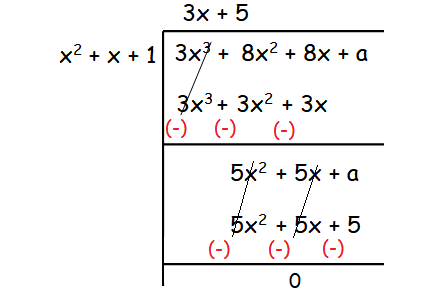
Hence the value of a is 5.

Problem 3 :

The equations x² - 6x + a = 0 and x² - bx + 6 = 0 have one root in common. The other root of the first and the second equations are integers in the ratio 4 : 3. Find the common root.

Solution :

Let α be the common root.

Let α, 4β be the roots of x² - 6x + a = 0.

Let α, 3β be the roots of x² - bx + 6 = 0.

Then, 4αβ = a and 3αβ = 6 which give αβ = 2 and a = 8.

The roots of x² - 6x + 8 = 0 are 2, 4.

If α = 2, then β = 1

If α = 4, then β = 1

2 which is not an integer.

Hence, the common root is 2.

Problem 4 :

Find the values of p for which the difference between the roots of the equation x² + px + 8 = 0 is 2.

Solution :

Let α and β be the roots of the equation x2 + px + 8 = 0.

Then, α + β = -p, αβ = 8 and |α - β| = 2.

Now, (α + β)² - 4αβ = (α - β)², which gives p² - 32 = 4.

Thus, p = ±6.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.