SOLVING INEQUALITIES USING MULTIPLICATION AND DIVISION

Example 1 : 

Solve the inequality given below. Graph and check the solution.

y/3 ≥ 5

Solution :

Step 1 : 

Solve the inequality 

y/3 ≥ 5

Use the Multiplication Property of Inequality.

Multiply both sides by 3. 

3.(y/3) ≥ (5).3

y ≥ 15

Step 2 : 

Graph the solution.

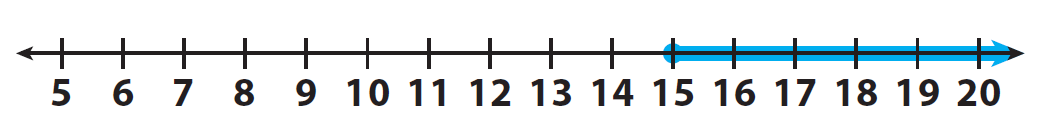
Step 3 :

Check the solution by substituting a solution from the shaded part of the graph into the original inequality. For convenience, choose a multiple of 3.

Substitute 18 for x into y/3 ≥ 5

18/3 ≥ 5

6 ≥ 5

The inequality is true.

Example 2 : 

Solve the inequality given below. Graph and check the solution.

-4x > 52

Solution :

Step 1 : 

Solve the inequality 

-4x > 52

Use the Division Property of Inequality.

Divide both sides by -4.  

(-4x) / (-4) < 52 / (-4)

x < -13

Step 2 : 

Graph the solution.

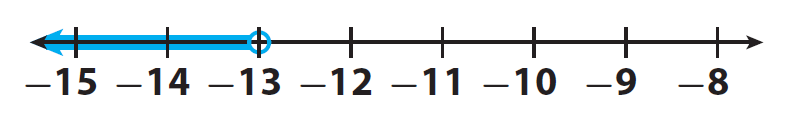
Step 3 :

Check the solution. Substitute a solution from the shaded part of your number line into the original inequality.

Substitute -15 for x into -4x > 52.

-4.(-15) > 52

60 > 52

The inequality is true.

Example 3 : 

Solve the inequality given below. Graph and check the solution.

-10y < 60

Solution :

Step 1 : 

Solve the inequality 

-10y < 60

Use the Division Property of Inequality.

Divide both sides by -10. 

(-10y) / (-10) > 60 / (-10)

y > -6

Step 2 : 

Graph the solution.

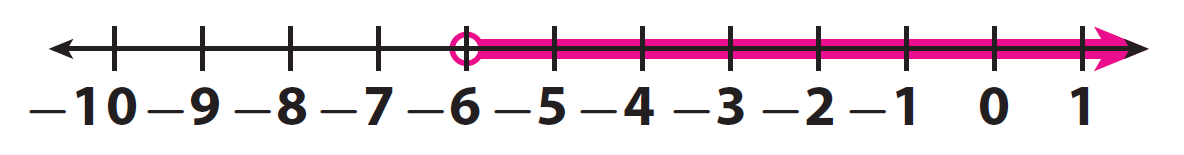
Step 3 :

Check the solution. Substitute a solution from the shaded part of your number line into the original inequality.

Substitute -2 for y into -10y < 60.

(-10).(-2) < 60

20 < 60

The inequality is true.

Example 4 : 

Solve the inequality given below. Graph and check the solution.

7 ≥ -t/6

Solution :

Step 1 : 

Solve the inequality 

7 ≥ -t/6

Use the Multiplication Property of Inequality.

Multiply both sides by -6. 

7.(-6) ≤ (-t/6).(-6)

-42 ≤ t

or t ≥ -42 

Step 2 : 

Graph the solution.

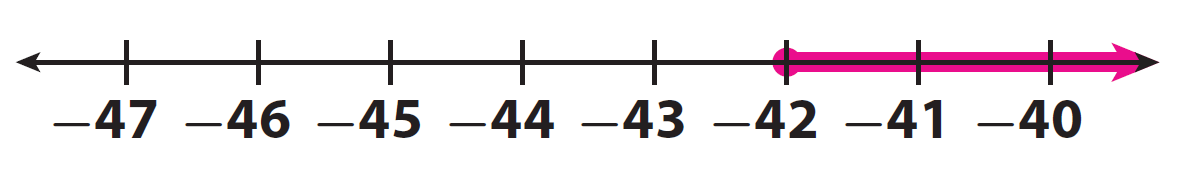
Step 3 :

Check the solution. Substitute a solution from the shaded part of your number line into the original inequality.

Substitute -40 for t into 7 ≥ -t/6.

7 ≥ -(-40)/6

7 ≥ 20/3

7 ≥ 6.67

The inequality is true.

Apart from the stuff given above, if you need any other stuff in math, please use our google custom search here.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.