SOLVE A SYSTEM OF EQUATIONS BY GRAPHING

Solve the following systems of linear equations by graphing :

Example 1 :

y = x – 3

y = 3

Solution :

y = x – 3 :

The given equation is in slope-intercept form. Substitute some random values for x and find their corresponding values of y.

When x = 0,

y = 0 - 3

= -3

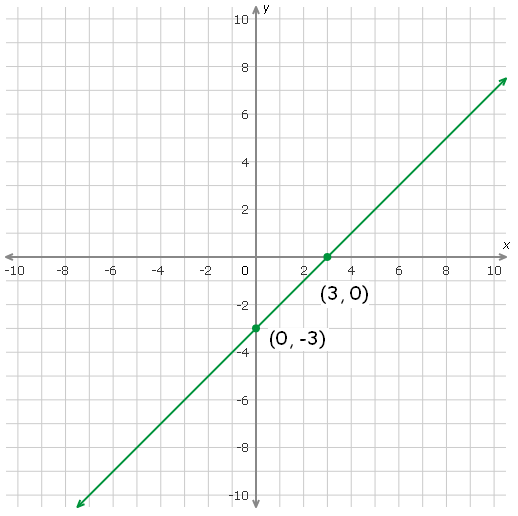
(0, -3)

When x = 3,

y = 3 - 3

= 0

(3, 0)

Plot the points (0, -3), and (3, 0) on a xy-plane and connect them.

y = 3 :

This is the equation of a line parallel to x-axis through the value 3 on y-axis.

So, graph a straight parallel to x-axis through the value 3 on y-axis.

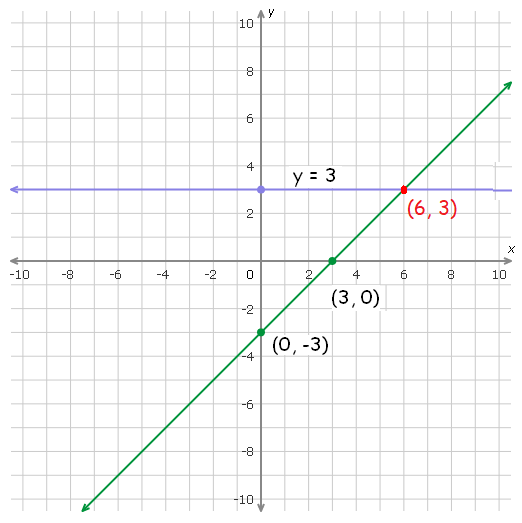
In the graph above, the two lines intersect at (6, 3).

So, 

x = 6 and y = 3

Example 2 :

2x + y - 2 = 0

5x + y + 1 = 0

Solution :

2x + y - 2 = 0 :

The given equation is not in slope-intercept form. Write the given equation is in slope intercept form.

2x + y - 2 = 0

Subtract 2x from both sides.

y - 2 = -2x

Add 2 to both sides.

y = -2x + 2

Now the equation is in slope-intercept form. Substitute some random values for x and find their corresponding values of y.

When x = -1,

y = -2(-1) + 2

= 2 + 2

= 4

(-1, 4)

When x = 0,

y = -2(0) + 2

= 0 + 2

= 2

(0, 2)

Plot the points (-1, 4), and (0, 2) on a xy-plane and connect them.

5x + y + 1 = 0 :

The given equation is not in slope-intercept form. Write the given equation is in slope intercept form.

5x + y + 1 = 0

Subtract 5x and 1 from both sides.

y = -5x - 1

Now the equation is in slope-intercept form. Substitute some random values for x and find their corresponding values of y.

When x = -1,

y = -5(-1) - 1

= 5 - 1

= 4

(-1, 4)

When x = 0,

y = -5(0) - 1

= 0 - 1

= -1

(0, -1)

Plot the points (-1, 4), and (0, -1) on a xy-plane and connect them.

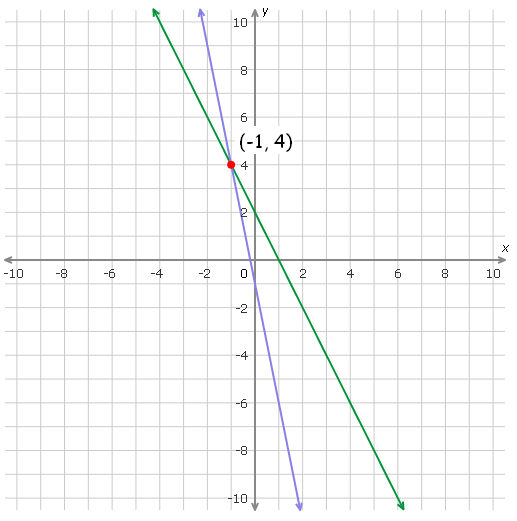
In the graph above, the two lines intersect at (-1, 4).

So, 

x = -1 and y = 4

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.