SOLID MENSURATION PRACTICE PROBLEMS

Problem 1 :

The barrel of a fountain-pen cylindrical in shape, is 7 cm long and 5 mm in diameter. A full barrel of ink in the pen will be used for writing 330 words on an average. How many words can be written using a bottle of ink containing one fifth of a liter?

Solution :

To find the number of words can be written using a bottle of ink, we have to find the quantity of ink in the barrel.

Volume of barrel in the cylindrical shape  =  πr²h

height of shape  =  7 cm

radius of the shape  =  (5/2) mm

10 mm  =  1 cm

(5/2)/10  =  (1/4) cm

Volume of barrel in the cylindrical shape :

  =  (22/7) ⋅ (1/4) (1/4) 7

  =  (11/8) cm³

1000 cm³  =  1 liter

  =  11/8000 liter

Quantity of ink in 1 liter  =  8000/11

Quantity of ink in the bottel =  (1/5) of liter of ink in the barrel

   =  (1/5) (8000/11) 

Number of word written using 1 bottel of ink  =  330

Required number of words  =   (1/5) (8000/11) ⋅ (1/4) 

=  48000 words

Problem 2 :

A hemi-spherical tank of radius 1.75 m is full of water. It is connected with a pipe which empties the tank at the rate of 7 liters per second. How much time will it take to empty the tank completely?

Solution :

To solve this problem, first let us find quantity of water in the tank in liters.

Volume of water in the hemispherical tank  =  (2/3)πr³

  =  (2/3) ⋅ (22/7) ⋅ (1.75)³

  =  (2/3) ⋅ (22/7)  (1.75)  (1.75)  (1.75)

  =  235.81/21

  =  11.22 m³

1 m³  =  1000 liter

=  11.22 (1000) 

=  11220 liters

Quantity of water empties  =   7 liter per second

=  7 (60)

=  420 liter per minute

Time taken to empty the tank  =  11220/420

=  26.71

=  27 minutes (approximately)

Problem 3 :

Find the maximum volume of a cone that can be carved out of a solid hemisphere of radius r units.

Solution :

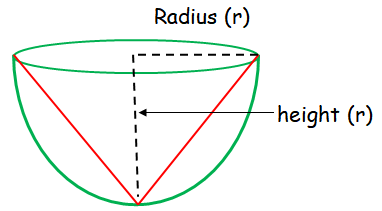
Volume of cone craved out from the hemisphere 

  =  (1/3) r²h

  =  (1/3) r²r

  =  (1/3) r³

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.