SLOPE OF A LINE

It is the change in y for a unit change in x along the line and usually denoted by the letter m.

Slope is sometimes referred to as 'rise over run'.

Because the fraction consists of the rise (the change in y, going up or down) divided by the run (the change in x, going from left to the right).

The figures shown below illustrate this. 

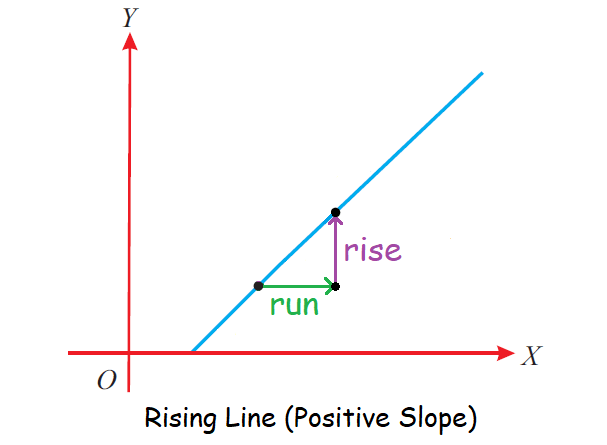
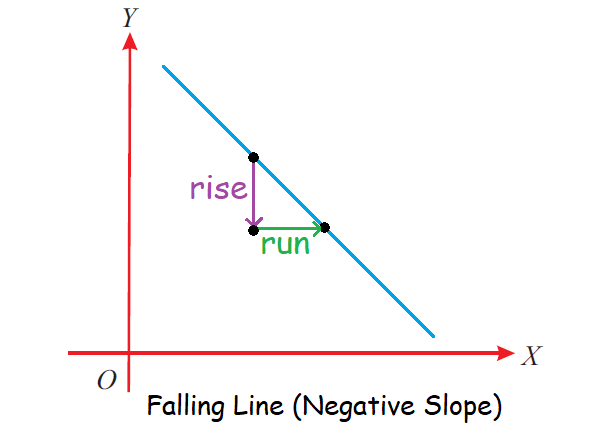
The simplest way to look at the slope is

ʳⁱˢᵉ⁄ᵣᵤₙ

(rise over run)

In the formula (ʳⁱˢᵉ⁄ᵣᵤₙ), we can "rise" up or down... but, we ALWAYS "run" to the right.

If the "rise" is up, it will have a positive value and if the "rise" is down, it will have a negative value.

Finding Slope Using the Two Points on the Line

From the above figure, the slope of the straight line joining the points A (x₁, y₁) and B (x₂, y₂) is

That is,

If the equation of a straight line given in general form

ax + by + c = 0,

then, the formula to find slope of the line is

Let θ be the angle between the straight line l and the positive side of x - axis.

The figure given below illustrates this.

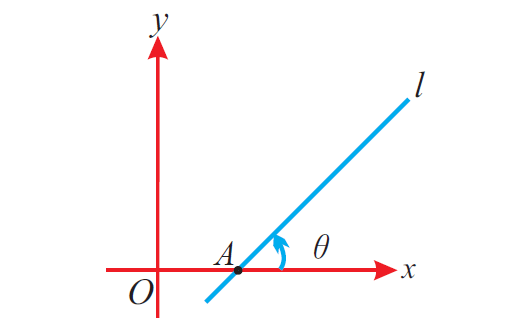
Then, the formula to find slope of the line is

m = tanθ

In the general form of equation of a straight line

ax + by + c = 0,

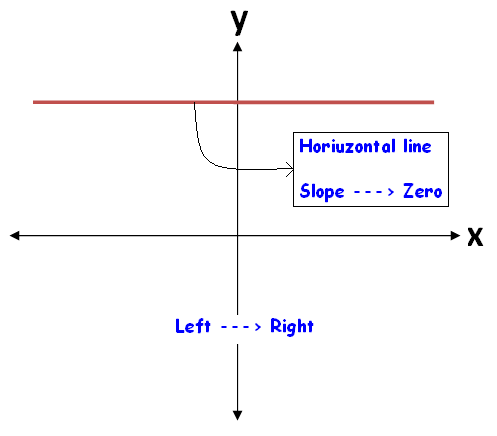
(i)  If x term is missing, then the line will be parallel to x-axis and its slope will be zero.

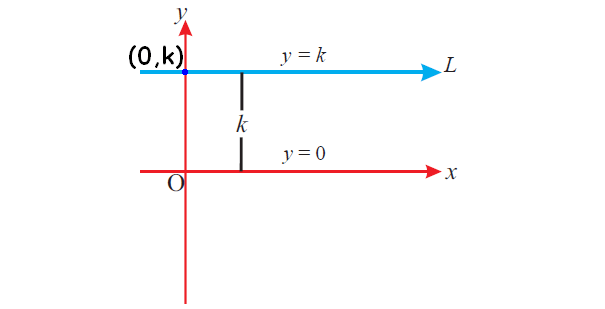
We know that

In the above figure, the value of y is fixed and that is k.

So, there is no change in y and change in y = 0.

(i) If y term is missing, then the line will be parallel to y-axis and its slope will be undefined. 

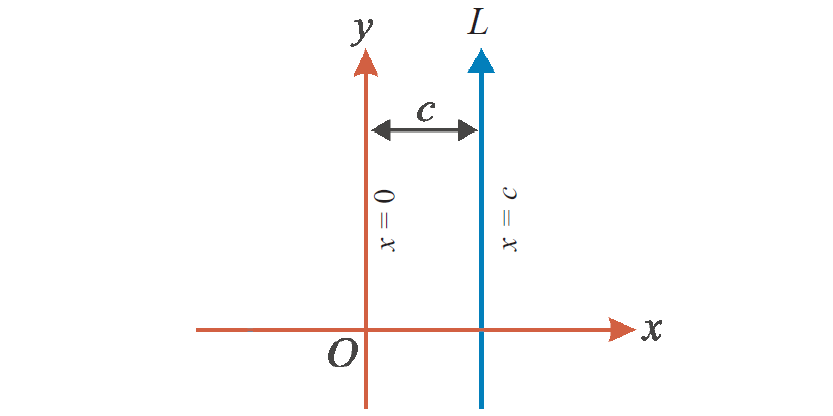
In the above figure, the value of x is fixed and that is c.

So, there is no change in x and change in x = 0.

Slope of the coordinate axes x and y.

(i) Slope of x axis zero.

(ii) Slope of y axis undefined.

Slope of a Line - Positive or Negative or Zero or Undefined

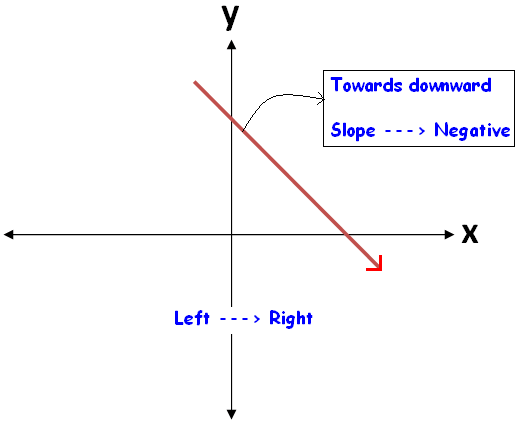
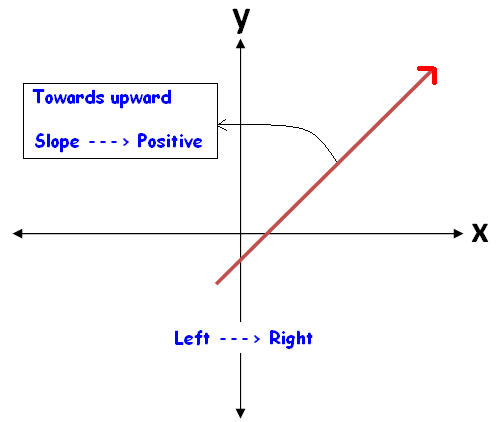
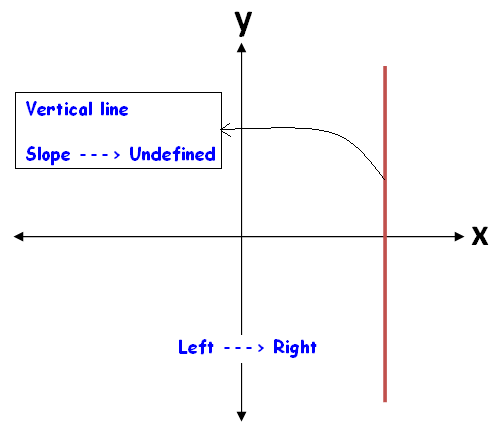
When we look at a straight line visually, we can come to know its slope easily.

To know the sign of slope of a straight line, always we have to look at the straight line from left to right.

The figures given below illustrate this.

Solved Problems

Problem 1 :

Find the angle of inclination of the straight line whose slope is 1/√3.

Solution :

Let θ be the angle of inclination of the line.

Then, slope of the line, m = tan θ.

Given : Slope = ¹⁄√₃.

So, we have

tanθ = ¹⁄√₃

θ = 30°

So, the angle of inclination is 30°.

Problem 2 :

Find the slope of the straight line passing through the points (3, -2) and (-1, 4).

Solution :

Formula to find slope of a line joining (x₁, y₁) and (x₂, y₂) :

Substitute (x₁, y₁) = (3, -2) and (x₂, y₂) = (-1, 4).

Problem 3 :

Using the concept of slope, show that the points A(5, -2), B(4, -1) and C(1, 2) are collinear.

Solution :

Formula to find slope of a line joining (x₁, y₁) and (x₂, y₂) :

Using the above formula, slope of the line AB joining the points A (5, - 2) and B (4- 1) :

Slope of the line BC joining the points B (4- 1) and C (1, 2) :

slope of AB = slope of BC

Also, B is the common point.

So, the points A, B and C are collinear.

Problem 4 :

Find the slope of the line 3x - 2y + 7 = 0.

Solution :

When the general form of equation of a straight line is given, the formula to find slope is

In the given line 3x - 2y + 7 = 0, coefficient of x =3 and coefficient of y = -2.

Then the slope is

m = -³⁄₍₋₂₎

m = ³⁄₂

Problem 5 :

If the straight line 5x + ky - 1 = 0 has the slope 5, find the value of k.

Solution :

When the general form of equation of a straight line is given, the formula to find slope is

In the given line 3x - 2y + 7 = 0, coefficient of x = 3 and coefficient of y = k.

Then the slope is

m = -⁵⁄k

Given : Slope = 5.

5 = -⁵⁄k

Multiply both sides by k.

k = -1

Divide both sides by 5.

k  =  -1

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.