SIMPLIFYING RADICALS WORKSHEET

Problem 1 :

Simplify : 

√20

Problem 2 : 

Simplify : 

√121

Problem 3 : 

Simplify : 

√52

Problem 4 : 

Simplify : 

√45

Problem 5 : 

Simplify : 

³√72

Problem 6 : 

Simplify : 

³√40

Problem 7 : 

Simplify : 

³√27

Problem 8 : 

Simplify : 

⁴√243

Problem 9 : 

Simplify : 

⁵√288

Problem 10 : 

Simplify : 

⁶√320

Detailed Answer Key

Problem 1 :

Simplify : 

√20

Solution : 

Decompose 20 into prime factors using synthetic division. 

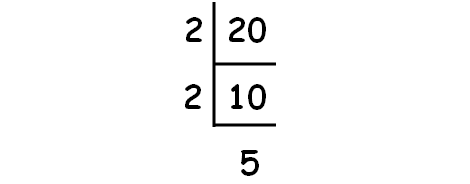
So, we have

√20  =  √(2 ⋅ 2 ⋅ 5)

√20  =  2√5

Problem 2 : 

Simplify : 

√121

Solution : 

Decompose 121 into prime factors.

√121  =  √(11 ⋅ 11)

√121  =  11

Problem 3 : 

Simplify : 

√52

Solution : 

Decompose 52 into prime factors using synthetic division.

So, we have

√52  =  √(2 ⋅ 2 ⋅ 13)

√52  =  2√13

Problem 4 : 

Simplify : 

√45

Solution : 

Decompose 45 into prime factors using synthetic division.

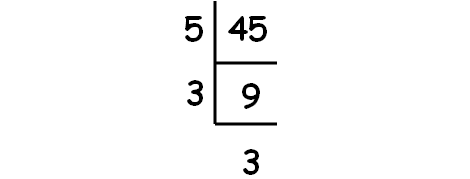
So, we have

√45  =  √(5 ⋅ 3 ⋅ 3)

√45  =  3√5

Problem 5 : 

Simplify : 

³√72

Solution : 

Decompose 72 into prime factors using synthetic division.

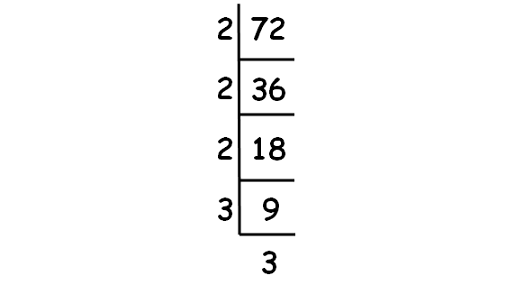
So, we have

³√72  =  ³√(2 ⋅ 2 ⋅ 2 ⋅ 3 ⋅ 3)

³√72  =  2 ⋅ ³√(3 ⋅ 3)

³√72  =  2 ⋅ ³√(3 ⋅ 3)

³√72  =  2³√9

Problem 6 : 

Simplify : 

³√40

Solution : 

Decompose 40 into prime factors using synthetic division.

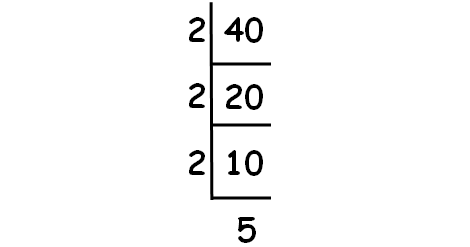
So, we have

³√40  =  ³√(2 ⋅ 2 ⋅ 2 ⋅ 5)

³√40  =  2³√5

Problem 7 : 

Simplify : 

³√27

Solution : 

Decompose 27 into prime factors using synthetic division.

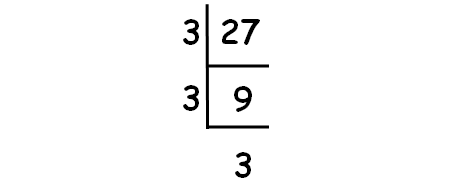
So, we have

³√27  =  ³√(3 ⋅ 3 ⋅ 3)

³√27  =  3

Problem 8 : 

Simplify :  

⁴√243

Solution : 

Decompose 243 into prime factors using synthetic division.

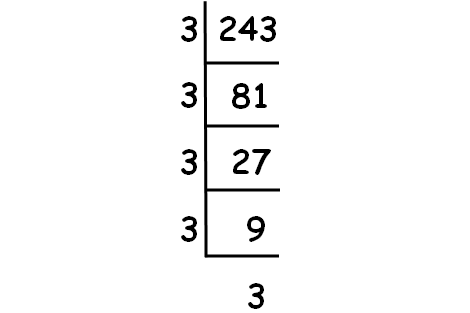
So, we have

⁴√243  =  √(3 ⋅ 3 ⋅ 3 ⋅ 3 ⋅ 3)

⁴√243  =  3√3

Problem 9 : 

Simplify : 

⁵√288

Solution : 

Decompose 288 into prime factors using synthetic division.

So, we have

⁵√288  =  ⁵√(2 ⋅ 2 ⋅ 2 ⋅ 2 ⋅ 2 ⋅ 3)

⁵√288  =  2√3

Problem 10 : 

Simplify : 

⁶√320

Solution : 

Decompose 320 into prime factors using synthetic division.

So, we have

⁶√320  =  ⁶√(2 ⋅ 2 ⋅ 2 ⋅ 2 ⋅ 2 ⋅ 2 ⋅ 5)

⁶√320  =  2√5

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.