RELATING SI AND IMPERIAL UNITS

Each measurement in the imperial system relates to a corresponding measurement in the SI system.

This table shows some approximate relationships between imperial units and SI units.

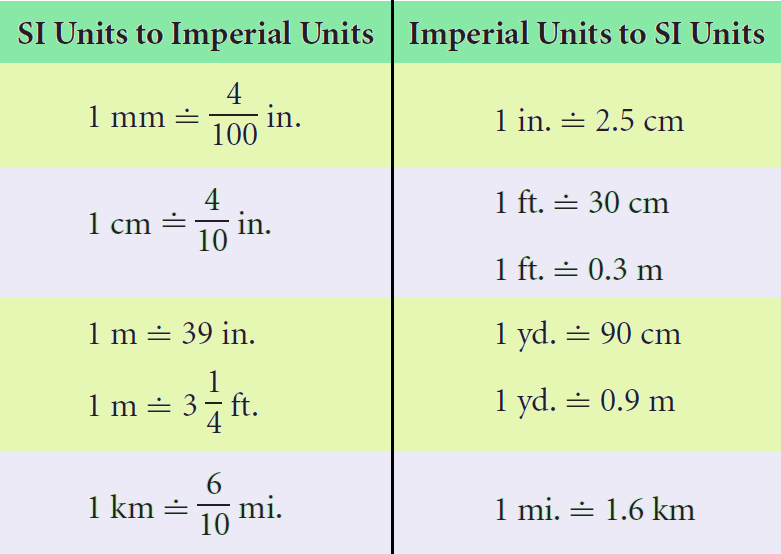
We can use the data in the table above to convert between SI and imperial units of measure.

Converting from Meters to Feet

Example 1 :

A bowling lane is approximately 20 m long. What is this measurement to the nearest foot?

Solution :

From the table above 1 m ≈ 3¼ ft.

20 m ≈ 20(3¼ ft.)

20 m ≈ 20(13/4 ft.)

20 m ≈ 65 ft.

A length of 20 m is approximately 65 ft.

Converting between Miles and Kilometers

Example 2 :

After meeting in New York, James drove 62 mi. south and Lily drove 98 km north. Who drove farther?

Solution :

To compare the distances, convert one measurement so the units are the same.

Method 1 :

Convert the distance James drove from miles to kilometers.

1 mi. ≈ 1.6 km

So,

62 mi. ≈ 62(1.6 km)

62 mi. ≈ 99.2 km

Since 99.2 km > 98 km, James drove farther.

Method 2 :

Convert the distance Lily drove from kilometers to miles.

1 km ≈ 6/10 mi.

So,

98 km ≈ 98(6/10 mi.)

98 km ≈ 98(0.6 mi.)

98 km ≈ 58.8 mi.

Since 62 mi. > 58.8 mi., James drove farther.

Solving a Problem that Involves Unit Conversions

Example 3 :

Lenin is 6 ft. 2 in. tall. To list his height on his driver’s license application, Lenin needs to convert this measurement to centimeters.

(a) What is Alex’s height to the nearest centimeter?

(b) Use mental math and estimation to justify that the answer is reasonable.

Solution :

Part (a) :

Convert 6 ft. 2 in. to inches.

1 ft. = 12 in.

So,

6 ft. = 6(12 in.)

6 ft. = 72 in.

6 ft. 2 in. = 72 in. + 2 in.

6 ft. 2 in. = 74 in.

Write a proportion to convert 74 in. to centimeters. Let h represent Lenin’s height in centimeters. The ratio of h centimeters to 74 in. is approximately equal to the ratio of 1 cm to 4/10 in.

h : 74 ≈ 1 : 4/10

h : 74 ≈ 1 : 0.4

h/74 ≈ 1/0.4

h/74 ≈ 2.5

Multiply both sides by 74.

h ≈ 185 cm

Lenin is about 185 cm tall.

Part (b) :

To check :

1 ft. ≈ 30 cm

6 ft. ≈ 6(30 cm)

6 ft. ≈ 180 cm

So, 6 ft. 2 in. ≈ 185 cm is reasonable.

Estimating and Calculating Using Unit Conversions

Example 4 :

A truck driver knows that her semitrailer is 3.5 m high. The support beams of a bridge are 11 ft. 9 in. high. Will the vehicle fit under the bridge? Justify the answer.

Answer :

Write a proportion to convert 3.5 m to feet. Let h represent the height of the vehicle in feet. The ratio of h feet to 3.5 m is approximately equal to the ratio of 1 ft. to 0.3 m.

h : 3.5 ≈ 1 : 0.3

h/3.5 ≈ 1/0.3

Multiply both sides by 3.5.

h ≈ 3.5/0.3

h ≈ 11.6666..... or 11⅔

The vehicle is about 11⅔ ft., or 11 ft. 8 in. high ; so it should fit under the bridge.

This height is an estimate that is very close to the bridge height. To be sure the vehicle will fit, calculate an exact conversion. Convert the height of the vehicle in centimeters to inches.

Use the conversion: 2.54 cm = 1 in.

1 cm = (1/2.54) in.

350 cm = 350(1/2.54) in.

350 cm = (350/2.54) in.

350 cm = 137.7952.... in.

350 cm = (137.7952..../12) ft.

350 cm = 11.4869.... ft.

This measurement is a little less than 11½ ft. or 11 ft. 6 in., so the vehicle will fit under the bridge.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.