QUARTILES IN STATISTICS

Quartiles are the values which divide the given data into four equal parts. To deal with quartiles, first we have to arrange the values in the given data in ascending order, that is, from least to greatest. Assume a rectangle, where the data is arranged from least to greatest as shown below. 

If we want to divide the rectangle above into four equal parts, we need three lines in the middle at equal distance. Those three lines are called quartiles.

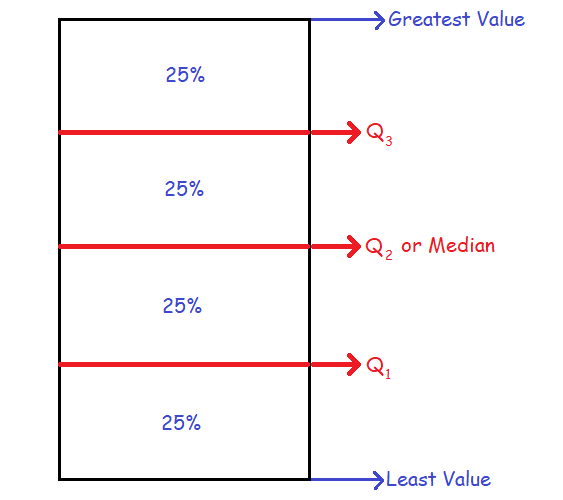
In the picture above,

Q₁ ----> first or lower quartile

Q₂ ----> second quartile or median

Q₃ ----> third or upper quartile

Since quartiles divide a data into four equal parts, they can also be called as partition values.

Example 1 :

Following are the wages of the 11  labourers :

$82, $56, $90, $50, $48, $99, $77, $75, $80, $97, $65

Find Q₁, Q₂ and Q₃ for the above data.

Solution :

Arrange the given data in ascending order :

$48, $50, $56, $65, $75, $77, $80, $82, $90, $97, $99

To get the first quartile, find the value of ⁽ⁿ ⁺ ¹⁾⁄₄.

= ⁽ⁿ ⁺ ¹⁾⁄₄

= ⁽¹¹ ⁺ ¹⁾⁄₄

= ¹²⁄₄

= 3

First Quartile (Q₁) :

= [⁽ⁿ ⁺ ¹⁾⁄₄]^th value in the ascending order of the data

= 3^rd value in the ascending order of the data

= $56

To get the second quartile, find the value of ⁽ⁿ ⁺ ¹⁾⁄₂.

= ⁽ⁿ ⁺ ¹⁾⁄₂

= ⁽¹¹ ⁺ ¹⁾⁄₂

= ¹²⁄₂

= 6

Second Quartile or Median (Q₂) :

= [⁽ⁿ ⁺ ¹⁾⁄₂]^th value in the ascending order of the data

= 6^th value in the ascending order of the data

= $77

To get the third quartile, find the value of ³⁽ⁿ ⁺ ¹⁾⁄₄.

= ³⁽ⁿ ⁺ ¹⁾⁄₄

= ³⁽¹¹ ⁺ ¹⁾⁄₄

= ³⁽¹²⁾⁄₄

= 3(3)

= 9

Third Quartile (Q₃) :

= [³⁽ⁿ ⁺ ¹⁾⁄₄]^th value in the ascending order of the data

= 9^th value in the ascending order of the data

= $80

Example 2 :

Ten values in of a data are arranged in ascending order as shown below.

12, 14, 17, 19, 26, 31, 33, 45, 49, 58

Find Q₁, Q₂ and Q₃ for the above data.

Solution :

The values in the data are already arranged in ascending order.

12, 14, 17, 19, 26, 31, 33, 45, 49, 58

Find find the value of ⁽ⁿ ⁺ ¹⁾⁄₄.

= ⁽ⁿ ⁺ ¹⁾⁄₄

= ⁽¹⁰ ⁺ ¹⁾⁄₄

= ¹¹⁄₄

= 2.75

First Quartile (Q₁) :

= [⁽ⁿ ⁺ ¹⁾⁄₄]^th value in the ascending order of the data

= 2.75^th value in the ascending order of the data

= 2^nd value + 0.75 x difference between the 3^rd and 2^rd values

= 14 + 0.75(17 - 14)

= 14 + 0.75(3)

= 14 + 2.25

= 16.25

To get the second quartile, find the value of ⁽ⁿ ⁺ ¹⁾⁄₂.

= ⁽ⁿ ⁺ ¹⁾⁄₂

= ⁽¹⁰ ⁺ ¹⁾⁄₂

= ¹¹⁄₂

= 5.5

Second Quartile or Median (Q₂) :

= [⁽ⁿ ⁺ ¹⁾⁄₂]^th value in the ascending order of the data

= 5.5^th value in the ascending order of the data

(5.5 is the exact middle value of 5 and 6. So, you can find the average of 5^th and 6^th values in the ascending order of the given data to get the second quartile or median)

= 28.5

To get the third quartile, find the value of ³⁽ⁿ ⁺ ¹⁾⁄₄.

= ³⁽ⁿ ⁺ ¹⁾⁄₄

= ³⁽¹⁰ ⁺ ¹⁾⁄₄

= ³⁽¹¹⁾⁄₄

= 3(2.75)

= 8.25

Third Quartile (Q₃) :

= [³⁽ⁿ ⁺ ¹⁾⁄₄]^th value in the ascending order of the data

= 8.25^th value in the ascending order of the data

= 8^th value + 0.25 x difference between the 8^th and 9^th values

= 45 + 0.25(49 - 45)

= 45 + 0.25(4)

= 45 + 1

= 46

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.