PROPERTIES OF PARALLELOGRAMS WORKSHEET

Problem 1 :

In the parallelogram given below, find ∠B, ∠C and ∠D.

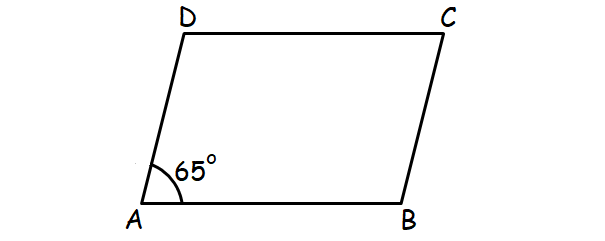
Problem 2 :

In the parallelogram ABCD given below, find ∠A, ∠B, ∠C and ∠D.

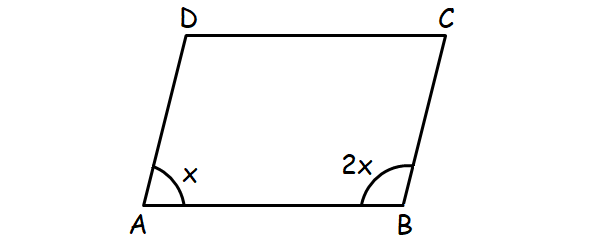
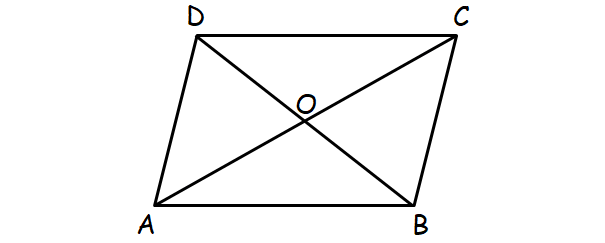
Problem 3 :

In the parallelogram given below, find the measures of ∠ABO and ∠ACB.

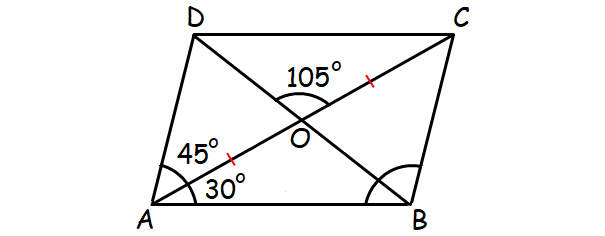
Problem 4 :

The perimeter of the parallelogram ABCD shown below is 30 units and the length of the side AB is 9 units, find the length of other sides of the parallelogram.

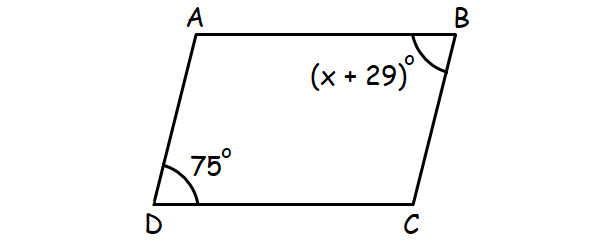
Problem 5 :

In the parallelogram given below, find the value of x, measures of ∠A and ∠C.

Problem 6 :

In the parallelogram given below,

AO = x + 40

OC = 2x + 18

Find the length of AO and OC.

Problem 7 :

In two adjacent angles of a parallelogram, if one angle is four times of the other, then find the measures of the two angles.

Problem 8 :

In the parallelogram given above, find the lengths of the sides AB and CD.

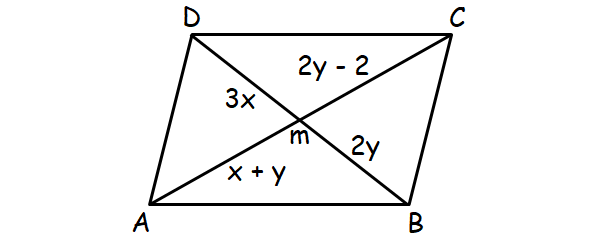
Problem 9 :

In the parallelogram given below, find the values of x and y.

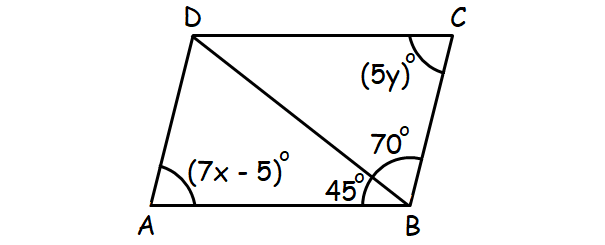
Problem 10 :

In the parallelogram given below, find the values of x and y.

1. Answer :

In a parallelogram, adjacent angles are supplementary.

In the above parallelogram, ∠A and ∠B are adjacent angles.

∠A + ∠B = 180°

65° + ∠B = 180°

∠B = 115°

Because opposite angles are congruent, we have

Hence, the measures of ∠B, ∠C and ∠D are 115°, 65° and 115° respectively.

2. Answer :

In a parallelogram, adjacent angles are supplementary.

In the above parallelogram, ∠A and ∠B are adjacent angles.

x + 2x = 180°

3x = 180°

 x = 60°

The measure of angle ∠A is

= x

= 60°

The measure of angle ∠B is

= 2x

= 2 ⋅ 60°

= 120°

According to the properties of parallelogram, the opposite angles are congruent.

Hence, the measures of ∠A, ∠B, ∠C and ∠D are 60°, 120°, 60° and 120° respectively.

3. Answer :

In the parallelogram given above ∠AOB and ∠COD are vertically opposite angles.

Because vertically opposite angles are equal, we have

∠AOB = ∠COD

∠AOB = 105°

In triangle ABO, we have

∠OAB + ∠AOB + ∠ABO = 180°

Substitute ∠OAB = 30° and ∠AOB = 105°.

30° + 105° + ∠ABO = 180°

135° + ∠ABO = 180°

∠ABO = 45°

In the parallelogram given above, AD||BC, AC is transversal and ∠OCB and ∠OAD are alternate interior angles.

If two parallel lines are cut by a transversal, alternate interior angles are equal.

∠OCB = ∠OAD

In the parallelogram given above, ∠OAD = 45°.

Then,

∠OCB = 45°

Because ∠OCB  ≅ ∠ACB, we have

∠ACB = 45°

Hence, the measures of ∠ABO and ∠ACB are 45° each.

4. Answer :

Given : Perimeter of the parallelogram is 30 units.

AB + BC + CD + AD = 30 ----(1) 

Because it is parallelogram, length of opposite sides must be equal.

AB = CD

AD = BC

Given : AB = 9 units.

In a parallelogram, opposite sides are equal, so AB = CD.

AB = CD = 6.

(1)----> 9 + BC + 9 + AD = 30

18 + BC + AD = 30  

BC + AD = 12

Because AD = BC,

AD + AD = 12 

2 ⋅ AD = 12

AD = 6

Then, the length of BC is also 6 units.

Hence, the length of CD is 9 units, AD and BC are 6 units each. 

5. Answer :

According to the properties of parallelogram, opposite angles are equal.

∠B = ∠D

(x + 29)° = 75°

x + 29 = 75

x = 46

In a parallelogram, adjacent angles are supplementary.

∠D + ∠C = 180°

75° + ∠C = 180°

∠C = 105°

In a parallelogram, opposite angles are equal.

∠A = ∠C

∠A = 105°

Hence, the measures of ∠A and ∠C are 105° each.

6. Answer :

AO = x + 40

OC = 2x + 18

According to the properties of parallelogram, the diagonals bisect each other.

AO = OC

x + 40 = 2x + 18

40 = x + 18

x = 22

Length of AO :

AO = x + 40

AO = 22 + 40

AO = 62

Length of OC :

OC = 2x + 18

OC = 2⋅ 22 + 18

OC = 44 + 18

OC = 62

Hence, the lengths of AO and OC are 62 units each.

7. Answer :

Let x be one of the angles.

Then, the adjacent angle of x is 4x

In a parallelogram, adjacent angles are supplementary.

x + 4x = 180°

5x = 180°

x = 36°

Then, the measure of the adjacent angle is

= 4x

= 4 ⋅ 36°

  = 144°

Hence, the measures of the two adjacent angles are 36° and 144°.

8. Answer :

According to the properties of parallelogram, the length of opposite sides are equal.

Length of AB = Length of CD

5x = x + 44

4x = 44

x = 11

Length of AB:

AB = 5x

= 5 ⋅ 11

= 55

Because opposite sides are equal, the length of CD is also 55 units.

Hence, the lengths of AB and CD are 55 units each. 

9.Answer :

According to the properties of parallelogram, the diagonals of a parallelogram bisect each other.

From the diagonal AC, we have

x + y = 2y - 2

x =  y - 2 ----(1)

From the diagonal BD, we have

3x = 2y ----(2)

Substitute x = y - 2 in (2).

3(y - 2) = 2y

3y - 6 = 2y

y = 6

Substitute y = 6 in (1).

x = 6 - 2

x = 4

Hence, the value of x is 4 and y is 6.

10. Answer :

In the parallelogram given above, the measure of angle Y is

∠Y = 45° + 70°

∠Y = 115°

In a parallelogram, adjacent angles are supplementary.

Because ∠F and ∠Y are supplementary, we have

 ∠F + ∠Y = 180°

Substitute ∠F = (7x - 5)° and ∠Y = 115°.

(7x - 5)° + 115° = 180°

7x - 5 + 115 = 180

7x + 110 = 180

7x = 70

x = 10

The measure of angle ∠F :

= (7x - 5)°

= (7 ⋅ 10 - 5)°

= (70 - 5)°

= 65°

In a parallelogram, opposite angles are equal.

∠D = ∠F

(5y)° = 65°

5y = 65

y = 13

Hence, the value of x is 10 and y is 13.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.