PROPERTIES OF EQUALITY
Addition Property of Equality
Words :
You can add the same number to both sides of an equation, and the statement will still be true.
Numbers :
5 = 5
5 + 3 = 5 + 3
8 = 8
Algebra :
x = y
x + z = y + z
Subtraction Property of Equality
Words :
You can subtract the same number from both sides of an equation, and the statement will still be true.
Numbers :
9 = 9
9 - 2 = 9 - 2
7 = 7
Algebra :
x = y
x - z = y - z
Multiplication Property of Equality
Words :
You can multiply both sides of an equation by the same non-zero number, and the statement will still be true.
Numbers :
4 = 4
4(7) = 4(7)
28 = 28
Algebra :
x = y
x(z) = y(z)
xz = yz
Division Property of Equality
Words :
You can divide both sides of an equation by the same non-zero number, and the statement will still be true.
Numbers :
4 = 4
4 ÷ 2 = 4 ÷ 2
2 = 2
Algebra :
x = y
x ÷ z = y ÷ z
ˣ⁄z = ʸ⁄z
Reciprocal Property of Equality
Words :
You can take reciprocal on both sides of an equation, and the statement will still be true.
Numbers :
4 = 4
¼ = ¼
Algebra :
x = y
¹⁄ₓ = ¹⁄y
Some oTher Properties of Inequality
Reflexive Property :
For any real number a,
a = a
Symmetric Property :
For any two real numbers a and b, if a = b, then b = a.
That is,
a = b -----> b = a
Transitive Property :
For any three real numbers a, b and c, if a = b and b = c, then a = c.
That is,
a = b, b = c -----> a = c
Substitution Property :
If a = b, then a can be substituted for b or b can be substituted for a in any equation or expression.
Distributive Property of Multiplication over Addition :
For all real numbers a, b and c,
a(b + c) = a ⋅ b + a ⋅ c
Distributive Property of Multiplication over Subtraction :
For all real numbers a, b and c,
a(b - c) = a ⋅ b - a ⋅ c
Commutative Property of Addition :
For all real numbers a an b,
a + b = b + a
Commutative Property of Multiplication :
For all real numbers a an b,
a ⋅ b = b ⋅ a
Associative Property of Addition :
For all real numbers a, b and c,
a + (b + c) = (a + b) + c
Associative Property of Multiplication :
For all real numbers a, b and c,
a ⋅ (b ⋅ c) = (a ⋅ b) ⋅ c
Note :
Commutative and Associative Properties are not true for subtraction and division.
Solving Equations
Property of equality along with other properties from algebra, such as the distributive property,
a(b + c) = ab + ac
can be used to solve equations.
For instance, let us solve the equation given below.
3(x + 2) = 2x + 8
Apply Distributive property on the left side of the equation.
3x + 6 = 2x + 8
Subtraction property :
Subtract 2x from each side of the equation.
x + 6 = 8
Subtract 6 from each side of the equation.
x = 2
Solving Problems
Problem 1 :
Solve 7x - 2 = 4x + 9 and write a reason for each step.
Solution :
Given :
7x - 2 = 4x + 13
Subtraction Property of Equality :
Subtract 4x from each side.
3x - 2 = 13
Addition Property of Equality :
Add 2 to each side.
3x = 15
Division Property of Equality :
Divide both sides by 2.
x = 5
Problem 2 :
Solve 52y - 3(12 + 9y) = 64 and write a reason for each step.
Solution :
Given :
52y - 3(12 + 9y) = 64
Distributive Property :
Distribute 3 to 12 and 9y.
52y - 36 - 27y = 64
Simplify :
25y - 36 = 64
Addition Property of Equality :
Add 36 to each side.
25y = 100
Division Property of Equality :
Divide both sides by 25.
y = 4
Problem 3 :
When we do exercise every day, we should find our target heart rate. This is the rate at which we achieve an effective workout while not placing too much strain on our heart. Our target heart rate r (in beats per minute) can be determined from our age a (in years) using the equation a = 220 - 10r/7.
(i) Solve the formula for r and write a reason for each step.
(ii) Use the result to find the target heart rate for a 16 year old.
(iii) Find the target heart rate for the following ages :
20, 30, 40, 50 and 60
What happens to the target heart rate as a person gets older ?
Solution (i) :
Given :
a = 220 - 10r/7
Addition Property of Equality :
Add 10r/7 to each side.
a + 10r/7 = 220
Subtraction Property of Equality :
Subtract a from each side.
10r/7 = 220 - a
Multiplication Property of Equality :
Multiply both sides by 7/10.
r = 7/10 ⋅ (220 - a)
Solution (ii) :
To find the target heart rate for a 16 year old, substitute a = 16.
r = 7/10 ⋅ (220 - 16)
Simplify :
r = 7/10 ⋅ 204
r = 1428 / 10
r = 142.8
The target hear rate for a 16 year old is about 142.8 beats per minute.
Solution (iii) :
The table given below shows the heart rate for ages 20, 30, 40, 50 and 60.
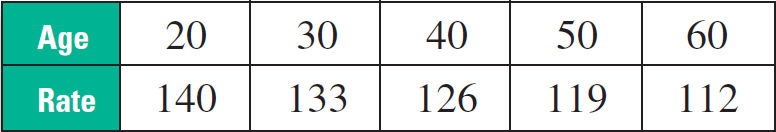
From the above table, it is clear that the target heart rate appears to decrease as a person gets older
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
10 Hard SAT Math Questions (Part - 14)
Sep 17, 25 09:38 AM
10 Hard SAT Math Questions (Part - 14) -
10 Hard SAT Math Questions (Part - 13)
Sep 14, 25 09:32 PM
10 Hard SAT Math Questions (Part - 13) -
10 Hard SAT Math Questions (Part - 12)
Sep 12, 25 09:50 PM
10 Hard SAT Math Questions (Part - 12)

