PROPERTIES OF DIVISION OF RATIONAL NUMBERS

Students who practice problems on dividing rational numbers must be aware of the properties of dividing rational numbers.

There are some properties of division of rational numbers like closure, commutative and associative. 

Closure Property

The collection of non-zero rational numbers is closed under division.

If a/b and c/d are two rational numbers, such that c/d ≠ 0,

then a/b ÷ c/d is always a rational number. 

Example :

2/3 ÷ 1/3  =  2/3 x 3/1  =  2 is a rational number.

Commutative Property

Division of rational numbers is not commutative.

If a/b and c/d are two rational numbers, 

then a/b ÷ c/d  ≠  c/d ÷ a/b

Example :

2/3 ÷ 1/3  =  2/3 x 3/1  =  2

1/3 ÷ 2/3  =  1/3 x 3/2  =  1/2

Hence, 2/3 ÷ 1/3  ≠  1/3 ÷ 2/3

Therefore, Commutative property is not true for division.

Associative Property

Division of rational numbers is not associative.

If a/b, c/d and e/f  are any three rational numbers,

then a/b ÷ (c/d ÷ e/f)  ≠  (a/b ÷ c/d) ÷ e/f

Example :

2/9 ÷ (4/9 ÷ 1/9)  =  2/9 ÷ 4  =  1/18 

(2/9 ÷ 4/9) ÷ 1/9  =  1/2 - 1/9  =  7/18

Hence, 2/9 ÷ (4/9 ÷ 1/9)  ≠  (2/9 ÷ 4/9) ÷ 1/9

Therefore, Associative property is not true for division.

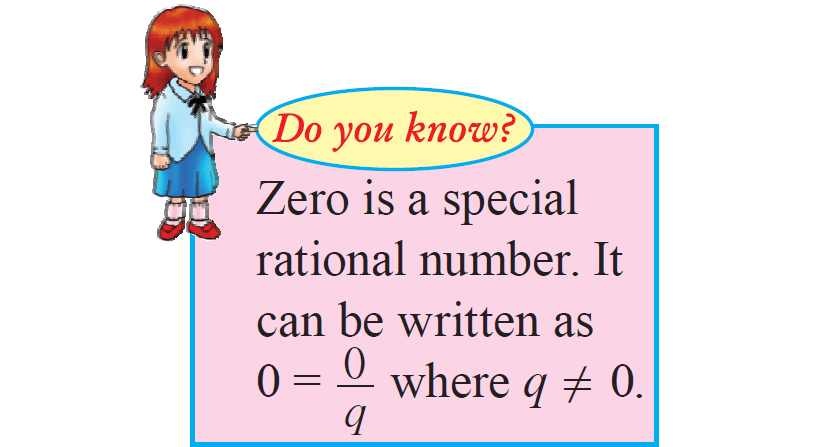
Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.