PROBLEMS BASED ON CIRCLES EXAMPLES

Question 1 :

Th e diameter of the circle is 52 cm and the length of one of its chord is 20 cm. Find the distance of the chord from the centre.

Solution :

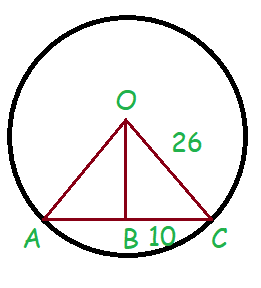
Diameter  =  52 cm

Radius of circle  =  26 cm

In triangle BOC,

OC²  =  OB² + BC²

26²  =  OB² + 10²

676 - 100  =  OB²

OB  =  √576

OB  =  24

Question 2 :

The chord of length 30 cm is drawn at the distance of 8 cm from the centre of the circle. Find the radius of the circle

Solution : 

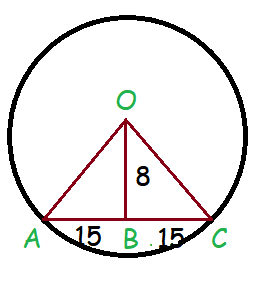
In triangle BOC,

OC²  =  OB² + BC²

OC²  =  8² + 15²

OC²  =  64 +  225

OC  =  √289

OC  =  17

Question 3 :

Find the length of the chord AC where AB and CD are the two diameters perpendicular to each other of a circle with radius 4√2 cm and also find <OAC and <OCA.

Solution :

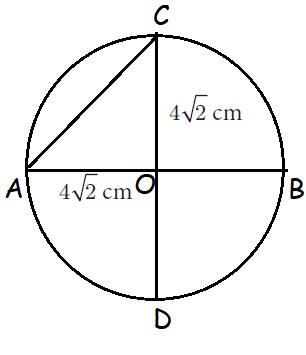
AC²  =  AO² + CO²

AC²  =  (4√2)² + (4√2)²

AC²  =  16(2) + 16(2)

AC²  =  64

AC  =  √64

AC  =  8 cm 

In triangle AOC,

<COA  =  90

OC  =  OA  

Equal sides will form a equal angles.

<OCA  =  <OBC

Question 4 :

A chord is 12 cm away from the centre of the circle of radius 15 cm. Find the length of the chord.

Solution : 

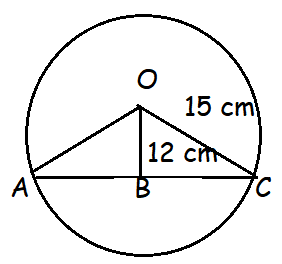
Let BC  =  x 

OC²  =  OB² + BC²

15²  =  12² + x²

225  -  144  =  x²

x  =  √81

x  =  9 cm 

AC  =  2BC  =  2(9)  =  18 cm

Question 5 :

In a circle, AB and CD are two parallel chords with centre O and radius 10 cm such that AB = 16 cm and CD = 12 cm determine the distance between the two chords?

Solution :

In triangle OPB,

OB²  =  OP² + PB²

OA  =  OB  =  OC  =  OD  =  10 (Radius)

10²  =  OP² + 8²

OP²  =  100 - 64

OP²  =  36

OP  =  √36

OP  =  6 cm

In triangle OQD,

OD²  =  OQ² + QD²

10²  =  OQ² + 6²

OQ²  =  100 - 36

OQ²  =  64

OQ  =  √64

OQ  =  8 cm

Distance between two chords  PQ  =  OQ - OP

  =  8 - 6

=  2 cm

Question 6 :

Two circles of radii 5 cm and 3 cm intersect at two points and the distance between their centres is 4 cm. Find the length of the common chord.

Solution :

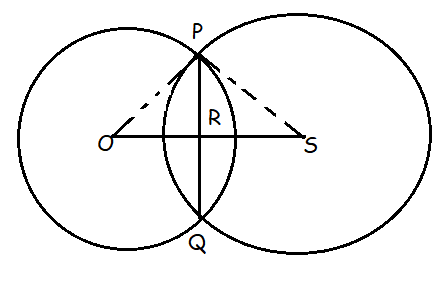
OP  =  3 cm, PS  =  5 cm and OS  =  4 cm

Let RS be x

OR  =  4 - x

In triangle POR,

OP²  =  OR² + PR²

3²  =  x² + PR²

PR²  =  9 - x²   ------(1)

In triangle PRS,

PS²  =  PR² + RS²

5²  =  PR² + (4 - x)²

PR²  =  25 - (4 - x)²

PR²  =  25 - (16 - 8x + x²)

PR²  =  25 - 16 + 8x - x²

PR²  =  9 + 8x - x²  ----(2)

(1)  =  (2)

9 - x²  =  9 + 8x - x²  

8x  =  0

x  =  0

By applying the value of x in (1), we get

PR²  =  9 - 0

PR²  =  9  ==>  PR  =  3

PQ  =  2PR

PQ  =  2(3)

PQ  =  6 cm

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.