PERPENDICULAR LINES IN THE COORDINATE PLANE

Perpendicular lines intersect at 90° or right angle.

In the coordinate plane, they would look like as shown below.

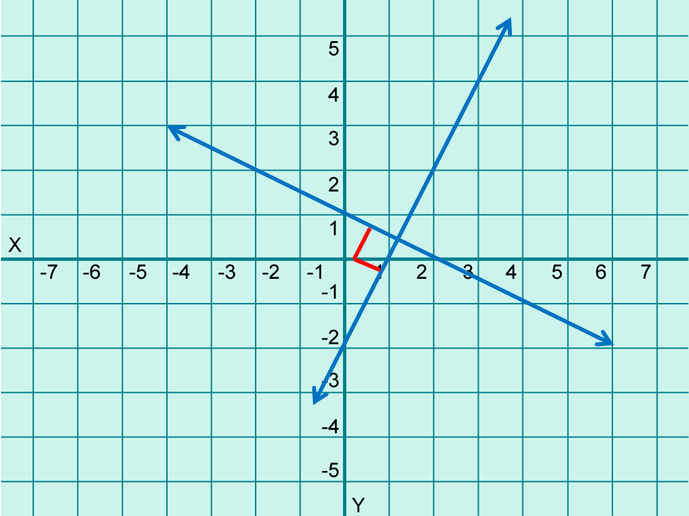
If we take a closer look at these two lines, we see that the slope of one is 2 and the other is -1/2.

This can be generalized to any pair of perpendicular lines in the coordinate plane. The slopes of perpendicular lines are opposite signs and reciprocals of each other.

or

The product of slopes of any two perpendicular lines is always equal to -1.

In the above example, we have

(-1/2) x 2 = -1

Postulate (Slopes of Perpendicular Lines) :

In a coordinate plane, two lines are perpendicular if and only if the product of their slopes is -1.

Slope of a Line in Coordinate Plane

The slope of a non vertical line is the ratio of the vertical change (the rise) to the horizontal change (the run).

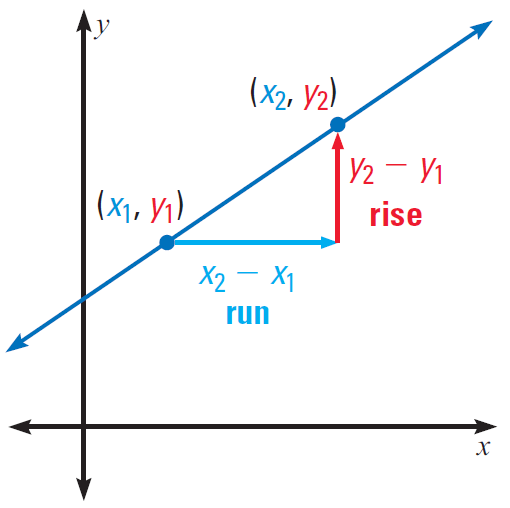
If the line passes through the points (x₁, y₁) and (x₂, y₂), then the slope is given by

Slope = rise/run

Slope = (y₂ - y₁)/(x₂ - x₁)

Usually, slope is represented by the variable m.

Example 1 :

In the diagram given below, find the slope of each line. Determine whether the lines j₁ and j₂ are perpendicular.

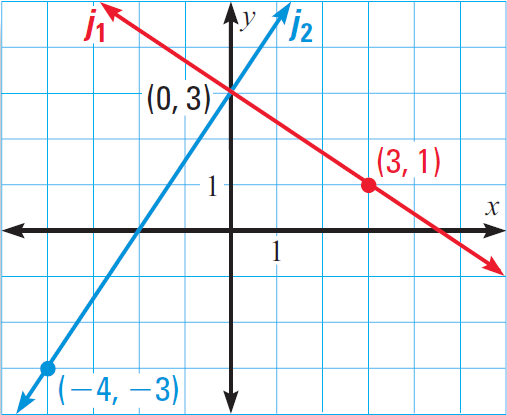
Solution :

Part 1 :

Find the slope of the line j₁. Line j₁ is passing through the points (0, 3) and (3, 1).

Let (x₁, y₁) = (0, 3) and (x₂, y₂) = (3, 1).

Slope (j₁) = (y₂ - y₁)/(x₂ - x₁)

Slope (j₁) = (1 - 3)/(3 - 0)

Slope (j₁) = -2/3

Part 2 :

Find the slope of the line j₂. Line j₂ is passing through the points (0, 3) and (-4, -3).

Let (x₁, y₁) = (0, 3) and (x₂, y₂) = (-4, -3).

Slope (j₂) = (-3 - 3)/(-4 - 0)

Slope (j₂) = -6/(-4)

Slope (j₂) = 3/2

Multiply the slopes :

The product is

= (-2/3) x (3/2) 

= -1

Since the product of slopes of the lines j_{1 and} j₂ is -1, the lines  j_1 and j₂ are perpendicular.

Example 2 :

In the diagram given below, find the slope of each line. Determine whether the lines are perpendicular.

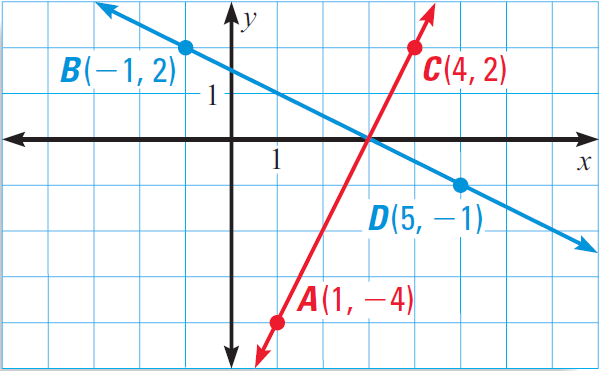
Solution :

Part 1 :

Find the slope of the line AC. Line AC is passing through the points (1, -4) and (4, 2).

Let (x₁, y₁) = (1, -4) and (x₂, y₂) = (4, 2).

Slope of AC = (y₂ - y₁)/(x₂ - x₁)

= [(2 - (-4)]/(4 - 1)

= (2 + 4)/3

= 6/3

= 2

Part 2 :

Find the slope of the line BD. Line BD is passing through the points (-1, 2) and (5, -1).

Let (x₁, y₁) = (-1, 2) and (x₂, y₂) = (5, -1).

Slope of BD = (-1 - 2)/[(5 - (-1)]

= -3/6

= -1/2

Multiply the slopes :

The product is

= 2 x (-1/2) 

= -1

Since the product of slopes of the lines is -1, the lines  AC and BD are perpendicular.

Example 3 :

Decide whether the lines are perpendicular.

Line 1 : y = 3x/4 + 2

Line 2 : y = -4x/3 - 3

Solution :

When we compare the given equations to slope intercept equation of a line y = mx + b, we get

slope of line 1 = 3/4

slope of line 2 = -4/3

Multiply the slopes :

The product is

= (3/4) x (-4/3) 

= -1

Since the product of slopes of the lines is -1, the given lines are perpendicular.

Example 4 :

Decide whether the lines are perpendicular.

Line 1 : 4x + 5y = 2

Line 2 : 5x + 4y = 3

Solution :

Rewrite each equation in slope-intercept form to find the slope.

Multiply the slopes :

The product is

= (-4/5) x (-5/4)

= 1

Since the product of slopes of the lines is not -1, the given lines  are not perpendicular.

Example 5 :

In the diagram given below, the equation y = 3x/2 + 3 represents a mirror. A ray of light hits the mirror at (-2, 0). What is the equation of the line p that is perpendicular to the mirror at this point?

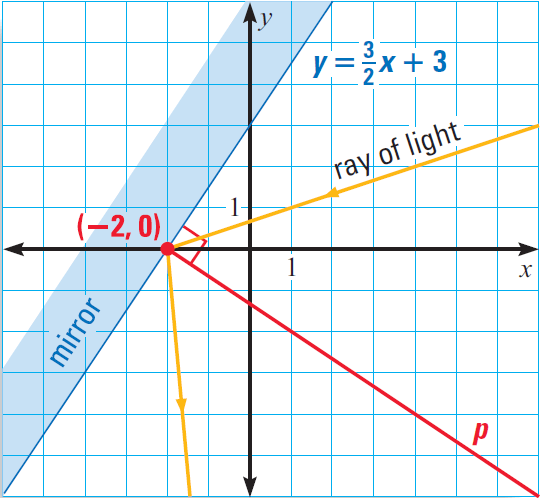
Solution :

The slope of the mirror is 3/2. So, the slope of the line p is -2/3.

Let y = mx + b be the equation of the line p.

Substitute (x, y) = (-2, 0) and m = -2/3 to find the value of b.

0 = (-2/3)(-2) + b

0 = 4/3 + b

Subtract 4/3 from both sides.

-4/3 = b

So, the equation of the line p is

y = -2x/3 - 4/3

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.