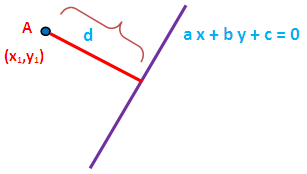
PERPENDICULAR DISTANCE

The perpendicular distance from the point (x₁, y₁) to the line ax + by + c = 0 is

|(ax₁ + by₁ + c) / √[a² + b²]|

Example 1:

Find the length of the perpendicular from (2, -3) to the straight line  2x - y + 9 = 0

Solution :

The length of the perpendicular from the point (x₁, y₁) to the line ax + by + c = 0 is

|(ax₁ + by₁ + c) / √[a² + b²]|

Substitute. 

=  |[2(2) - (-3) + 9] / √[2² + (-1)²]|

=  |(4 + 3 + 9) / √(4 + 1)|

=  |16/√5|

=  16/√5 units

Example 2 :

Find the length of the perpendicular from (5,2) to the straight line 3x + 2y - 1 = 0

Solution :

The length of the perpendicular from the point (x₁, y₁) to the line ax + by + c = 0 is

|(ax₁ + by₁ + c) / √[a² + b²]|

Substitute.

 =  |[3(5) + 2(2) - 1] / √[3² + 2²]|

=  |[15 + 4 - 1] / √[9 + 4]|

=  |18/√13|

=  18/√13 units

Example 3 :

Find the length of the perpendicular from (0,1) to the straight line x - 3y + 2 = 0

Solution :

The length of the perpendicular from the point (x₁, y₁) to the line ax + by + c = 0 is

|(ax₁ + by₁ + c) / √[a² + b²]|

Substitute.

 =  |[1(0) - 3(1) + 2] / √[1² + (-3)²]|

=  |[0 - 3 + 2] / √[1 + 9]|

=  |-1/√10|

=  1/√10 units

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.