PERMUTATION WITH REPETITION PROBLEMS WITH SOLUTIONS

Question :

8 women and 6 men are standing in a line.

(i) How many arrangements are possible if any individual can stand in any position?

(ii) In how many arrangements will all 6 men be standing next to one another?

(iii) In how many arrangements will no two men be standing next to one another?

Solution :

(i) How many arrangements are possible if any individual can stand in any position ?

Total number of members  =   14

The first person can choose any one of 14 places. So he or she has 14 options.

The 2nd person has 13 options. So each person will have 1 option less than the previous person has.

  =  14  ⋅ 13 ⋅ 12 ⋅ 11 ⋅ 10 ............. 1

So the total number of arrangements  =  14!

(ii) In how many arrangements will all 6 men be standing next to one another?

Solution :

Let us consider all 6 men as one unit. So, 8 women can choose their places out of 9 places (14 - 6). 

These 6 men can shuffle them in 6 places.

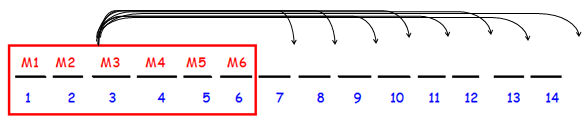
So, the number of arrangements for this case is 9! ⋅ 6!

(iii) In how many arrangements will no two men be standing next to one another?

Solution :

First, let us arrange the seats for women then, we may allot the seats for men between them.

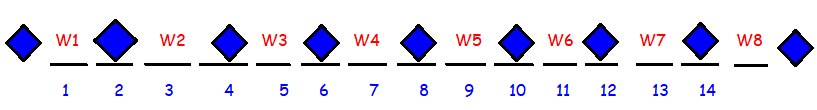
Women are having 8 seating options. They may shuffle them into 8!.

Next considering the number of seating arrangements for men, we have 9 seats in between them. Out of these 9 seats, they may choose any 6.

So, the total number of ways  =  8! ⋅ ⁹P₆

Question 2 :

Find the distinct permutations of the letters of the word MISSISSIPPI?

Solution :

Since we have repeating letters, we have to use the concept given below.

Total number of letters  =  11

In the word "MISSISSIPPI", the letter "S" is appearing 4 times. "I" is appearing 4 times, "P" is appearing 2 times.

  =  11!/4!4!2!

  =  11 ⋅ 10 ⋅ 9 ⋅ 8 ⋅ 7 ⋅ 6 ⋅ 5 ⋅ 4!/(4! 4! 2!)

  =  11 ⋅ 10 ⋅ 9 ⋅ 8 ⋅ 7 ⋅ 6 ⋅ 5 / (4 ⋅ 3 ⋅ 2 ⋅ 1)(2 ⋅ 1)

  =  34650

Hence the distinct permutations of the letters of the word is 34650.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.