PERIMETER OF SQUARE WORKSHEET

Problem 1 :

Find the perimeter of the square having side length 14 cm.

Problem 2 :

If the perimeter of square is 32 inches, then find the length of each side. 

Problem 3 :

The square has side length 250 cm. Find its perimeter in meter.

Problem 4 :

The length of each side of a square is 48 inches. Find its perimeter in feet. 

Problem 5 :

If the length of each diagonal of a square is 2√2 cm, then find its perimeter.

Problem 6 :

If the area of a square is 49 square inches, then find its perimeter. 

Problem 7 :

Find the perimeter of the figure which has the following vertices in xy-coordinate plane.

E(-1, 3), F(4, 3), G(4, -2) and H(-1, -2)

Problem 8 :

PQ is one of the sides of the square PQRS and the side PQ is defined by P(0, 2) and Q(6, 9). Find the perimeter of the square PQRS. 

Problem 9 :

AC is one of the diagonals of the square ABCD and the diagonal AC is defined by A(1, 4) and C(4, 8). Find the perimeter of the square ABCD. 

Problem 10 :

If the lengths of the sides of two squares are in the ratio 2 : 5. then find the ratio of their perimeters.

Solutions

Problem 1 :

Find the perimeter of the square having side length 14 cm.

Solution:

Formula for perimeter of a square :

=  4s 

Substitute 14 for s.

=  4(14)

=  56

So, the perimeter of the square is 56 cm.

Problem 2 :

If the perimeter of square is 32 inches, then find the length of each side. 

Solution:

Perimeter of the square  =  32 inches

4s  =  32

Divide each side by 4.

 s  =  8 

So, the length of each side of the square is 8 inches. 

Problem 3 :

The square has side length 250 cm. Find its perimeter in meter.

Solution:

Formula for perimeter of a square :

=  4s 

Substitute 250 for s.

=  4(250)

=  1000 cm -----(1)

We know  

100 cm  =  1 m

Therefore, to convert centimeter into meter,  we have to divide by 100. 

(1)-----> Perimeter  =  1000 cm

Divide the right side by 100 to convert cm into m.

Perimeter  =  (1000 / 100) m

=  10 m

So, the perimeter of the square is 10 meters.

Problem 4 :

The length of each side of a square is 48 inches. Find its perimeter in feet. 

Solution : 

Formula for perimeter of a square :

=  4s 

Substitute 48 for s.

=  4(48)

=  192 inches -----(1)

We know  

12 inches   =  1 ft

Therefore, to convert inches into feet,  we have to divide by 12. 

(1)-----> Perimeter  =  192 inches

Divide the right side by 12 to convert inches into ft.

Perimeter  =  (192 / 12) ft

=  16 ft

So, the perimeter of the square is 16 ft.

Problem 5 :

If the length of each diagonal of a square is 2√2 cm, then find its perimeter.

Solution:

To find the perimeter of a square, first we have to know the length of each side.

Let s be the length of each side of the square. 

Draw a sketch. 

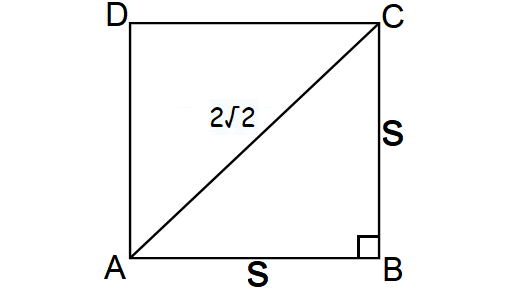
In the figure shown above, consider the right triangle ABC. 

By Pythagorean Theorem, we have

AB² + BC²  =  AC²

Substitute.

s² + s²  =  (2√2)²

Simplify and solve for s. 

2s²  =  2² ⋅(√2)²

2s²  =  4 ⋅(2)

2s²  =  8

Divide each side by 2. 

s²  =  4

Find positive square root on both sides.

 √s²  =  √4

 √s²  =  √(2 ⋅ 2)

s  =  2

Formula for perimeter of a square. 

Perimeter  =  4s

Substitute 2 for s. 

=  4(2)

=  8

So, the perimeter of the the square is 8 cm. 

Problem 6 :

If the area of a square is 49 square inches, then find its perimeter. 

Solution:

Area of the square  =  49 square inches

s²  =  49

Find positive square root on both sides.

 √s²  =  √49

 √s²  =  √(7 ⋅ 7)

s  =  7

Formula for perimeter of a square : 

=  4s

Substitute 7 for s. 

=  4(7)

=  28

So, the perimeter of the square is 28 inches.

Problem 7 :

Find the perimeter of the figure which has the following vertices in xy-coordinate plane.

E(-1, 3), F(4, 3), G(4, -2) and H(-1, -2)

Solution:

Draw a sketch with the given vertices.  

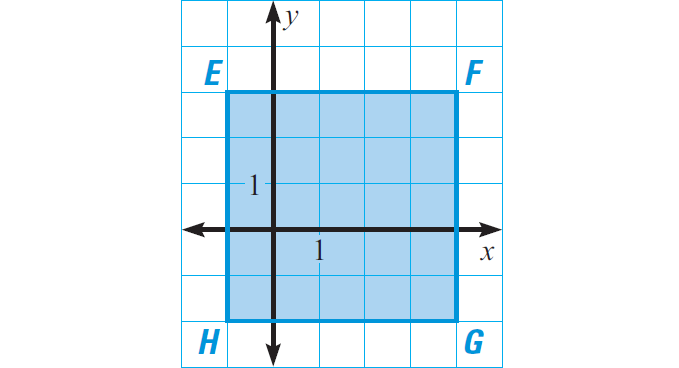
Clearly, the above figure is a square with side length of 5 units.

Formula for perimeter of a square :

=  4s

Substitute 5 for s.

=  4(5)

=  20

So, the perimeter of the square is 20 units.

Problem 8 :

PQ is one of the sides of the square PQRS and the side PQ is defined by P(0, 2) and Q(6, 9). Find the perimeter of the square PQRS. 

Solution:

Distance between the two points (x₁, y₁) and (x₂, y₂) is 

=  √[(x₂-x₁)²+(y₂-y₁)²]

To find the distance between P and Q, substitute 

(x₁, y₁)  =  (0, 2)

(x₂, y₂)  =  (6, 9)

in the above formula.

Distance between P and Q : 

=  √[(6-0)² + (9-2)²]

=  √[6² + 7²]

=  √[36 + 49]

=  √85

Therefore, the length of one of the sides is √85 units.

Formula for perimeter of a square :

=  4s

Substitute s  =  √85. 

=  4√85

Use calculator and simplify.

≈  36.88

So, the perimeter of the square PQRS is about 36.88 units.  

Problem 9 :

AC is one of the diagonals of the square ABCD and the diagonal AC is defined by A(1, 4) and C(4, 8). Find the perimeter of the square ABCD. 

Solution : 

Distance between the two points (x₁, y₁) and (x₂, y₂) is 

=  √[(x₂-x₁)²+(y₂-y₁)²]

To find the distance between A and C, substitute 

(x₁, y₁)  =  (1, 4)

(x₂, y₂)  =  (4, 8)

in the above formula.

Distance between A and C : 

=  √[(4-1)² + (8-4)²]

=  √[3² + 4²]

=  √[9 + 16]

=  √25

=  5

Therefore, the length of the diagonal AC is 5 units.

To find the perimeter of a square, first we have to know the length of each side.

Let s be the length of each side of the square. 

Draw a sketch. 

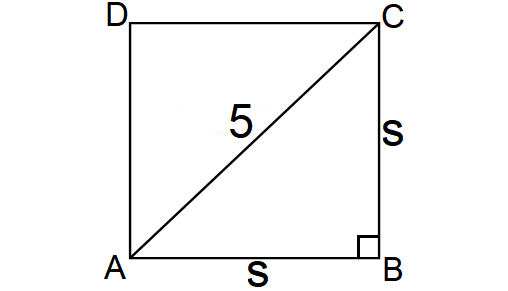
In the figure shown above, consider the right triangle ABC. 

By Pythagorean Theorem, we have

AB² + BC²  =  AC²

Substitute.

s² + s²  =  5²

Simplify and solve for s. 

2s²  =  25

Divide each side by 2. 

s²  =  12.5

Find positive square root on both sides.

 √s²  =  √12.5

 s  =  √12.5

Formula for perimeter of a square. 

Perimeter  =  4s

Substitute √12.5 for s. 

=  4√12.5

Use calculator and simplify.

≈  14.14

So, the perimeter of the the square is about 14.14 units.

Problem 10 :

If the lengths of the sides of two squares are in the ratio 2 : 5. then find the ratio of their perimeters.

Solution:

From the ratio 2 : 5, let the sides  of two squares be 2x and 5x respectively.

Formula for perimeter of a square :

=  4s

Ratio of the perimeters :

=  8x : 10x

Divide each term by 2x.

=  2 : 5

So, the ratio of the perimeters of two squares is 2 : 5.

Note :

The ratio of the sides of two squares and the ratio of the perimeters of two squares are same. 

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.