PAIR OF STRAIGHT LINES SOLVED PROBLEMS

Problem 1 :

The slope of one of the straight lines ax² + 2hxy + by² = 0 is twice that of the other, show that 8h² = 9ab.

Solution :

If the given pair of straight line is in the form ax² + 2hxy + by²  =  0, then their exists the following relationship.

m₁ + m₂  =  -2h/b  ------(1)

m₁ m₂  =  a/b  ------(2)

From the question given, we know that 

Slope of one straight line  =  2 (slope of other)

m₁  =  2 m₂

2m₂ + m₂  =  -2h/b

3 m₂  =  -2h/b

m₂  =  -2h/3b

Now we are going to apply m₁  =  2 m₂ and m₂  =  -2h/3b in the second equation.

2 (-2h/3b)(-2h/3b)  =  a/b 

8h²/9b²  =  a/b

8h²  =  9ab

Problem 2 :

The slope of one of the straight lines ax² + 2hxy + by² = 0 is three times the other, show that 3h² = 4ab.

Solution :

If the given pair of straight line is in the form ax² + 2hxy + by²  =  0, then their exists the following relationship.

m₁ + m₂  =  -2h/b  ------(1)

m₁ m₂  =  a/b  ------(2)

From the question given, we know that 

Slope of one straight line  =  3 (slope of other)

m₁  =  3 m₂

3m₂ + m₂  =  -2h/b

4 m₂  =  -2h/b

m₂  =  -2h/4b  =  -h/2b

Now we are going to apply m₁  =  3 m₂ and m₂  =  -h/2b in the second equation.

m₁ m₂  =  a/b

3 m₂m₂  =  a/b

3 (m₂)²  =  a/b

3(-h/2b)²  =  a/b

3h²/4b²  =  a/b

3h²  =  4ab

Problem 3 :

A ΔOPQ is formed by the pair of straight lines x² −4xy +y² = 0 and the line PQ. The equation of PQ is x + y − 2 = 0. Find the equation of the median of the triangle ΔOPQ drawn from the origin O.

Solution :

We cannot find the factors from the pair of straight lines. By solving the given equations, we may get the vertices P and Q.

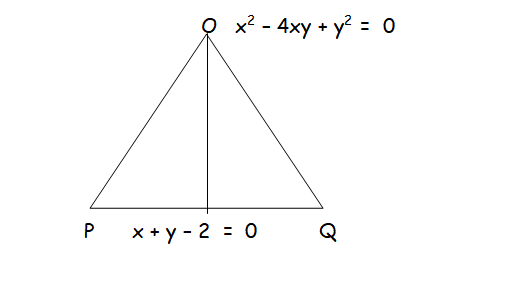
x² −4xy + y² = 0   --------(1)

x + y − 2 = 0   --------(2)

Apply y  =  -x + 2 in the first equation, we get

x² − 4x (-x + 2) + (-x + 2)² = 0

x² + 4x² - 8x + x² - 4x + 4  =  0

6x² - 12x + 4  =  0

3x² - 6x + 2  =  0

x  =  (-b ± √b² - 4ac) / 2a

x  =  [6 ± √6² - 4(3)(2)] / 2(3)

x  =  [6 ± √(36 - 24)] / 2(3)

x  =  [6 ± √12] / 6

x  =  [6 ± 2√3] / 6

x  =  1 ± (√3/3)

Midpoint of PQ :

  =  (x₁ + x₂)/2,  (y₁ + y₂)/2

=  2/2, 2/2

Midpoint of PQ is (1, 1)

Equation of median PQ :

O (0, 0) M (1, 1)

(y - 0)/(1 - 0)  =  (x - 0)/(1 - 0)

y/1  =  x/1

y  =  x

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.