OPERATIONS WITH FRACTIONS WORKSHEETS

Problem 1 : 

Simplify :

2/5 + 3/5

Problem 2 : 

Simplify :

7/5 - 3/5

Problem 3 : 

Simplify :

1/8 + 1/3

Problem 4 :

Simplify :

5/12 + 1/20

Problem 5 : 

Convert the fraction 17/5 into mixed number

Problem 6 : 

Multiply 2/3 and 4/5.

Problem 7 : 

Divide 6 by 2/5.

Problem 8 : 

Divide 1/5 by  3/7.

Problem 9 : 

Each part of a multi part question on a test is worth the same number of points. The whole question is worth 37.5 points. Daniel got 1/2 of the parts of a question correct. How many points did Daniel receive ?

Problem 10 :

The bill for a pizza was $14.50. Charles paid for 3/5 of the bill. How much did he pay ?

Solutions

Problem 1 : 

Simplify :

2/5 + 3/5

Solution : 

Here, for both the fractions, we have the same denominator, we have to take only one denominator and add the numerators. 

Then, we get 

2/5 + 3/5  =  (2+3) / 5

2/5 + 3/5  =  5/5

2/5 + 3/5  =  1

Problem 2 :

Simplify :

7/5 - 3/5

Solution : 

Here, for both the fractions, we have the same denominator, we have to take only one denominator and subtract the numerators. 

Then, we get 

7/5 - 3/5  =  (7-3) / 5

7/5 - 3/5  =  4/5

Problem 3 : 

Simplify :

1/8 + 1/3

Solution :

In the given two fractions, denominators are 8 and 3.

For 8 and 3, there is no common divisor other than 1.

So 8 and 3 are co-prime.

Here we have to apply cross-multiplication method to add the two fractions 1/8 and 1/3 as given below. 

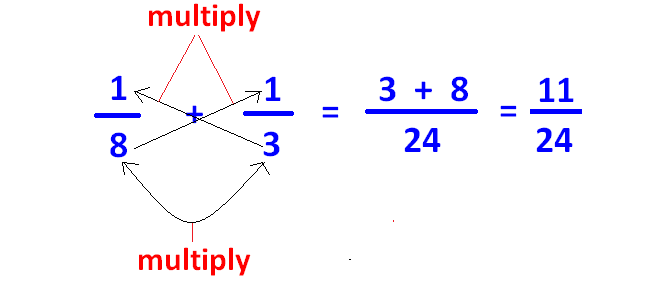
Hence, 

1/8 + 1/3  =  11/24

Problem 4 :

Simplify :

5/12 + 1/20

Solution :

In the given two fractions, denominators are 12 and 20.

For 12 and 20, if there is at least one common divisor other than 1, then 12 and 20 are not co-prime.

For 12 & 20, we have the following common divisors other than 1.

2 and 4

So 12 and 20 are not co-prime.

In the next step, we have to find the L.C.M (Least common multiple) of 12 and 20.

12  =  2² ⋅ 3

20  =  2² ⋅ 5

When we decompose 12 and 20 in to prime numbers, we find 2, 3 and 5 as prime factors for 12 and 20. 

To get L.C.M of 12 and 20, we have to take 2, 3 and 5 with maximum powers found above.

So, L.C.M of 12 and 20 is 

=  2² ⋅ 3 ⋅ 5

=  4 ⋅ 3 ⋅ 5

=  60

Now we have to make the denominators of both the fractions to be 60 and add the two fractions 5/12 and 1/20 as given below.

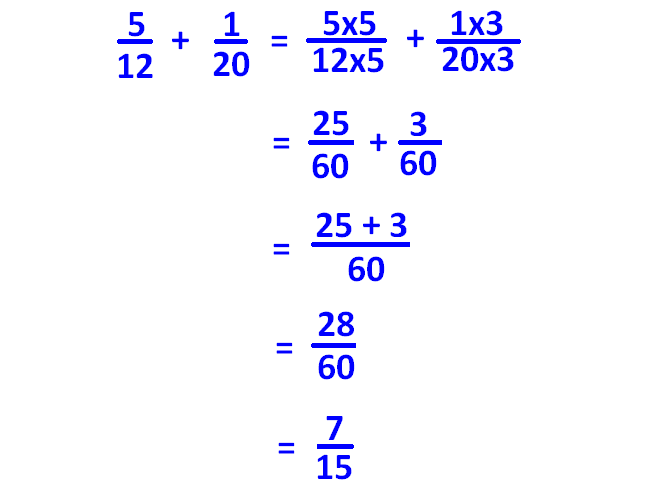
Hence,

5/12 + 1/20  =  7/15

Problem 5 :

Convert the fraction 17/5 into mixed number.

Solution :

The picture given below clearly illustrates, how to convert the fraction 17/5 into mixed number.

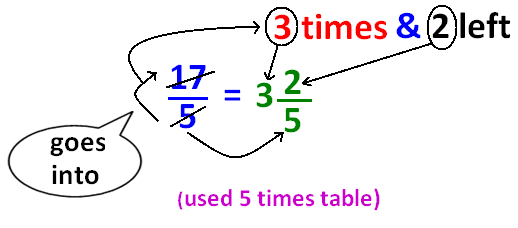
Hence,

17/5  =  3 2/5

Problem 6 :

Multiply 2/3 and 4/5.

Solution :

To multiply a proper or improper fraction by another proper or improper fraction, we have to multiply the numerators and denominators. 

That is, 

2/3 ⋅ 4/5   =  8/15

Problem 7 :

Divide 6 by 2/5.

Solution :

To divide a whole number by any fraction, multiply that whole number by the reciprocal of that fraction.

That is,  

6 ÷  2/5  =  6 ⋅ 5/2

6 ÷  2/5  =  (6 ⋅ 5)/2

6 ÷  2/5  =  30/2

6 ÷  2/5  =  15

Problem 8 :

Divide 1/5 by  3/7.

Solution :

To divide a fraction by another fraction, multiply the first fraction by the reciprocal of the second fraction.

That is, 

1/5 ÷  3/7  =  1/5 ⋅ 7/3

1/5 ÷  3/7  =  (1 ⋅ 7) / (5 ⋅ 3)

1/5 ÷  3/7  =  7/15

Problem 9 : 

Each part of a multipart question on a test is worth the same number of points. The whole question is worth 37.5 points. Daniel got 1/2 of the parts of a question correct. How many points did Daniel receive ?

Solution : 

To find the total points received by Daniel, we have to multiply 1/2 and 37.5.  

Step 1 :

Convert the decimal 3.75 as the fraction 75/2.

(1/2) ⋅ 37.5  =  (1/2) ⋅ (75/2) 

Step 2 :

Multiply. Write the product in simplest form.

(1/2) ⋅ (75/2)  =  (1 ⋅ 75) / (2 ⋅ 2)

(1/2) ⋅ (75/2)  =  75 / 4

(1/2) ⋅ (75/2)  =  18 3/4

Hence, Daniel received 18 3/4 points. 

Problem 10 : 

The bill for a pizza was $14.50. Charles paid for 3/5 of the bill. How much did he pay ?

Solution : 

To find the amount paid by Charles, we have to multiply 3/5 and 14.50  

Step 1 :

Convert the decimal 14.50 as the fraction 29/2

(3/5) ⋅ 14.50  =  (3/5) ⋅ (29/2) 

Step 2 :

Multiply. Write the product in simplest form.

(3/5) ⋅ (29/2)  =  (3 ⋅ 29) / (5 ⋅ 2)

(3/5) ⋅ (29/2)  =  87 / 10

(3/5) ⋅ (29/2)  =  8 7/10

Hence, Charles paid $8 7/10. 

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.