MODELING TWO STEP EQUATIONS WITH ALGEBRA TILES

In this section, you will learn how to model two step equations using algebra tiles. 

Here, we can use the following algebra tiles to model two-step equations.

Example 1 : 

Use algebra tiles to model 3x - 4  =  5.

Solution : 

Step 1 :

How can we model the left side of the equation ?

We find 3x - 4 on the left side of the equation. So we can use three positive variable tiles and four -1-tiles to model the left side of the equation.

Step 2 :

How can we model the right side of the equation ?

We find positive 5 on the right side of the equation. So we can use five +1 tiles to model the right side of the equation.

Step 3 : 

Now use the above mentioned algebra tiles or draw them to model the equation on the mat.

Example 2 : 

Use algebra tiles to model 2x + 1  =  3.

Solution : 

Step 1 :

How can we model the left side of the equation ?

We find 2x + 1 on the left side of the equation. So we can use two positive variable tiles and one +1-tiles to model the left side of the equation.

Step 2 :

How can we model the right side of the equation ?

We find positive 3 on the right side of the equation. So we can use three +1 tiles to model the right side of the equation.

Step 3 : 

Now use the above mentioned algebra tiles or draw them to model the equation on the mat.

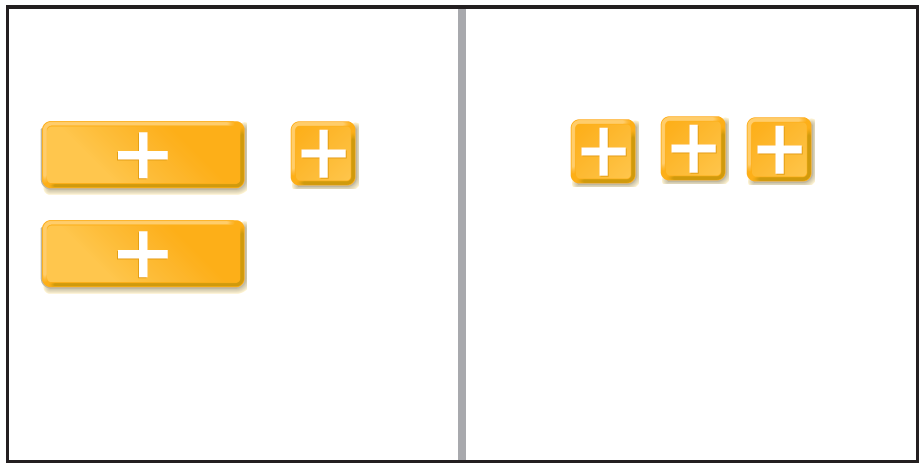
Example 3 : 

Use algebra tiles to model 4 - 3x  =  5.

Solution : 

Step 1 :

How can we model the left side of the equation ?

We find 4 - 3x on the left side of the equation. So we can use  four +1-tiles and three negative variable tiles to model the left side of the equation.

Step 2 :

How can we model the right side of the equation ?

We find positive 5 on the right side of the equation. So we can use five +1 tiles to model the right side of the equation.

Step 3 : 

Now use the above mentioned algebra tiles or draw them to model the equation on the mat.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.