DIRECT VARIATION AND INVERSE VARIATION
Direct Variation
Please look at the following situations.
You buy more pens ---> Costs you more
More no. of students ---> More no. of teachers
Travel less distance ---> Time taken is less
No. of books reduced ---> Weight of bag is less
Thus we can say, if an increase in one quantity produces a proportionate increase in another quantity, then the quantities are said to be in direct variation.
or
If a decrease in one quantity produces a proportionate decrease in another quantity, then the quantities are said to be in direct variation.
Change in both the quantities must be same.
That is,
Increase ----> Increase
or
Decrease ----> Decrease
Inverse Variation
Please look at the following situations.
More men ----> Less days to complete a work
More speed ----> Less time to cover the distance
More vehicles on the road ----> Less road space
Less time per day ----> More days to complete the work
Thus we can say, if an increase in one quantity produces a proportionate decrease in another quantity, then the quantities are said to be in inverse variation.
or
If a decrease in one quantity produces a proportionate increase in another quantity, then the quantities are said to be in inverse variation.
Changes in the quantities must be opposite.
That is,
Increase -----> Decrease
or
Decrease -----> Increase
Shortcuts

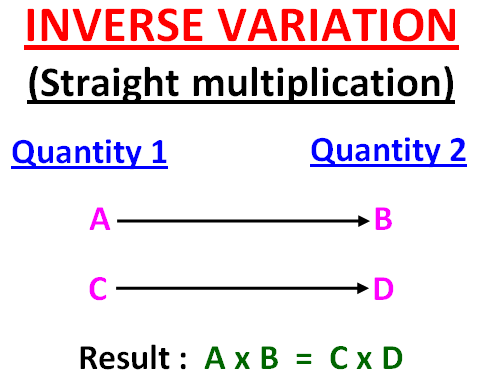
Solved Problems
Problem 1 :
75 basketballs cost $1143.75. Find the cost of 26 basketballs.
Solution :
This is a situation of direct variation.
Because,
less number of basket balls ----> cost will be less
Let m be the cost of 26 basket balls.
|
No. of Basket Balls 75 26 |
Cost 1143.75 m |
Since this is direct variation, we have to apply the shortcut cross multiplication.
75m = 26(1143.75)
m = (29737.5)/75
m = $396.50
Problem 2 :
7 men can complete a work in 52 days. In how many days will 13 men finish the same work?
Solution :
This is a situation of inverse variation.
Because,
more men ----> less days
Let m be the required no. of days.
|
No. of Men 7 13 |
No. of Days 52 m |
Since this is inverse variation, we have to apply the shortcut straight multiplication.
7(52) = 13m
364/13 = m
m = 28 days
Problem 3 :
A book contains 120 pages and each page has 35 lines. How many pages will the book contain if every page has 24 lines per page ?
Solution :
This is a situation of inverse variation.
Because,
less lines ----> more pages
Let m be the required number of pages.
|
No. of Pages 120 m |
No. of Lines 35 24 |
Since this is inverse variation, we have to apply the shortcut straight multiplication.
120(35) = m(24)
(4200)/24 = m
m = 175 pages.
Problem 4 :
A truck covers a particular distance in 3 hours with the speed of 60 miles per hour. If the speed is increased by 30 miles per hour, find the time taken by the truck to cover the same distance
Solution :
This is a situation of inverse variation.
Because,
more speed ----> less time
If the given speed 60 mph is increased by 30 mph,
then the new speed = 90 mph
Let m be the required time.
|
No. of Hours 3 m |
Speed 60 90 |
Since this is inverse variation, we have to apply the shortcut straight multiplication.
3(60) = m(90)
180/90 = m
m = 2 hrs
Problem 5 :
In a business, if A can earn $7500 in 2.5 years, At the same rate, find his earning for 4 years.
Solution :
This is a situation of direct variation.
Because,
more time ----> more earning
|
No. of Years 2.5 4 |
Earning 7500 m |
Since this is direct variation, we have to apply the shortcut cross multiplication
2.5m = 4(7500)
m = (30000)/2.5
m = $12000
So, the earning for 4 years is $12000.
Problem 6 :
In 36.5 weeks, Miguel raised $2,372.50 for cancer research. How much money will he raise 20 weeks ?
Solution :
This is a situation of direct variation.
Because,
less number of weeks ----> amount raised will be less
Let m be the required amount of money.
|
Weeks 36.5 20 |
Amount of Money 2372.50 m |
Since this is direct variation, we have to apply the shortcut cross multiplication.
36.5m = 20(2372.50)
m = (47450)/36.5
m = $1300
Problem 7 :
Alex takes 15 days to reduce 30 kilograms of his weight by doing 30 minutes exercise per day. If he does exercise for 1 hour 30 minutes per day, how many days will he take to reduce the same weight ?
Solution :
This is a situation of inverse variation.
Because,
more minutes per day----> less days to reduce the weight
And also
1 hour 30 minutes per day = 90 minutes per day
Let m be the required number of days.
|
No. of Days 15 m |
No. of Minutes 30 90 |
Since this is inverse variation, we have to apply the shortcut straight multiplication
15(30) = m(90)
450/90 = m
m = 5 days
Problem 8 :
If 5 men can paint a house in 18 hours, how many men will be able to paint it in 10 hours ?
Solution :
This is a situation of inverse variation.
Because,
less hours ----> more men
Let m be the required number of men.
|
No. of Men 5 m |
No. of Hours 18 10 |
Since this is inverse variation, we have to apply the shortcut straight multiplication.
5(18) = m(10)
90/10 = m
m = 9 men
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Time and Work Problems
Oct 20, 25 07:13 AM
Time and Work Problems - Concept - Solved Problems -
10 Hard SAT Math Questions (Part - 30)
Oct 17, 25 07:27 PM
10 Hard SAT Math Questions (Part - 30) -
10 Hard SAT Math Questions (Part - 29)
Oct 16, 25 06:04 AM
10 Hard SAT Math Questions (Part - 29)
