HOW TO FIND ASYMPTOTES OF A RATIONAL FUNCTION

Find the asymptotes of the following curves :

Problem 1 :

f(x)  =  x²/(x² - 1)

Solution :

Horizontal asymptote :

Horizontal asymptote is  =  1/1

y  =  1

Vertical asymptote :

x²-1  =  0

x²  =  1

x  =  ±1

There is no slant asymptote.

Problem 2 : 

x²/(x + 1)

Solution :

Horizontal asymptote :

Degree of numerator > degree of denominator

So, there is no horizontal asymptote.

Vertical asymptote :

x + 1  =  0

x  =  -1

Slant asymptote :

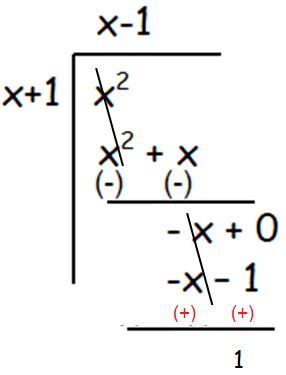
So, the slant asymptote is y  =  x - 1.

Problem 3 :

f(x)  =  3x/√(x² + 2)

Solution :

Horizontal asymptote :

Degree of numerator = degree of denominator

Horizontal asymptotes are  =  3/1 and 3/-1

So, horizontal asymptotes are 3 and -3

Vertical asymptote :

√(x²+2)  =  0

x²  =  -2

No real values for x.

There is no slant asymptotes.

Problem 4 :

f(x)  =   (x² - 6x - 1)/(x + 3)

Solution :

Since degree of numerator is greater than the denominator, there is no horizontal asymptote.

x + 3  =  0.

x  =  -3

So, vertical asymptote is x  =  -3.

Slant asymptote :

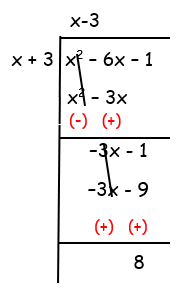
Slant asymptote is y  =  x - 3.

Problem 5 :

f(x)  =  x² + 6x - 4/(3x - 6)

Solution :

Since the numerator is having highest exponent, there is no horizontal asymptote. 

3x-6  =  0

x  =  2

So, vertical asymptote is x  =  2

Slant asymptote :

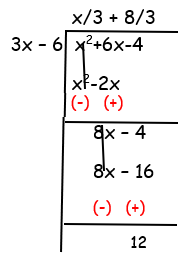
Slant asymptote is y  =  x/3 + 8/3.

Problem 6 :

Find the horizontal and vertical asymptotes of the rational function

f(x) = (x + 11) / (x² - 25x)

Solution :

f(x) = (x + 11) / (x² - 25x)

Horizontal asymptote :

f(x) = (x + 11) / x(x - 25)

The highest exponent of the denominator is greater than the highest exponent of the numerator. Then it has horizontal asymptote as y = 0.

Vertical asymptote :

To find the vertical asymptote, we have to equate the denominator to 0.

x(x - 25) = 0

x = 0 and x = 25

Problem 7 :

Find the horizontal and vertical asymptotes of the rational function

f(x) = -3x² / (x² + 7x - 44)

Solution :

f(x) = -3x² / (x² + 7x - 44)

Horizontal asymptote :

Since both numerator and denominator has same power the horizontal asymptote will be

y = leading coefficient of numerator / leading coefficient of denominator

y = -3/1

y = -3

Vertical asymptote :

x² + 7x - 44 = 0

x² + 11x - 4x - 44 = 0

x(x + 11) - 4(x + 11) = 0

(x + 11)(x - 4) = 0

x = -11 and x = 4

So, vertical asymptotes are x = -11 and x = 4.

Problem 8 :

Give the equation of the oblique asymptote, if any, of the function.

f(x) = (x² + 8x + 9)/(x + 2)

Solution :

f(x) = (x² + 8x + 9)/(x + 2)

Since the highest exponent of the numerator is greater than the highest exponent of the denominator, we will get only slant asymptote.

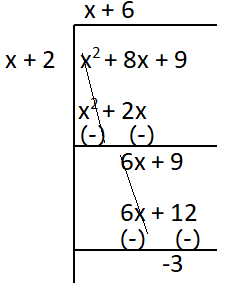
= (x + 6) - 3/(x + 2) 

Equation of slant asymptote is y = x + 6

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.