GRAPHING LINEAR FUNCTIONS WORKSHEET

1. Graph the linear function y = (2/3)x - 1.

2. Graph the linear function y = (-5/2)x + 3.

3. Ken has a weekly goal of burning 2400 calories by taking brisk walks. The equation y = -300x + 2400 represents the number of calories y Ken has left to burn after x hours of walking which burns 300 calories per hour. After how many hours of walking will Ken have 600 calories left to burn ? After how many hours will he reach his weekly goal?

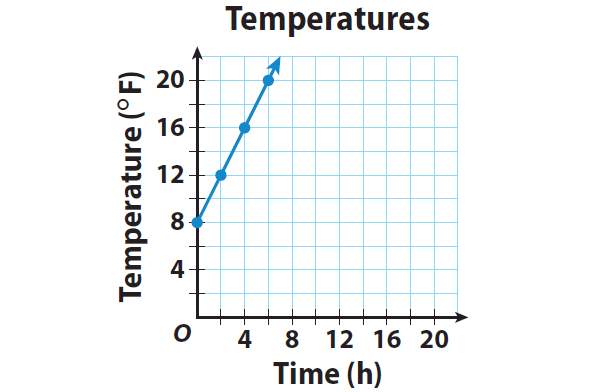
4. The temperature at dawn was 8 °F and increased steadily 2 °F every hour. The equation y = 2x + 8 gives the temperature y after x hours. State whether the relationship between the time and the temperature is proportional or non proportional. Then graph the function.

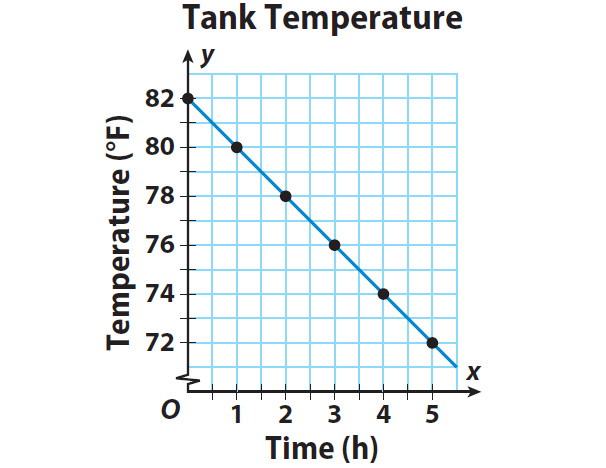
5. The temperature of a fish tank was 82 °F and decreased steadily 2 °F every hour. The equation y = -2x + 82 gives the temperature y after x hours. State whether the relationship between the time and the temperature is proportional or non proportional. Then graph the function.

1. Answer :

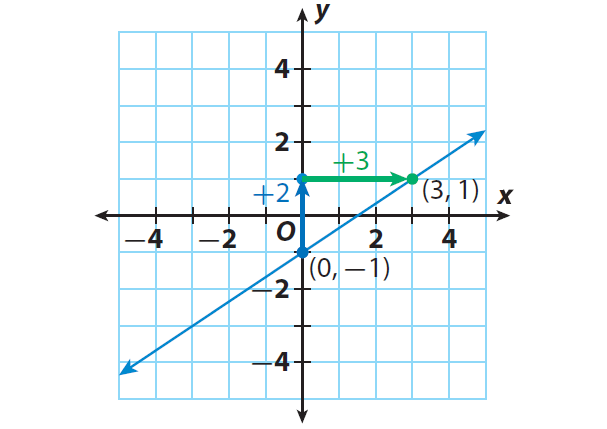
y = (2/3)x - 1

Step 1 :

The y-intercept is b = -1. Plot the point that contains the y-intercept : (0, -1).

Step 2 :

The slope is m = 2/3. Use the slope to find second a point. Since the slope = rise/run, from (0, -1), count up 2 units and right 3 units.

Then, the new point is (3, 1).

Step 3 :

Draw a line through the points.

2. Answer :

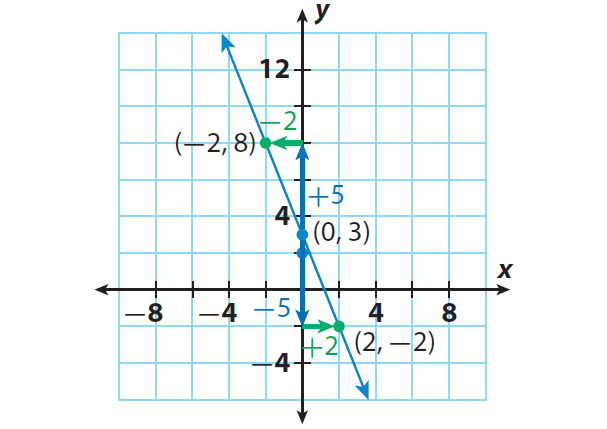
y = (-5/2)x + 3

Step 1 :

The y-intercept is b = 3. Plot the point that contains the y-intercept : (0, 3).

Step 2 :

The slope is m = -5/2. Use the slope to find second a point. Since the slope = rise/run, from (0, 3), count down 5 units and right 2 units or up 5 units and left 2 units.

Then, the new point is (2, -1) or (-2, 8).

Step 3 :

Draw a line through the points.

3. Answer :

Step 1 :

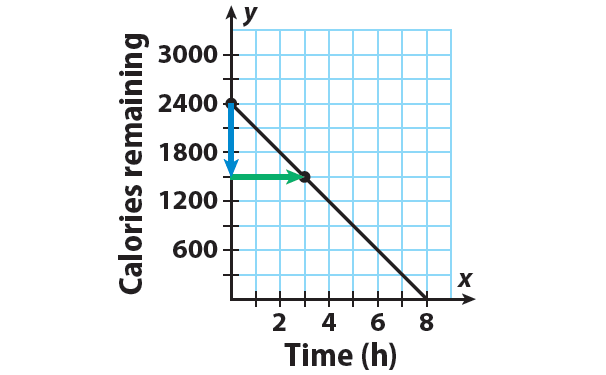
 y = -300x + 2400

The y-intercept is b = 2400. Plot the point that contains the y-intercept : (0, 2400).

Step 2 :

Write the slope as a fraction.

m = -300/1 = -600/2 = -900/3

Using the slope as -900/3 helps in drawing a more accurate graph.

The slope is m = -900/3. Use the slope to find second a point. Since the slope  =  rise / run, from (0, 2400), count down 900 units and right 3 units.

Then, the new point is (3, 1500).

Step 3 :

Draw a line through the points.

Step 4 :

To find after how many hours of walking will Ken have 600 calories left to burn.

Locate 600 calories on the y-axis. Read across and down to the x-axis.

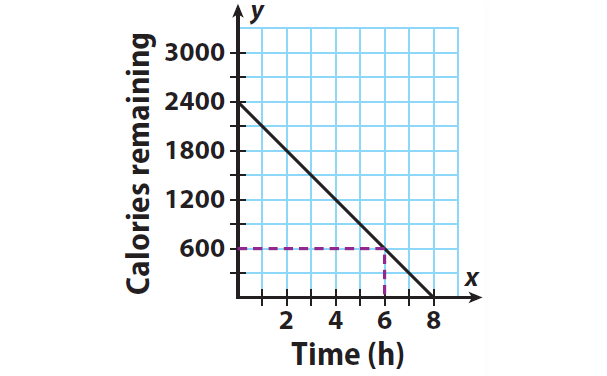
From the graph, we can know that Ken will have 600 calories left to burn after 6 hours.

Step 5 :

Ken will reach his weekly goal when the number of calories left to burn is 0. Because every point on the x-axis has a y-value of 0, find the point where the line crosses the x-axis.

Ken will reach his goal after 8 hours of brisk walking.

4. Answer :

Step 1 :

The given equation y = 2x + 8 is in slope-intercept form linear equation. That is, y = mx + b.

When we compare the equation y = 2x + 8 with y = mx + b, we get m = 2 and b = 8.

Therefore, the equation is a linear equation. Since b ≠ 0, the relationship is non proportional.

Step 2 :

Choose several values for the input x. Plug these values for x in the equation to find the output y.

Step 3 :

Graph the ordered pairs. Then draw a line through the points to represent the solutions of the function.

5. Answer :

Step 1 :

The given equation y = -2x + 82 is in slope-intercept form linear equation. That is, y = mx + b.

When we compare the equation y = -2x + 82 with y = mx + b, we get m = -2 and b = 82.

Therefore, the equation is a linear equation. Since b ≠ 0, the relationship is non proportional.

Step 2 :

Choose several values for the input x. Plug these values for x in the equation to find the output y.

Step 3 :

Graph the ordered pairs. Then draw a line through the points to represent the solutions of the function.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.

WORD PROBLEMS

HCF and LCM  word problems

Word problems on simple equations 

Word problems on linear equations 

Word problems on quadratic equations

Algebra word problems

Word problems on trains

Area and perimeter word problems

Word problems on direct variation and inverse variation 

Word problems on unit price

Word problems on unit rate 

Word problems on comparing rates

Converting customary units word problems 

Converting metric units word problems

Word problems on simple interest

Word problems on compound interest

Word problems on types of angles 

Complementary and supplementary angles word problems

Double facts word problems

Trigonometry word problems

Percentage word problems 

Profit and loss word problems 

Markup and markdown word problems 

Decimal word problems

Word problems on fractions

Word problems on mixed fractions

One step equation word problems

Linear inequalities word problems

Ratio and proportion word problems

Time and work word problems

Word problems on sets and Venn diagrams

Word problems on ages

Pythagorean theorem word problems

Percent of a number word problems

Word problems on constant speed

Word problems on average speed 

Word problems on sum of the angles of a triangle is 180 degree

OTHER TOPICS 

Profit and loss shortcuts

Percentage shortcuts

Times table shortcuts

Time, speed and distance shortcuts

Ratio and proportion shortcuts

Domain and range of rational functions

Domain and range of rational functions with holes

Graphing rational functions

Graphing rational functions with holes

Converting repeating decimals in to fractions

Decimal representation of rational numbers

Finding square root using long division

L.C.M method to solve time and work problems

Translating the word problems in to algebraic expressions

Remainder when 2 power 256 is divided by 17

Remainder when 17 power 23 is divided by 16

Sum of all three digit numbers divisible by 6

Sum of all three digit numbers divisible by 7

Sum of all three digit numbers divisible by 8

Sum of all three digit numbers formed using 1, 3, 4

Sum of all three four digit numbers formed with non zero digits

Sum of all three four digit numbers formed using 0, 1, 2, 3

Sum of all three four digit numbers formed using 1, 2, 5, 6