FINDING DISTANCE BETWEEN TWO POINTS EXAMPLES

Let (x₁, y₁) and (x₂, y₂) be the two points as shown below. 

Then, the formula for the distance between the two points is 

√[(x₂ - x₁)² + (y₂ - y₁)²]

Examples

Example 1 :

Check whether (5,-2) (6,4) and (7,-2) are the vertices of an isosceles triangle.

Solution :

Let the given points as A(5,-2)  B(6,4) and C(7,-2)

Distance between two points = √(x₂ - x₁)² + (y₂ - y₁)²

Length of the side AB :

Here, x₁  =  5, y₁  =  -2, x₂  =  6  and  y₂  =  4

  =  √(6 - 5)² + (4 - (-2))²

  =  √1² + (4+2)²

  =  √1 + 36

  =  √37

Length of the side BC :

Here, x₁  =  6, y₁  =  4, x₂  =  7  and  y₂  =  -2

  =  √(7 - 6)² + (-2 - 4)²

  =  √1² + (-6)²

  =  √1 + 36

  =  √37

Length of the side CA :

Here, x₁  =  7, y₁  =  -2, x₂  =  5  and  y₂  =  -2

  =  √(-5 - 7)² + (-2 - (-2))²

  =  √(-12)² + (-2 + 2)²

  =  √144 + 0

  =  √144

  =  12

AB  =  BC

Since length of two sides are equal, the given points are the vertices of a triangle.

Example 2 :

In a classroom 4 friends are seated at the points A,B,C and D as shown in figure given below. Champa and Chameli walk into the class and after observing for a few minutes champa asks Chameli,"Don't you think ABCD is a square?" Chameli disagrees. Using distance formula, find which of them is correct.

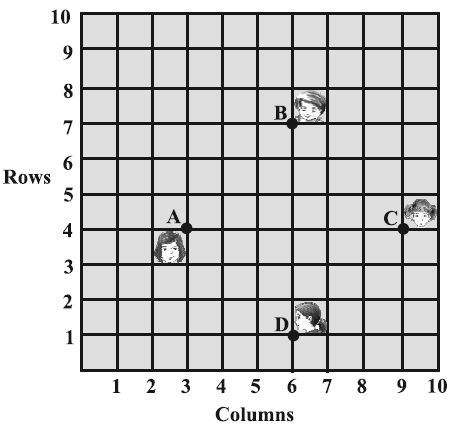
Solution :

It can be observed that A (3, 4) , B (6, 7), C(9, 4) and D(6, 1) are the position of these 4 friends.

Length of AB :

Here, x₁  =  3, y₁  =  4, x₂  =  6  and  y₂  =  7

  =  √(6 - 3)² + (7 - 4)²

  =  √(3)² + (3)²

  =  √9 + 9

  =  √18

  =  3√2

Length of BC :

Here, x₁  =  6, y₁  =  7, x₂  =  9  and  y₂  =  4

  =  √(9 - 6)² + (4 - 7)²

  =  √(3)² + (-3)²

  =  √(9 + 9)

  =  √18

  =  3√2

Length of CD :

Here, x₁  =  9, y₁  =  4, x₂  =  6  and  y₂  =  1

  =  √(6 - 9)² + (1 - 4)²

  =  √(-3)² + (-3)²

  =  √9 + 9

  =  √18

  =  3√2

Length of DA :

Here, x₁  =  6, y₁  =  1, x₂  =  3  and  y₂  =  4

  =  √(3 - 6)² + (4 - 1)²

  =  √(-3)² + (3)²

  =  √9 + 9

  =  √18

  =  3√2

Length of diagonal AC :

Here, x₁  =  3, y₁  =  4, x₂  =  9  and  y₂  =  4

  =  √(9 - 3)² + (4 - 4)²

  =  √6² + 0²

  =  √36

  =  6

Length of diagonal BD : 

Here, x₁  =  6, y₁  =  7, x₂  =  6  and  y₂  =  1

  =  √(6 - 6)² + (1 - 7)²

  =  √0² + (-6)²

  =  √36

  =  6

It can be observed that all the sides of this quadrilateral ABCD are the same length and also the diagonals are of the same length.

Therefore, ABCD is a square. Hence, Champa was correct.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.