FINDING AREA USING INTEGRATION

We can use definite integrals to find the area of a region bounded by a curve, x or y-axis and the lines. For example, consider the curve y = f(x) above the x-axis as shown below.

Then, the area bounded by the curve y = f(x), the x-axis and the ordinates x = a and x = b is given by

Let the curve y = f(x) be below the x-axis as shown below. 

Then, the area bounded by the curve y = f(x), the x-axis and the  ordinates x = a and x = b is given by

Example 1 :

Find the area of the region bounded by the line y = x - 3, x = 1, x = 5 and x-axis.

Solution :

The line y = x - 3 crosses x-axis at x = 3.

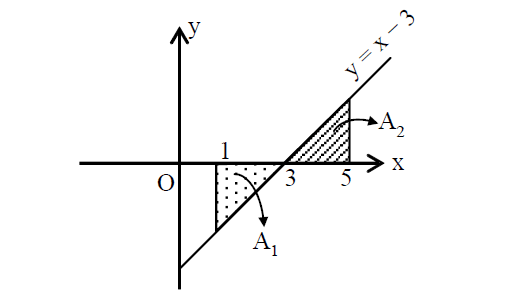
From the diagram, it is clear that A₁ lies below x-axis.

Then, we have

As A₂ lies above the x-axis,

Therefore, the total area is

Example 2 :

Find the area of the region bounded by y = x² - 5x + 4, x = 2, x = 3 and x-axis.

Solution :

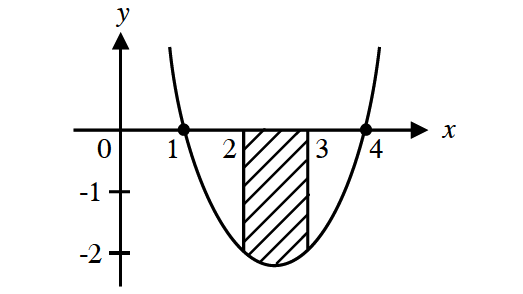
Required area is

Example 3 :

Find the area bounded by the curve y = sin(2x) between the ordinates x = 0, x = π and x-axis.

Solution :

The points where the curve y = sin(2x) meets the x-axis can be obtained by putting y = 0.

sin 2x = 0

2x = nπ, n ∈ Z

The values of x between x = 0 are x = π are x = 0, ^π⁄₂, π.

The limits for the first arch are 0 and ^π⁄₂ and the curve lies above x-axis.

The limits for the second arch are ^π⁄₂ and π and the curve lies below x-axis.

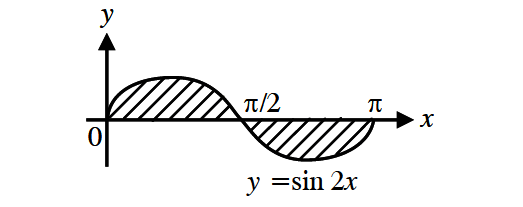
Required area is

Example 4 :

Find the area bounded by the curve y = x² - x - 2, x-axis, x = -2, and x = 4.

Solution :

Substuite y = 0 into y = x² - x - 2 to find at where the curve intersects x-axis.

x² - x - 2 = 0

x² + x - 2x - 2 = 0

x(x + 1) - 2(x + 1) = 0

(x + 1)(x - 2) = 0

x = -1  or  x = 2

This curve intersects x-axis at x = -1 and x = 2.

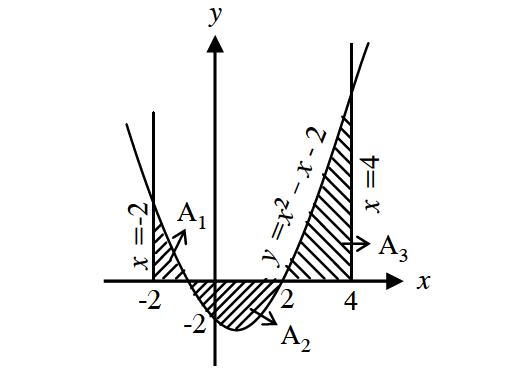
The part A₂ lies below x-axis. Then, we have

Therefore, the required area is 

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.