FIND THE RATIO IN WHICH THE LINE SEGMENT IS DIVIDED BY X OR Y AXIS

Example 1 :

Find the ratio in which x axis divides the line segment joining the points (6, 4) and (1,- 7).

Solution :

Let l : m be the ratio of the line segment joining the points (6, 4) and (1, -7) and let p(x, 0) be the point on the x axis.

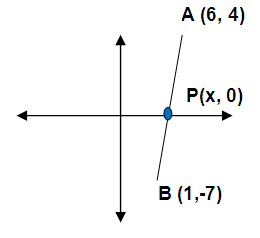
Section formula internally  =  

(lx₂+mx₁)/(l+m),  (ly₂+my₁)/(l+m)

(x, 0)  =  [l(1)+m(6)]/(l+m) , [l(-7)+m(4)]/(l+m)

(x , 0)  =  [l+6m]/(l+m) , [-7l+4m]/(l+m)

Equating y-coordinates 

[-7l+4m]/(l+m)  =  0

-7l+4m  =  0

-7l  =  -4m

l/m  =  4/7

l : m = 4 : 7

So, x-axis divides the line segment in the ratio 4:7.

Example 2 :

Find the ratio in which x axis divides the line segment joining the points (6 , 4) and (1 ,- 7).

Solution :

Let l:m be the ratio of the line segment joining the points (6, 4) and (1, -7) and let p(x, 0) be the point on the x axis.

Let l:m be the ratio of the line segment joining the points (-5, 1) and (2, 3) and let p(0, y) be the point on the y axis.

Section formula  internally  =

 (lx₂+mx₁)/(l+m),  (ly₂+my₁)/(l+m)

(0 , y)  =   [l(2)+m(-5)]/(l+m) , [l(3)+m(1)]/(l+m)

(0 , y)  =  [2l-5m]/(l+m), [3l+m]/(l+m)

(2l-5m)/(l+m)  =  0

2l-5 m  =  0

2l  =  5m

l/m  =  5/2

l:m  =  5 : 2

To find the required point we have to apply this ratio in the formula.

(0, y)  =   [2(5)–5(2)]/(5+2), [3(5)+2]/(5+2)

(0, y)  =  (10–10)/7, (15+2]/7

(0 , y)  =   (0, 17/7)

So,  the required point is (0, 17/7).

Example 3 :

In what ratio is the line segment joining A(2, -3) and B(5, 6) is divided by the x-axis. Also find the coordinates of the intersection of AB on the x-axis.

Solution :

Let l : m be the ratio of the line segment joining the points A(2, -3) and B(5, 6).

Let p(x, 0) be the point on the x axis.

Section formula internally  =  

(lx₂ + mx₁)/(l + m),  (ly₂ + my₁)/(l + m)

(x, 0)  =  [l(5) + m(2)]/(l + m) , [l(6) + m(-3)]/(l + m)

(x , 0)  =  [5l+2m]/(l+m) , [6l-3m]/(l+m)

Equating y-coordinates 

[6l-3m]/(l+m)  =  0

6l - 3m = 0

6l = 3m

l/m = 3/6

l : m = 1 : 2

So, x-axis divides the line segment in the ratio 1 : 2.

To find the required point we have to apply this ratio in the formula.

= [l(5) + m(2)]/(l + m) , [l(6) + m(-3)]/(l + m)

= (5l + 2m) / (l + m), (6l - 3m) / (l + m)

= (5(1) + 2(2)) / (1 + 2), (6(1) - 3(2)) / (1 + 2)

= (5 + 4)/3, (6 - 6) / 3

= 9/3, 0/3

= (3, 0)

So, the required point is (3, 0).

So, the required point is (0, 1).

Example 4 :

In what ratio is the line segment joining A(-2, 3) and (3, 7) is divided the y-axis. Also find the coordinates of the point of intersection of AB on the y-axis.

Solution :

Let l:m be the ratio of the line segment joining the points A(-2, -3) and (3, 7)  

let p(0, y) be the point on the y axis.

Section formula  internally  =

 (lx₂+mx₁)/(l+m),  (ly₂+my₁)/(l+m)

(0 , y)  =   [l(3)+m(-2)]/(l+m) , [l(7)+m(-3)]/(l+m)

(0 , y)  =  [3l - 2m]/(l+m), [7l - 3m]/(l+m)

Equating x-coordinates, we get

(3l - 2m) / (l + m) = 0

3l - 2m = 0

3l = 2m

l/m = 2/3

l : m = 2 : 3

So, the required ratio is 2 : 3.

To find the required point we have to apply this ratio in the formula.

[3l - 2m]/(l+m), [7l - 3m]/(l+m)

= [3(2) - 2(3)]/(2 + 3), [7(2) - 3(3)]/(2 + 3)

= [6 - 6]/5, [14 - 9]/5

= 0/5, 5/5

= (0, 1)

So, the required point is (0, 1).

Example 5 :

In what ratio is the line segment joining A(1, -5) and B(-4, 5) is divided by the x-axis. Also find the coordinates of the intersection of AB on the x-axis.

Solution :

Let l : m be the ratio of the line segment joining the points A(1, -5) and B(-4, 5)

Let p(x, 0) be the point on the x axis.

Section formula internally  =  

(lx₂ + mx₁)/(l + m),  (ly₂ + my₁)/(l + m)

(x, 0)  =  [l(-4) + m(1)]/(l + m) , [l(5) + m(-5)]/(l + m)

(x , 0)  =  [-4l + m]/(l + m) , [5l - 5m]/(l + m)

Equating y-coordinates 

[5l - 5m]/(l + m)  =  0

5l - 5m = 0

5l = 5m

l/m = 5/5

l : m = 1 : 1

So, x-axis divides the line segment in the ratio 1 : 1

To find the required point we have to apply this ratio in the formula.

= [-4l + m]/(l + m) , [5l - 5m]/(l + m)

= [-4(1) + 1]/(1 + 1) , [5(1) - 5(1)]/(1 + 1)

= (-4 + 1) / 2, (5 - 5) / 2

= -3/2, 0/2

= (-3/2, 0)

So, the required point is (-3/2, 0).

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.