FIND THE EQUATION OF THE PARABOLA WITH THE GIVEN INFORMATION

Question 1 :

Find the equation of the parabola in each of the cases given below:

(i) focus (4, 0) and directrix x = −4.

Solution :

From the given information, we know that the given parabola is symmetric about x-axis and it is open leftward.

Equation of the parabola with vertex (0, 0)

y²  =  4ax

Here a = 4

y²  =  4(4)x

y²  =  16x

(ii) passes through (2,-3) and symmetric about y -axis.

Solution :

Equation of the parabola which is symmetric about y-axis and open down ward

x²  =  -4ay  ----(1)

The parabola is passes through the point (2, -3)

2²  =  -4a(-3)

4  =  12a

a  =  4/12

a  =  1/3

By applying the value of a in (1), we get

 x²  =  -4(1/3)y

 3x²  =  -4y

(iii) vertex (1,-2) and focus (4,-2).

Solution :

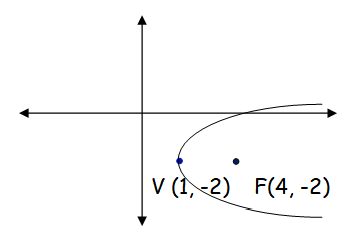
The parabola is symmetric about x axis and open right ward.

(y - k)²  =  4a(x   - h)

Here (h, k) ==>  (1, -2) and F (4, -2)

Distance between vertex and focus  =  a

a  =  √(1 - 4)² + (-2 + 2)²

a  =  3

(y + 2)²  =  4(3)(x   - 1)

(y + 2)²  =  12(x   - 1)

(iv) end points of latus rectum(4,-8) and (4,8) .

Solution :

Latus rectum must be perpendicular to the axis of parabola.

Distance between endpoints of latus rectum.

a  =  √(4 - 4)² + (-8 - 8)²

a  =  √16

a  =  4

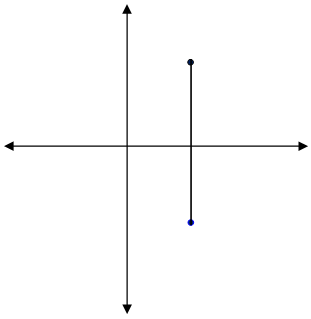
The parabola is symmetric about x-axis.

The parabola is passing through the points (4, -8) and (4, 8) 

(-8 - k)²  =  4(4)(4   - h)

(-8 - k)²  =  16(4 - h)  ----(1)

(8 - k)²  =  4(4)(4 - h)

(8 - k)²  =  16(4 - h) ----(2)

(1)  =  (2)

(8 + k)²  =  (8 - k)²

64 + 16k + k²  =  64 + k² - 16k

-32k  =  0

 k  =  0

By applying k = 0 in (1), we get 

(-8)²  =  16(4 - h)

64/16   =  4 - h

4 - h  =  4

-h  =  4 - 4

h  =  0

(y - 0)²  =  4(4)(x - 0)

y²  =  16x

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.