FACTORING METHOD SOLVING QUADRATIC EQUATIONS

The following steps will be useful to solve a quadratic equation by factoring.

Step 1 :

Write the equation in the form ax² + bx + c = 0.

Step 2 :

If the coefficient of x² is 1, we have to take the constant term and split it into two factors such that the product of those factors must be equal to the constant term and simplified value must be equal to the middle term.

If the coefficient of x² is not 1, we have to multiply the constant term along with the coefficient of x².Split the product into two factors.

Step 3 :

After factorizing, the given quadratic equation can be written as product of two linear factors.

Step 4 :

Equate each linear factor to zero and solve for x. These values of x gives the roots of the equation.  

How to assign signs for the two factors ?

Solved Questions

Question 1 :

Solve the following quadratic equations by factorization method :

√2x² + 7x + 5√2 = 0

Solution :

Product of coefficient of x²  and constant is 10.

√2x² + 2x + 5x + 5√2 = 0

2  =  √2 √2

√2x(x + √2) + 5(x + √2)  =  0

(√2x + 5) (x + √2)  =  0

√2x + 5  =  0     (or)   x + √2  =  0

Question 2 :

Solve the following quadratic equations by factorization method :

10x² - x - (1/5)  =  0

Solution :

(50x² - 5x - 1)/5  =  0

50x² - 5x - 1  =  0

50x² - 10x  + 5x - 1  =  0

10x(5x - 1) + 1(5x - 1)  =  0

(5x - 1)(10x + 1)  =  0

5x - 1 = 0  (or)  10x + 1 = 0

5x = 1  (or)  10x  =  -1

x = 1/5  (or)  x = -1/10

Question 3 :

The number of volleyball games that must be scheduled in a league with n teams is given by G (n) = (n² - n)/2 where each team plays with every other team exactly once. A league schedules 15 games. How many teams are in the league?

Solution :

Number of games scheduled  =  15

(n² - n)/2   =  15

n² - n   =  30

n² - n - 30  = 0

n² - 6n + 5n - 30  = 0

n(n -6) + 5(n - 6)  =  0

(n - 6)(n + 5)  =  0

n - 6  =  0  and  n + 5  =  0

 n  =  6    and  n = -5

Hence 6 teams are in the league.

Question 4 :

Victor is y years old. His brother Fred is four years old than Victor. The product of their ages is 780.

(a) Set up an equation to represent this information.

(b) Solve your equation from (a) to find Victor’s age.

Solution :

Age of Victor = y

Age of Fred = y + 4

The product of age = 780

y(y + 4) = 780

y² + 4y = 780

y² + 4y - 780 = 0

(y + 30)(y - 26) = 0

y = -30 and y = 26

Age of Victor = 26 years

Age of Fred = 26 + 4 ==> 30 years.

Question 5 :

A rectangular field is 30m longer than wide. The area of the field is 8800 m² Work out the perimeter of the field.

Solution :

Let x be the width of the rectangular field, width be x + 30

Area of rectangular field = length x width

x(x + 30) = 8800

x² + 30x = 8800

x² + 30x - 8800 = 0

(x + 110)(x - 80) = 0

x = -110 and x = 80

So, width of the rectangle is 80 m and width is 110 m.

Question 6 :

Shown is a right angled triangle. Show that

11x² − 62x − 105 = 0

and solve for x.

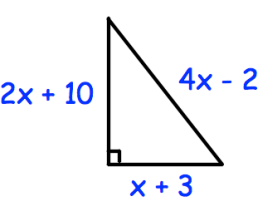
Solution :

Hypotenuse = 4x - 2, base = x + 3 and height = 2x + 10

Using Pythagorean theorem,

(4x - 2)² = (x + 3)² + (2x + 10)²

(4x)² - 2(4x) (2) + 2² = x² - 2x (3) + 3² + (2x)² - 2(2x) (10) + 10²

16x² - 16x + 4 = x² - 6x + 9 + 4x² - 40x + 100

16x² - 16x + 4 = 5x² - 46x + 109

16x² - 5x² - 16x - 46x + 4 - 109 = 0

9x² - 62x - 105 = 0

9x² + 27x - 35x - 105 = 0

9x(x + 3) - 35(x + 3) = 0

(9x - 35) (x + 3) = 0

So, the value of x is 35/9.

Question 7 :

Shown is a right angled triangle with sides are measured in centimeters.

(a) Show that x² − 5x − 14 = 0

(b) Solve for x.

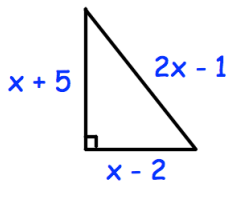
Solution :

Hypotenuse = 2x - 1, base = x - 2 and height = x + 5

Using Pythagorean theorem,

(2x - 1)² = (x - 2)² + (x + 5)²

(2x)² - 2(2x) (1) + 1² = x² - 2x (2) + 2² + x² + 2x (5) + 5²

4x² - 4x + 1 = x² - 4x + 4 + x² + 10x + 25

4x² - 4x + 1 = 2x² + 6x + 29

4x² - 2x² - 4x - 6x + 1 - 29 = 0

2x² - 10x - 28 = 0

Dividing by 2, we get

x² - 5x - 14 = 0

x² - 7x + 2x - 14 = 0

x(x - 7) + 2(x - 7) = 0

(x + 2)(x - 7) =0

x = -2 and x = 7

Question 8 :

The surface area of the cuboid is 270 cm².

(a) Show x² + 4x − 45 = 0

(b) Solve for x

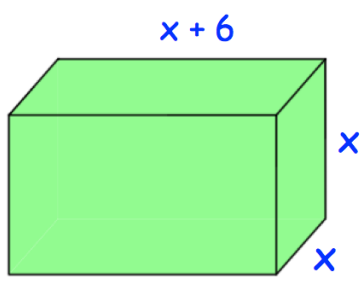
Solution :

Length = x + 6, width = x and height = x

Surface area of cuboid = 2(lw + wh + hl)

2(x² + 6x + x² + x² + 6x)

2(3x² + 12x) = 270

(3x² + 12x) = 135

3x² + 12x - 135 = 0

3x² + 27x - 15x - 135 = 0

3x(x + 9) - 15(x + 9) = 0

(3x - 15)(x + 9) = 0

x = 15/3 and x = -9

x = 5 and x = -9

So, the value of x is 5 cm.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.