DOMAIN AND RANGE OF LOGARITHMIC FUNCTIONS
In this section, you will learn how to find domain and range of logarithmic functions.
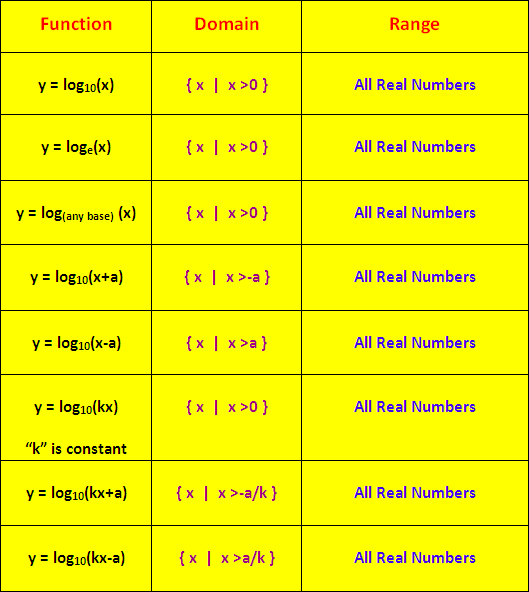
The table shown below gives the domain and range of different logarithmic functions.
Name of the Parts of a Logarithm
Usually a logarithm consists of three parts.
Let us come to the names of those three parts with an example.
log10A = B
In the above logarithmic function,
10 is called as Base
A is called as Argument
B is called as Answer
Fact to be known about domain of logarithm functions
A very important fact that we have to know about the domain of a logarithm to any base is,
"A logarithmic function is defined only for positive values of argument"
For example, if the logarithmic function is
y = log10x,
then the domain is
x > 0 or (0, +∞)
Domain of y = log₁₀ (x)
In the logarithmic function
y = log10(x),
the argument is 'x'.
From the fact explained above, argument must always be a positive value.
So, the values of x must be greater than zero.
Therefore, the domain of the above logarithmic function is
x > 0 or (0, +∞)
Domain of y = log₁₀ (x+a)
In the logarithmic function
y = log10(x+a),
the argument is 'x+a'.
From the fact explained above, argument must always be a positive value.
So, the values of 'x+a' must be greater than zero.
Then,
x + a > 0
Subtract 'a' from each side.
x > -a
Therefore, the domain of the above logarithmic function is
x > -a or (-a, +∞)
Domain of y = log₁₀ (x-a)
In the logarithmic function
y = log10(x-a),
the argument is 'x-a'.
From the fact explained above, argument must always be a positive value.
So, the values of 'x-a' must be greater than zero.
Then,
x - a > 0
Add 'a' to each side.
x > a
Therefore, the the domain of the above logarithmic function is
x > a or (a, +∞)
Domain of y = log₁₀ (kx)
In the logarithmic function
y = log10(kx),
the argument is 'kx'.
From the fact explained above, argument must always be a positive value.
So, the values of 'kx' must be greater than zero.
Then,
kx > 0
Divide each side by 'k'.
x > 0
Therefore, the the domain of the above logarithmic function is
x > 0 or (0, +∞)
Domain of y = log₁₀ (kx+a)
In the logarithmic function
y = log10(kx+a),
the argument is 'kx+a'.
From the fact explained above, argument must always be a positive value.
So, the values of 'kx+a' must be greater than zero.
Then,
kx + a > 0
Subtract 'a' from each side.
kx > -a
Divide each side by k.
x > -a/k
Therefore, the the domain of the above logarithmic function is
x > -a/k or (-a/k, +∞)
Domain of y = log₁₀ (kx-a)
In the logarithmic function
y = log10(kx-a),
the argument is 'kx-a'.
From the fact explained above, argument must always be a positive value.
So, the values of 'kx-a' must be greater than zero.
Then,
kx - a > 0
Add 'a' to each side.
kx > a
Divide each side by k.
x > a/k
Therefore, the the domain of the above logarithmic function is
x > a/k or (a/k, +∞)
Some more stuff on domain of logarithmic functions
Let us consider the logarithmic functions which are explained above.
y = log10(x)
y = log10(x+a)
y = log10(x-a)
y = log10(kx)
y = log10(kx+a)
y = log10(kx-a)
Domain is already explained for all the above logarithmic functions with the base '10'.
In case, the base is not '10' for the above logarithmic functions, domain will remain unchanged.
For example, in the logarithmic function
y = log10(x),
instead of base '10', if there is some other base, the domain will remain same. That is
x > 0 or (0, +∞)
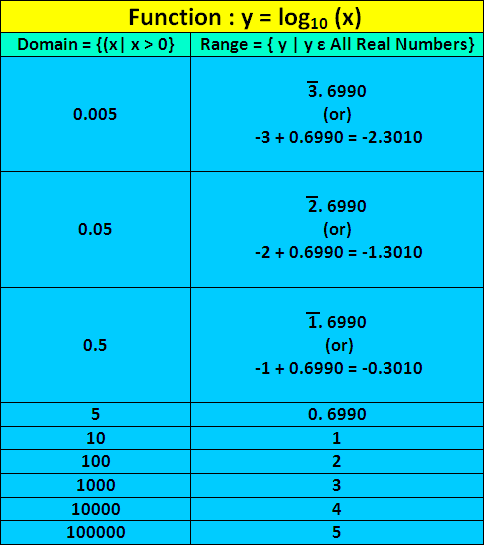
Range of Logarithmic Functions
The table shown below explains the range of y = log10(x).
That is,
"All Real Numbers"
Here, we may think that if the base is not 10, what could be the range of the logarithmic functions?
Whatever base we have for the logarithmic function, the range is always
"All Real Numbers"
For the base other than '10', we can define the range of a logarithmic function in the same way as explained above for base '10'.
Apart from the stuff given in this section, if you need any other stuff in math, please use our google custom search here.
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
WORD PROBLEMS
Word problems on simple equations
Word problems on linear equations
Word problems on quadratic equations
Area and perimeter word problems
Word problems on direct variation and inverse variation
Word problems on comparing rates
Converting customary units word problems
Converting metric units word problems
Word problems on simple interest
Word problems on compound interest
Word problems on types of angles
Complementary and supplementary angles word problems
Markup and markdown word problems
Word problems on mixed fractions
One step equation word problems
Linear inequalities word problems
Ratio and proportion word problems
Word problems on sets and Venn diagrams
Pythagorean theorem word problems
Percent of a number word problems
Word problems on constant speed
Word problems on average speed
Word problems on sum of the angles of a triangle is 180 degree
OTHER TOPICS
Time, speed and distance shortcuts
Ratio and proportion shortcuts
Domain and range of rational functions
Domain and range of rational functions with holes
Graphing rational functions with holes
Converting repeating decimals in to fractions
Decimal representation of rational numbers
Finding square root using long division
L.C.M method to solve time and work problems
Translating the word problems in to algebraic expressions
Remainder when 2 power 256 is divided by 17
Remainder when 17 power 23 is divided by 16
Sum of all three digit numbers divisible by 6
Sum of all three digit numbers divisible by 7
Sum of all three digit numbers divisible by 8
Sum of all three digit numbers formed using 1, 3, 4
Sum of all three four digit numbers formed with non zero digits
Sum of all three four digit numbers formed using 0, 1, 2, 3
Sum of all three four digit numbers formed using 1, 2, 5, 6
©All rights reserved. onlinemath4all.com
Recent Articles
-
Linear Growth and Decay
May 22, 22 03:05 AM
Linear Growth and Decay
-
Worksheet on Probability
May 22, 22 01:15 AM
Worksheet on Probability
-
Probability Worksheet
May 22, 22 01:12 AM
Probability Worksheet