DESCRIBING DECIMAL FORMS OF RATIONAL NUMBERS

A rational number is a number that can be written as a ratio of two integers a and b, where b is not zero. For example, 4/7 is a rational number, as is 0.37 because it can be written as the fraction 37/100.

Example 1 : 

Use a calculator to find the equivalent decimal form of each fraction in the table.

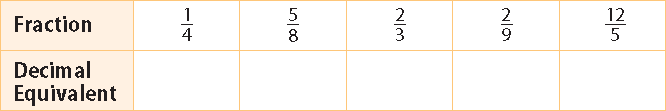
Solution : 

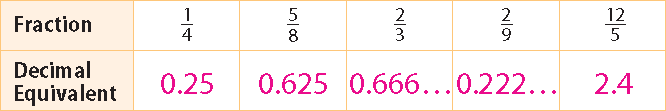
Example 2 : 

Now find the corresponding fraction of the decimal equivalents given below. Write the fractions in simplest form.

0.2, 0.875

Solution : 

0.2  =  2/10  =  1/5

0.875  =  875/1000  =  7/8

Example 3 : 

What do you notice about the digits after the decimal point in the decimal forms of the fractions? Compare notes with your neighbor and refine your conjecture if necessary.

Solution : 

The digits after the decimal point either repeat or terminate.

Example 4 : 

Consider the decimal 0.101001000100001000001…. Do you think this decimal represents a rational number? Why or why not?

Solution : 

Sample answer : No; since the digits after the decimal point do not terminate or repeat, it does not represent a rational number.

Example 5 : 

Do you think a negative sign affects whether or not a number is a rational number ? Use -8/5 as an example.

Solution : 

No; -8/5  =  -1.6, which is a rational number since the decimal terminates. Rational numbers can be negative.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.