DEFINE INTEGRALS

Example 1 :

Evaluate the following

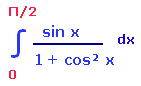
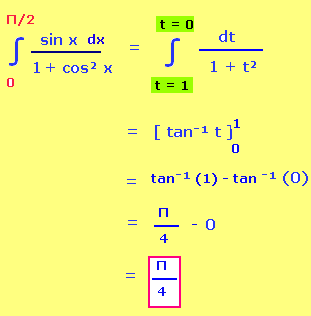
Solution :

Let t  =  cos x

Differentiating both sides with respect to x

dt  =  -sin x dx and sin x dx  =  -dt

We have changed the given function in terms of t from the variable x .So, we need to change the limits also.

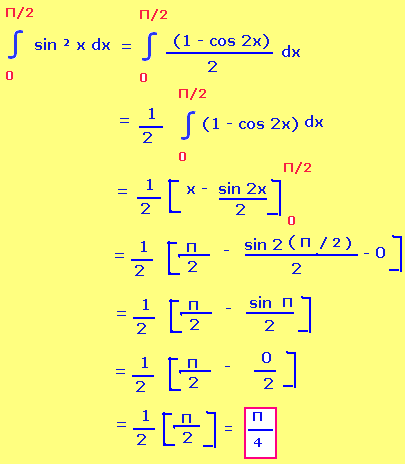
Example 2 :

Evaluate the following

Integral 0 to Π/2 sin² x

Solution :

To solve this problem we have to use the trigonometric formula for sin² x.

sin²x  =   (1 - cos 2x)/2

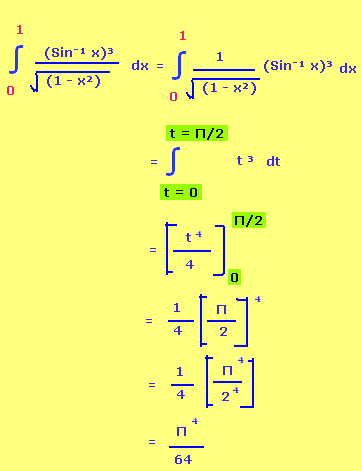
Example 3 :

Evaluate the following

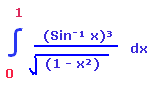
Solution :

t  =  Sin⁻¹x

Differentiating with respect to x on both sides

 dt  =  1/√(1-x²) dx

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.