COMBINED FIGURES

Example 1 :

Find the perimeter and area of the following figures:

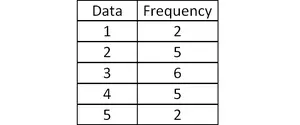
Solution :

In the above figure we have four semi circles and one square. To find the perimeter, we have to add the sum of four semicircles and a square.

Radius  =  7/2  =>  3.5 cm   

Length of each side of square = 7 cm

The perimeter of the given figure

=  4 (perimeter of semicircles) + Perimeter of square

Perimeter of semi circle AEB  =  Π r

=  (22/7) x 3.5

=  22 x 0.5

=  11

Perimeter of 4 semi circles  =  4 (11)

=  44 cm

Perimeter of square = 4a

=  28 cm

The perimeter of the given figure = 44 cm + 28 cm

= 72 cm

Area of 1 semi circle = Π r²/2

=  (1/2) x (22/7) x (7/2)²

=  (1/2) x (22/7) x (7/2) x (7/2)

=  (1/2) x 11 x (7/2)

=  77/4

=  19.25 cm²

Area of 4 semi circles  =  4 (19.25)

=  77

Area of square  =  a²

=  7x7

=  49 cm²

Area of given figure

= Area of 4 semi circles + Area of square

=  77 + 49 

=  126 cm²

Example 2 :

Find the area of shaded portion.

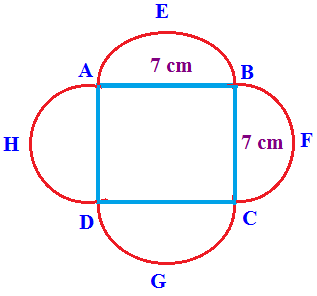
Solution :

Area of shaded portion

=  Area of rectangle - Area of 4 quadrants of circle

Area of rectangle  =  Length x Width

Length of rectangle  =  15 cm

Width of rectangle  =  8 cm

=  15 x 8

=  120 cm²

Area of quadrant  =  Π r²/4

radius of quadrant = 3 cm

=  [(22/7) x 3²]/4       

=  (22 x 3 x 3)/(7 x 4)   

=  198/28

Area of 4 quadrant = 4 x 198/28

=  198/7

=  28.28 cm²

Area of shaded portion  =  120 - 28.28

=  91.715 cm²

Example 3 :

The kitchen in Mario’s Italian restaurant is 18  meters long and 12 meters wide. A square  pantry is connected to the kitchen area. The  pantry is 3 meters wide. What is the total area  of the kitchen and pantry?

Solution :

Total area of the kitchen and pantry  =  Area of rectangle + Area of square

Area of rectangle  =  length x width

Area of square  =  a x a

Length of rectangle  =  18 m and width  =  12 m

Side length square pantry  =  3 m

Required area  =  (18 x 12) + (3 x 3)

=  216+9

=  225 square meter

Example 4 :

The area of  triangle QTU is 6 square units, and the  area of triangle RSU is 6 square units. The  dimensions in the figure below are labeled in  units. What is the area of  triangle STU in square  units?

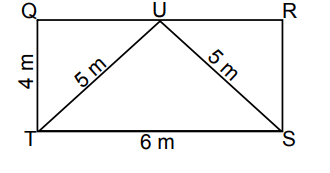
Solution :

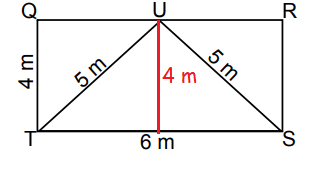
Area of triangle SUT 

=  Area of rectangle TQRS - 2(Area of TQU)

Area of rectangle  =  length x width

=  6 x 4

=  24 m²

TU²  =  TQ² + UQ²

5²  =  4² + UQ²

UQ²  =  25-16

UQ²  =  9

UQ  =  3

Area of triangle TQU  =  (1/2) x base x height

=  (1/2) x 3 x 4

=  6

Area of triangles TQU and SRU  =  12

Required area  =  24 - 12

=  12 m²

Example 5 :

The figure below is divided into four small  squares. The sides of each small square are 6  cm long. What is the area, in square  centimeters, of the entire figure?

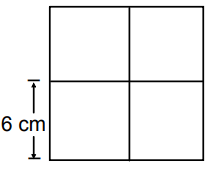
Solution :

Area of square  =  a x a

Side length  =  6 + 6  ==>  12 cm

Area of square  =  12 x 12

=  144 cm²

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.