AREA OF RHOMBUS
The area of a rhombus is equal to one half the product of the lengths of the diagonals.
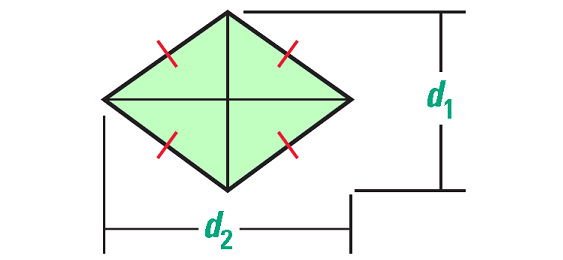
Let d1 and d2 be the lengths of diagonals of a rhombus.
Problem 1 :
If the lengths of the diagonals of a rhombus are 16 cm and 30 cm, find its area.
Solution :
Formula for area of a rhombus :
= 1/2 ⋅ (d1d2)
Substitute 16 for d1 and 30 for d2.
= 1/2 ⋅ (16 ⋅ 30)
= 8 ⋅ 30
= 240 cm2
So, area of the rhombus is 240 square cm.
Problem 2 :
Find the area of the rhombus shown below.
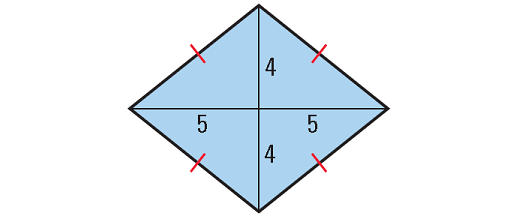
Solution :
In the rhombus shown above,
d1 = 5 + 5 = 10 units
d2 = 4 + 4 = 8 units
Formula for area of a rhombus :
= 1/2 ⋅ (d1d2)
Substitute 10 for d1 and 8 for d2.
= 1/2 ⋅ (10 ⋅ 8)
= 5 ⋅ 8
= 40
So, area of the rhombus is 40 square units.
Problem 3 :
Area of a rhombus is 192 square cm. If the length of one of the diagonals is 16 cm, find the length of the other diagonal.
Solution :
Area of the rhombus = 192 cm2
1/2 ⋅ (d1d2) = 192
Substitute 16 for d1.
1/2 ⋅ (16 ⋅ d2) = 192
8 ⋅ d2 = 192
Divide each side by 8.
d2 = 24 cm
So, the length of the other diagonal is 24 cm.
Problem 4 :
Area of a rhombus is 120 square units. If the lengths of the diagonals are 10 units and (7x + 3) units, then find the value of x.
Solution :
Area of the rhombus = 120 cm2
1/2 ⋅ (d1d2) = 120
Substitute 10 for d1 and (7x + 3) for d2.
1/2 ⋅ [10(7x + 3)] = 120
5(7x + 3) = 120
Divide each side by 5.
7x + 3 = 24
Subtract 3 from each side.
7x = 21
Divide each side by 7.
x = 3
Problem 5 :
Area of the rhombus shown below is 48 square inches. What is the value of x ?

Solution :
In the rhombus shown above,
d1 = 8 + 8 = 16 units
d2 = x + x = 2x units
Given : Area of the rhombus is 48 square inches.
Then,
1/2 ⋅ (d1d2) = 48
Substitute 16 for d1 and 2x for d2.
1/2 ⋅ (16 ⋅ 2x) = 48
8 ⋅ 2x = 48
16x = 48
Divide each side by 16.
x = 3
Problem 6 :
Find the area of the rhombus shown below.
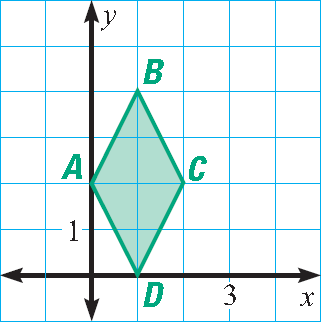
Solution :
Measure the lengths of the diagonals AC and BD.
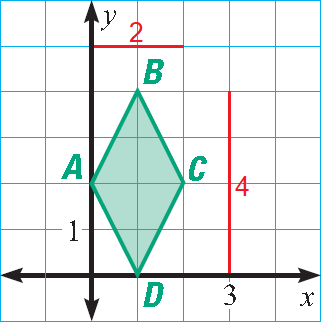
The lengths of the diagonals are 4 units and 2 units.
Formula for area of a rhombus :
= 1/2 ⋅ (d1d2)
Substitute 4 for d1 and 2 for d2.
= 1/2 ⋅ (4 ⋅ 2)
= 2 ⋅ 2
= 4
So, area of the rhombus is 4 square units.
Problem 7 :
Find the area of the rhombus having each side equal to 17 cm and one of its diagonals equal to 16 cm.
Solution :
Let A, B, C and D be the vertices of the rhombus.
The diagonals of a rhombus will be perpendicular and they will bisect each other.
Then, we have
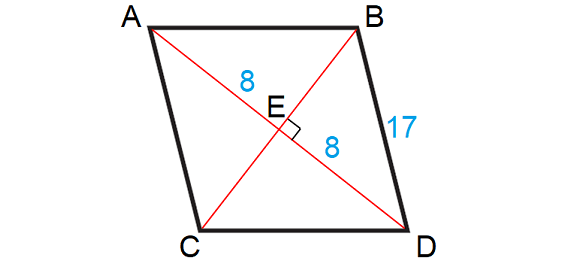
In the above rhombus, consider the right angled triangle BDE.
By Pythagorean Theorem,
BD2 = BE2 + DE2
172 = BE2 + 82
289 = BE2 + 64
Subtract 64 from each side.
225 = BE2
152 = BE2
15 = BE
Then,
EC = 15
Length of the diagonal BC :
BC = BE + EC
BC = 15 + 15
BC = 30 units
So, the lengths of the diagonals are 16 units and 30 units.
Formula for area of a rhombus :
= 1/2 ⋅ (d1d2)
Substitute 16 for d1 and 30 for d2.
= 1/2 ⋅ (16 ⋅ 30)
= 8 ⋅ 30
= 240
So, area of the rhombus is 240 square units.
Problem 8 :
One of the diagonals of a rhombus is double and other diagonal. Its area is 25 sq.cm. The sum of the diagonal is :
a) 10 cm b) 12 cm c) 15 cm d) 16 cm
Solution :
Let d1 and d2 be the length of diagonals.
d1 = 2d2
Area of rhombus = 1/2 ⋅ (d1d2)
25 = (1/2) ⋅ (2d2d2)
25 = d22
d2 = √25
d2 = 5 cm
d1 = 2(5) ==> 10 cm
Sum of the diagonal = d1 + d2
= 10 + 5
= 15 cm
So, the sum of the diagonals is 15 cm.
Problem 9 :
The perimeter of the rhombus is 56 m, and its height is 5 m. Its area is
a) 64 sq.m b) 70 sq.m c) 78 sq.m d) 84 sq.m
Solution :
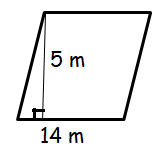
Perimeter of rhombus = 56 m
4a = 56
a = 56/4
a = 14 m
Area of rhombus = base x height
= 14 x 5
= 70 sq.m
So, area of the rhombus is 70 sq.m
Problem 10 :
The length of one diagonal of a rhombus is 80% of the other diagonal. The area of the rhombus is how many times the square of the length of other diagonal ?
a) 4/5 b) 2/5 c) 3/4 d) 1/4
Solution :
Let d1 and d2 be the length of diagonals.
d1 = 0.80d2
Area of rhombus = 1/2 ⋅ (d1d2)
= 1/2 ⋅ (0.80d2d2)
= 0.40 d22
= (40/100) d22
= (2/5) d22
So, option b is correct.
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Time and Work Problems
Oct 20, 25 07:13 AM
Time and Work Problems - Concept - Solved Problems -
10 Hard SAT Math Questions (Part - 30)
Oct 17, 25 07:27 PM
10 Hard SAT Math Questions (Part - 30) -
10 Hard SAT Math Questions (Part - 29)
Oct 16, 25 06:04 AM
10 Hard SAT Math Questions (Part - 29)
