EXPONENTS AND SCIENTIFIC NOTATION

Exponents

The exponent of a number says, how many times the number has to be multiplied by itself. 

For example,

5³  =  5 x 5 x 5

In words, 5³ could be called as 5 to the power 3 or 5 cube.

The other names of exponents are indices and powers.

Laws of Exponents

Law 1 : 

x^m ⋅ xⁿ  =  x^m+n

Example :

3⁴ ⋅ 3⁵  =  3⁴⁺⁵

3⁴ ⋅ 3⁵  =  3⁹

Law 2 : 

x^m ÷ xⁿ  =  x^m-n

Example :

3⁷ ÷ 3⁵  =  3^7-5

3⁷ ÷ 3⁵  =  3²

Law 3 : 

(x^m)ⁿ  =  x^mn

Example :

(3²)⁴  =  3⁽²⁾⁽⁴⁾

(3²)⁴  =  3⁸

Law 4 : 

(xy)^m  =  x^m ⋅ y^m

Example :

(3 ⋅ 5)²  =  3² ⋅ 5²

(3 ⋅ 5)²  =  9 ⋅ 25

(3 ⋅ 5)²  =  225.

Law 5 : 

(x / y)^m  =  x^m / y^m

Example :

(3 / 5)²  =  3² / 5²

(3 / 5)²  =  9 / 25

Law 6 : 

x^-m  =  1 / x^m

Example :

3^-2  =  1 / 3²

3^-2  =  1 / 9

Law 7 : 

x⁰  =  1

Example :

3⁰  =  1

Law 8 : 

x¹  =  x

Example :

3¹  =  3

Law 9 : 

x^m/n  =  y -----> x  =  y^n/m

Example :

x^1/2  =  3

x  =  3^2/1

x  =  3²

x  =  9

Law 10 : 

(x / y)^-m  =  (y / x)^m

Example :

(5 / 3)^-2  =  (3 / 5)²

(5 / 3)^-2  =  3² /  5²

(5 / 3)^-2  =  9 / 25

Law 11 : 

a^x  =  a^y -----> x  =  y

Example :

3^m  =  3⁵ -----> m  =  3

Law 12 : 

x^a  =  y^a -----> x  =  y

Example :

k³  =  5³ -----> k  =  5

Scientific Notation Rules

Every number in the scientific notation must be in the form of 

a x 10ⁿ

where 1 ≤ a < 10 and n must be a positive or negative integer. 

To convert a number into scientific notation, first we have to identify where the decimal point and non zero digit come. 

There are two cases in it. 

Case 1 : 

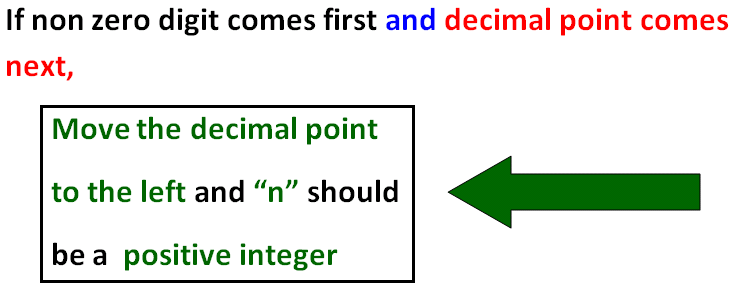
To move the decimal point to the left, we have to count number of digits as explained in the example given below. 

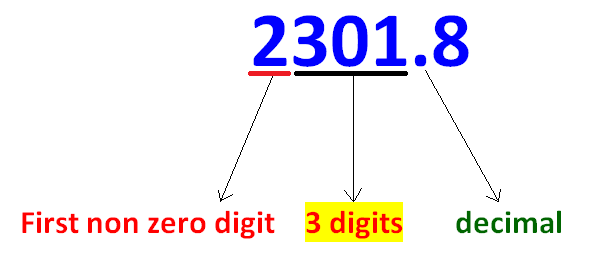
According to the example given above, we have to move the decimal point 3 digits to the left and exponent of 10 should be 3 (positive integer)

When we do so, we get the scientific notation of the given number. 

Hence, 2301.8 = 2.3018 x 10³

Case 2 : 

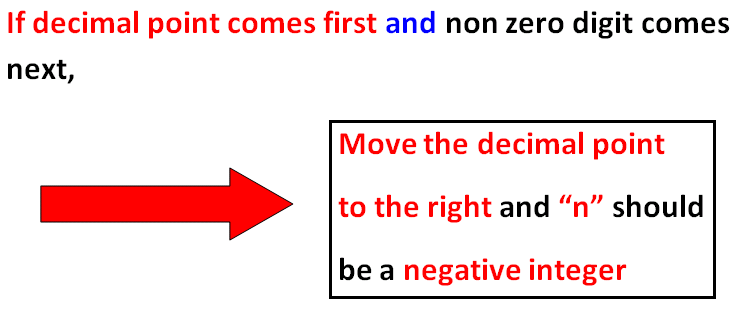
To move the decimal point to the right, we have to count number of digits as explained in the example given below. 

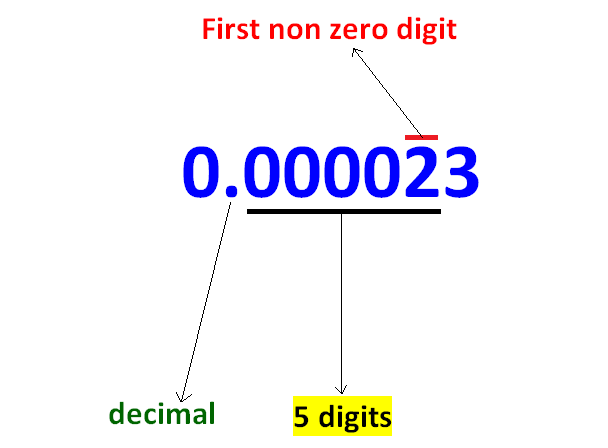
According to the example given above, we have to move the decimal point 5 digits to the right and exponent of 10 should be -5 (negative integer)

When we do so, we get the scientific notation of the given number. 

Hence, 0.000023 = 2.3 x 10⁻⁵

Important Note: 

If we don't find decimal point at anywhere of the given number, we have to assume that there is decimal point at the end of the number.

For example, 

2300000 -------------> 2300000.

Here, the non zero digit comes first and decimal point comes next. So we have to apply case 1 to convert this number into scientific notation.  

If you would like to have practice problems on exponents,

Please click here

If you would like to have practice problems on scientific notation,

Please click here

Apart from the stuff given above, if you need any other stuff, please use our google custom search here. 

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.